What Is 24/30 As A Percent
Kalali
Apr 23, 2025 · 5 min read
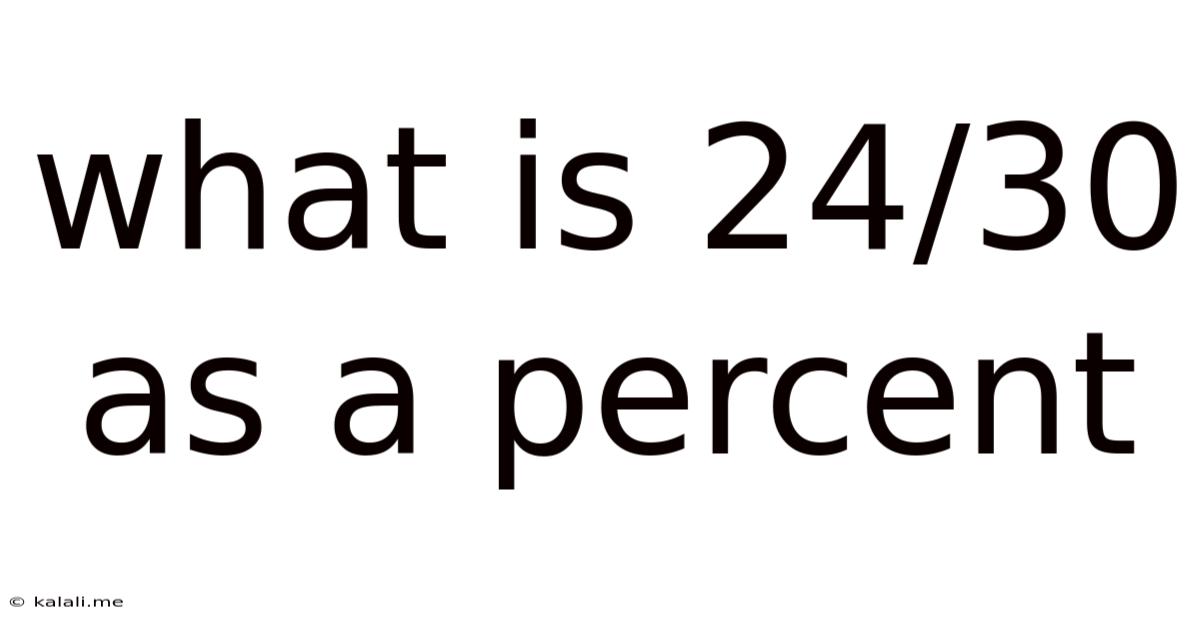
Table of Contents
What is 24/30 as a Percent? A Comprehensive Guide to Fraction-to-Percentage Conversion
This article will delve into the conversion of the fraction 24/30 into a percentage, exploring the underlying mathematical principles and providing practical examples. We'll also discuss different methods for performing this conversion, empowering you to tackle similar fraction-to-percentage problems with confidence. Understanding this process is crucial for various applications, from everyday calculations to more advanced statistical analyses. This guide is designed for all levels, from those needing a quick refresher to those seeking a deeper understanding of the mathematical concepts involved.
Meta Description: Learn how to convert the fraction 24/30 into a percentage. This comprehensive guide explains the process step-by-step, covering different methods and providing practical examples for various applications.
Understanding Fractions and Percentages
Before diving into the conversion, let's briefly review the concepts of fractions and percentages. A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). The denominator indicates the total number of equal parts, and the numerator indicates how many of those parts are being considered.
A percentage, on the other hand, represents a proportion out of 100. It's denoted by the symbol "%". Percentages are widely used to express proportions, rates, and changes in various contexts, making them a convenient way to compare and analyze data.
Method 1: Converting the Fraction to a Decimal, then to a Percentage
This is arguably the most straightforward method. We first convert the fraction 24/30 into a decimal, and then convert that decimal into a percentage.
-
Simplify the Fraction: Before converting to a decimal, simplifying the fraction makes the calculation easier. Both 24 and 30 are divisible by 6:
24/30 = (24 ÷ 6) / (30 ÷ 6) = 4/5
-
Convert the Fraction to a Decimal: To convert the simplified fraction 4/5 to a decimal, we divide the numerator (4) by the denominator (5):
4 ÷ 5 = 0.8
-
Convert the Decimal to a Percentage: To convert a decimal to a percentage, we multiply the decimal by 100 and add the "%" symbol:
0.8 * 100 = 80%
Therefore, 24/30 is equal to 80%.
Method 2: Using Proportions
This method utilizes the concept of proportions to directly convert the fraction to a percentage. We set up a proportion where the fraction 24/30 is equal to x/100, where 'x' represents the percentage we're trying to find.
-
Set up the Proportion:
24/30 = x/100
-
Cross-Multiply: Cross-multiplying gives us:
30x = 24 * 100
30x = 2400
-
Solve for x: Divide both sides by 30:
x = 2400 ÷ 30
x = 80
Therefore, x = 80, meaning 24/30 is equal to 80%.
Method 3: Using the Percentage Formula
The percentage formula provides a direct route to converting a fraction to a percentage. The formula is:
Percentage = (Numerator / Denominator) * 100%
Applying this formula to the fraction 24/30:
Percentage = (24/30) * 100%
First, simplify the fraction: 24/30 = 4/5
Percentage = (4/5) * 100% = 0.8 * 100% = 80%
Understanding the Significance of 80%
The result, 80%, signifies that 24 represents 80% of the total value of 30. This percentage can be interpreted in various contexts. For instance, if a student answered 24 out of 30 questions correctly on a test, their score would be 80%. Similarly, if a store offers an 80% discount on an item originally priced at 30 units of currency, the discount amounts to 24 units of currency.
Practical Applications of Fraction-to-Percentage Conversion
Converting fractions to percentages is a fundamental skill with numerous applications across various fields:
- Academic Performance: Calculating grades and scores based on correct answers.
- Business and Finance: Analyzing profit margins, sales growth, and market shares.
- Data Analysis: Representing data proportions in charts and graphs for easy visualization and understanding.
- Everyday Life: Calculating discounts, tips, and tax rates.
- Science and Engineering: Expressing experimental results and probabilities.
Advanced Concepts and Further Exploration
While this article focuses on the basic conversion of 24/30 to a percentage, the underlying principles can be extended to more complex scenarios.
-
Converting Fractions with Decimals: The same methods can be applied to fractions with decimal numerators or denominators. The key is to maintain precision during the calculations.
-
Percentage Change: Understanding percentage change involves calculating the percentage increase or decrease between two values. This involves finding the difference between the two values and then expressing that difference as a percentage of the original value.
-
Compound Interest: Compound interest calculations involve repeated percentage increases, leading to exponential growth over time.
-
Statistical Analysis: Percentages are widely used in statistical analysis to represent probabilities, proportions, and other key metrics.
Conclusion: Mastering Fraction-to-Percentage Conversions
Mastering the conversion of fractions to percentages is a valuable skill that enhances problem-solving capabilities across various domains. The methods outlined in this article—converting to a decimal, using proportions, and applying the percentage formula—provide versatile approaches to tackling such conversions. By understanding the underlying mathematical principles and practicing these methods, you'll develop confidence in handling similar calculations efficiently and accurately. Remember to always simplify the fraction whenever possible to streamline the process. The ability to effortlessly convert fractions to percentages is a fundamental step towards a deeper understanding of mathematical concepts and their practical applications in everyday life and various professional fields. Practice regularly, and you'll find this skill becomes second nature!
Latest Posts
Latest Posts
-
Cuantos Grados Celsius Son 50 Fahrenheit
Apr 23, 2025
-
Is The Pacific Ocean Warmer Than The Atlantic
Apr 23, 2025
-
What Is 2 To The 10th Power
Apr 23, 2025
-
How Many Ounces Are 200 Grams
Apr 23, 2025
-
What Is 67 Inches In Cm
Apr 23, 2025
Related Post
Thank you for visiting our website which covers about What Is 24/30 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.