What Is 3 Out Of 20 As A Percentage
Kalali
Apr 18, 2025 · 5 min read
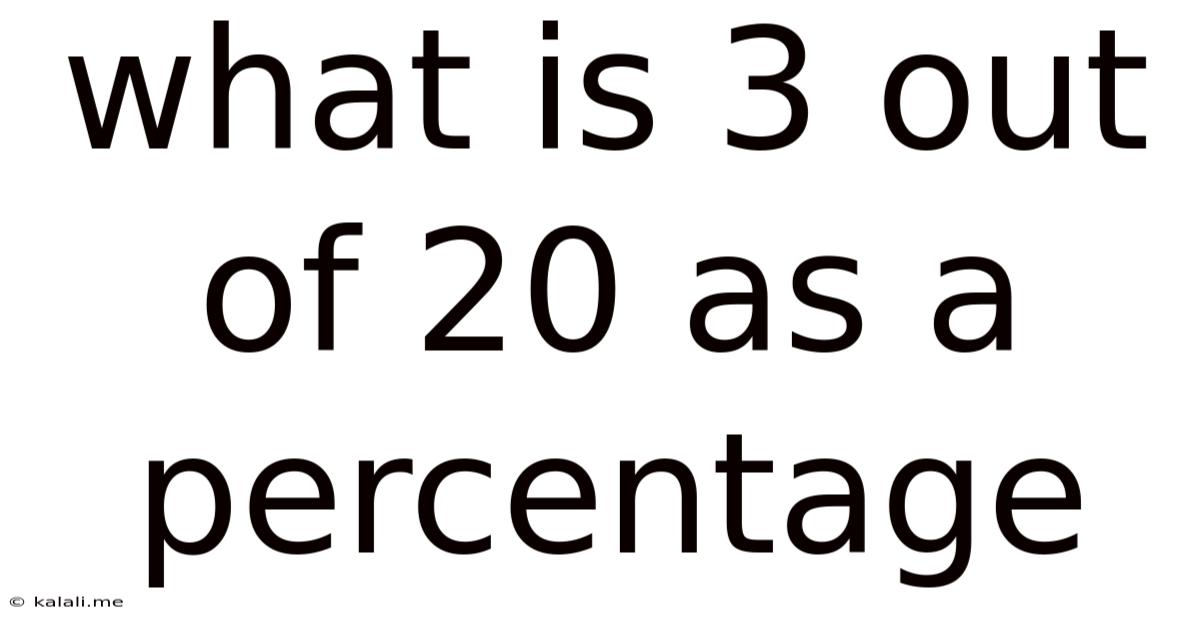
Table of Contents
What is 3 out of 20 as a Percentage? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many areas of life, from calculating discounts and taxes to understanding statistics and financial reports. This article will comprehensively guide you through calculating "3 out of 20 as a percentage," explaining the process step-by-step and exploring various methods and applications. We’ll also delve into the broader context of percentage calculations and their practical uses. By the end, you’ll not only know the answer but also grasp the underlying principles, enabling you to tackle similar percentage problems with confidence.
Meta Description: Learn how to calculate 3 out of 20 as a percentage. This comprehensive guide provides step-by-step instructions, explores different calculation methods, and offers practical applications of percentage calculations in everyday life.
Understanding Fractions and Percentages
Before we dive into the specific calculation, let's refresh our understanding of fractions and percentages. A fraction represents a part of a whole. In our case, "3 out of 20" is a fraction, written as 3/20. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100."
Calculating 3 out of 20 as a Percentage: Method 1 - Direct Conversion
The most straightforward method involves converting the fraction 3/20 into a percentage. Here's how:
-
Convert the fraction to a decimal: Divide the numerator (3) by the denominator (20): 3 ÷ 20 = 0.15
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.15 x 100 = 15
Therefore, 3 out of 20 is 15%.
Calculating 3 out of 20 as a Percentage: Method 2 - Proportion Method
This method uses the concept of proportions to find the equivalent percentage. We set up a proportion where x represents the percentage we want to find:
3/20 = x/100
To solve for x, we cross-multiply:
20x = 300
Then, divide both sides by 20:
x = 300 ÷ 20 = 15
Again, we find that 3 out of 20 is 15%.
Calculating 3 out of 20 as a Percentage: Method 3 - Using a Calculator
Most calculators have a percentage function that simplifies the process. Simply enter 3 ÷ 20 and then multiply the result by 100. The calculator will directly display the answer as 15%.
Practical Applications of Percentage Calculations
Understanding percentages is crucial in numerous real-world scenarios. Here are a few examples:
- Discounts: If a store offers a 15% discount on an item, you can easily calculate the savings.
- Taxes: Sales tax is usually expressed as a percentage of the purchase price.
- Grades and Scores: Academic performance is often represented as percentages. For example, a score of 15 out of 20 on a test translates to a 75% score (15/20 * 100 = 75%).
- Financial Analysis: Percentages are essential in interpreting financial statements, such as profit margins, return on investment (ROI), and interest rates.
- Statistics and Data Analysis: Percentages are used to represent proportions in data sets, making it easier to visualize and compare different groups or categories. For instance, if 3 out of 20 people surveyed preferred a particular product, it means 15% of the respondents favoured that product.
- Probability: Probabilities are often expressed as percentages. For example, a 15% chance of rain implies a 15 out of 100 probability of rainfall.
- Growth Rates: Percentages are used to calculate growth rates in various contexts, such as population growth, economic growth, and investment growth. For example, if a company's sales increase from 20 to 23 units, the growth rate is 15% ((3/20) * 100).
Beyond the Basics: Working with Larger Numbers and More Complex Percentages
While the example of 3 out of 20 is relatively simple, the same principles apply to more complex percentage calculations. Let's consider some variations:
-
Larger Numbers: Imagine you have 300 out of 2000 items. Using the same method (300/2000 * 100), you'll find this is still 15%. The underlying ratio remains the same.
-
Finding the Whole: Let's say you know that 15% of a certain quantity is 3. To find the total quantity, you can set up a proportion:
15/100 = 3/x
Solving for x, we get x = 20.
- Percentage Increase/Decrease: If a value increases from 20 to 23, the percentage increase is calculated as: [(23-20)/20] * 100 = 15%. Similarly, a decrease would be calculated using the same formula, resulting in a negative percentage.
Common Mistakes to Avoid When Calculating Percentages
Several common mistakes can lead to incorrect percentage calculations. Here are some to watch out for:
-
Incorrect order of operations: Always remember the order of operations (PEMDAS/BODMAS). Division should be performed before multiplication when converting fractions to percentages.
-
Misunderstanding the context: Clearly identify what the whole represents before calculating the percentage. For example, if you’re calculating the percentage of students who passed an exam, the total number of students is the whole, not just the number of students who passed.
-
Rounding errors: Be mindful of rounding errors, especially when dealing with several decimal places. Round only at the final stage of your calculation.
Advanced Percentage Calculations and Applications
For more advanced applications, you might need to work with:
-
Compound interest: This involves calculating interest on both the principal amount and accumulated interest.
-
Percentage change over time: Analyzing percentage changes across multiple periods requires understanding of compounding and potentially exponential growth or decay.
Conclusion
Calculating 3 out of 20 as a percentage is a fundamental exercise that illustrates the core principles of percentage calculations. Understanding these principles is essential for numerous applications in various fields. By mastering these techniques and avoiding common pitfalls, you can confidently tackle percentage problems of all levels of complexity. Remember to always carefully consider the context of the problem and choose the most appropriate method for your calculation. This comprehensive guide has equipped you with the knowledge and tools to navigate the world of percentages with ease and precision.
Latest Posts
Latest Posts
-
25 Is What Percent Of 65
Apr 19, 2025
-
How Many Ounces In 2 1 2 Quarts
Apr 19, 2025
-
17 Is What Percent Of 68
Apr 19, 2025
-
How Big Is 36 Inches In Feet
Apr 19, 2025
-
40 Celsius Is What In Fahrenheit
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 Out Of 20 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.