What Is 5 Out Of 6 As A Percentage
Kalali
Mar 11, 2025 · 5 min read
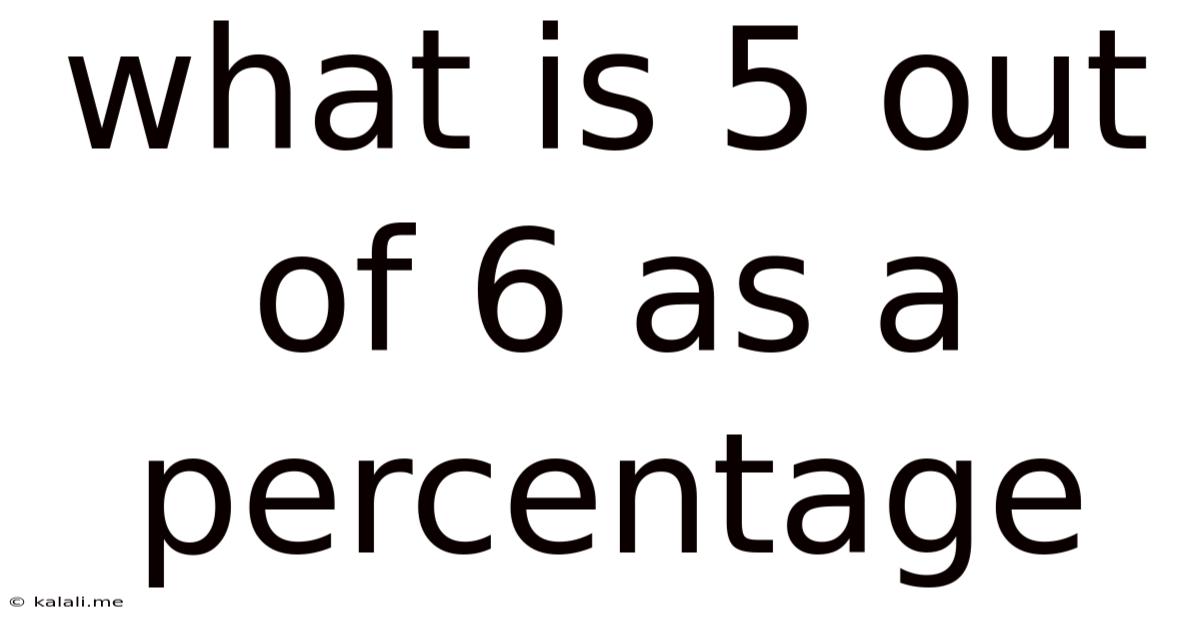
Table of Contents
What is 5 out of 6 as a Percentage? A Comprehensive Guide
Determining percentages is a fundamental skill with applications spanning various fields, from everyday calculations to complex statistical analysis. Understanding how to convert fractions to percentages is crucial for anyone seeking to improve their numeracy skills. This comprehensive guide will delve into the process of calculating "5 out of 6 as a percentage," exploring different methods and providing a deeper understanding of percentage calculations. We'll also touch upon real-world applications and provide you with the tools to tackle similar percentage problems with confidence.
Understanding Percentages
Before diving into the calculation, let's establish a clear understanding of percentages. A percentage is simply a fraction or ratio expressed as a number out of 100. The term "percent" is derived from the Latin "per centum," meaning "out of a hundred." Therefore, a percentage represents a portion of a whole, where the whole is always considered 100%.
For instance, 50% represents 50 out of 100, which simplifies to 1/2 or one-half. Similarly, 25% is 25 out of 100, simplifying to 1/4 or one-quarter. This concept forms the basis for converting fractions to percentages.
Calculating 5 out of 6 as a Percentage: Method 1 - The Fraction Method
The most straightforward method involves converting the fraction "5 out of 6" into a decimal and then multiplying by 100 to obtain the percentage.
Step 1: Represent the fraction:
The statement "5 out of 6" can be mathematically represented as the fraction 5/6.
Step 2: Convert the fraction to a decimal:
To convert the fraction to a decimal, divide the numerator (5) by the denominator (6):
5 ÷ 6 = 0.8333... (The 3s repeat infinitely)
Step 3: Convert the decimal to a percentage:
Multiply the decimal by 100:
0.8333... × 100 = 83.33...%
Therefore, 5 out of 6 is approximately 83.33%. The "..." indicates that the decimal is recurring; for practical purposes, you might round it to two decimal places (83.33%).
Calculating 5 out of 6 as a Percentage: Method 2 - The Proportion Method
This method uses the concept of proportions to solve the problem. We set up a proportion where we equate the fraction 5/6 to an equivalent fraction with a denominator of 100 (since percentages are out of 100).
Step 1: Set up the proportion:
5/6 = x/100
Where 'x' represents the percentage we need to find.
Step 2: Solve for x:
To solve for x, cross-multiply:
6x = 500
Step 3: Isolate x:
Divide both sides by 6:
x = 500/6 = 83.333...
Step 4: Convert to percentage:
x ≈ 83.33%
This confirms that 5 out of 6 is approximately 83.33%.
Understanding Recurring Decimals and Rounding
Notice that in both methods, we encounter a recurring decimal (0.8333...). Recurring decimals arise when a fraction cannot be expressed exactly as a terminating decimal. In practical applications, it's necessary to round the decimal to a suitable number of decimal places. The level of precision depends on the context. For most purposes, rounding to two decimal places (83.33%) is sufficient.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is invaluable in numerous everyday situations and professional fields. Here are some examples:
- Calculating grades: If you got 5 out of 6 questions correct on a test, your score is 83.33%.
- Discounts and sales: A store offering a 20% discount on an item initially priced at $60 will reduce the price by $12 ($60 x 0.20 = $12), resulting in a final price of $48.
- Tax calculations: Calculating sales tax or income tax involves determining a percentage of a given amount.
- Financial analysis: Percentages are crucial for interpreting financial statements, analyzing investment returns, and calculating interest rates.
- Statistical analysis: Percentages are used extensively in statistical analysis to represent proportions, frequencies, and probabilities.
- Data representation: Charts and graphs often use percentages to visualize data proportions effectively.
- Surveys and polls: Results from surveys and polls are often expressed as percentages to show the proportion of respondents who chose a particular option.
Further Percentage Calculations: Practice Problems
Let's solidify your understanding with a few more practice problems:
- What is 3 out of 5 as a percentage?
- What is 7 out of 10 as a percentage?
- What is 2 out of 3 as a percentage?
- A student answered 12 out of 15 questions correctly. What is their percentage score?
- A company's profits increased by 15% from $50,000 last year. What is their profit this year?
Solutions:
- 60%
- 70%
- 66.67%
- 80%
- $57,500
Advanced Percentage Calculations: Beyond the Basics
While the examples above cover common percentage calculations, the concept extends to more complex scenarios, such as:
- Percentage increase/decrease: Calculating the percentage change between two values. This involves finding the difference between the two values, dividing the difference by the original value, and multiplying by 100.
- Percentage points: A percentage point refers to an absolute difference between two percentages, not a relative change. For example, an increase from 10% to 15% is a 5-percentage-point increase, not a 50% increase.
- Compound interest: Calculating interest on principal plus accumulated interest. This is a more complex calculation involving exponential growth.
- Working backwards from percentages: If you know the final value after a percentage increase or decrease, you can calculate the original value.
Conclusion: Mastering Percentage Calculations
Calculating percentages, such as determining "5 out of 6 as a percentage," is a fundamental mathematical skill with widespread applications in daily life and various professions. By understanding the methods outlined in this guide – the fraction method and the proportion method – you'll be able to confidently tackle various percentage problems and interpret data effectively. Remember to practice regularly to build proficiency and explore the more advanced percentage calculations to broaden your understanding of this important mathematical concept. Mastering percentages will significantly enhance your numerical skills and empower you to make informed decisions in a range of situations.
Latest Posts
Latest Posts
-
How Long To Heat Water In Microwave
Jul 18, 2025
-
40 Oz Of Water Is How Many Cups
Jul 18, 2025
-
How Many Eighths In A Quarter Pound
Jul 18, 2025
-
Can The Sine Of An Angle Ever Equal 2
Jul 18, 2025
-
How Many Months Is A Hundred Days
Jul 18, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 Out Of 6 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.