What Is 5 To The Power Of 3
Kalali
Apr 12, 2025 · 5 min read
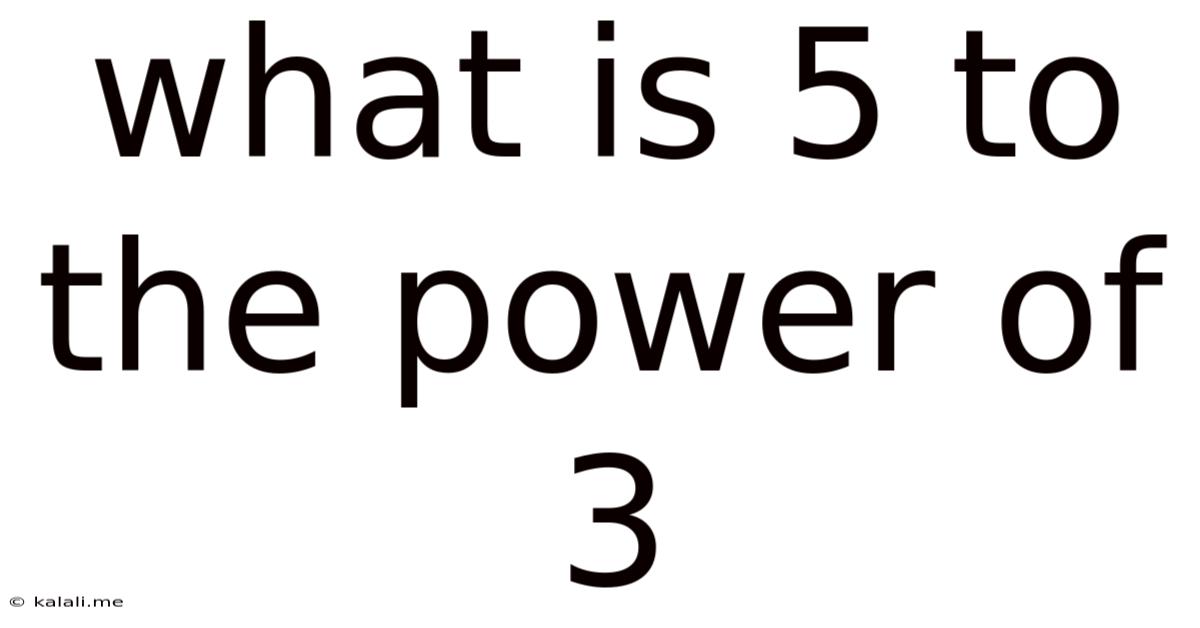
Table of Contents
What is 5 to the Power of 3? A Deep Dive into Exponentiation and its Applications
What is 5 to the power of 3? At first glance, this seems like a simple question with a straightforward answer. It is, but exploring this seemingly simple mathematical concept opens doors to a fascinating world of exponentiation, its practical applications, and its broader implications within mathematics and beyond. This article will delve deep into understanding 5 to the power of 3, exploring the underlying principles, real-world examples, and related mathematical concepts. We'll also touch on how to calculate higher powers and explore some of the intriguing properties of exponents.
Meta Description: This comprehensive guide explains what 5 to the power of 3 is, covering exponentiation principles, practical applications, and related mathematical concepts. Learn about calculating higher powers and discover the intriguing properties of exponents.
Understanding Exponentiation: The Basics
Before we tackle 5 to the power of 3, let's establish a firm understanding of exponentiation. Exponentiation is a mathematical operation that involves raising a number (the base) to a certain power (the exponent). It represents repeated multiplication of the base by itself. The general form is written as b<sup>e</sup>, where:
bis the base (the number being multiplied)eis the exponent (the number of times the base is multiplied by itself)
For instance, 2<sup>3</sup> means 2 multiplied by itself 3 times: 2 × 2 × 2 = 8. Similarly, 10<sup>2</sup> (ten squared) means 10 × 10 = 100. The exponent dictates the number of times the base is used as a factor in the multiplication.
Calculating 5 to the Power of 3
Now, let's address the central question: What is 5 to the power of 3 (5<sup>3</sup>)? This simply means multiplying 5 by itself three times:
5<sup>3</sup> = 5 × 5 × 5 = 125
Therefore, 5 to the power of 3 is 125. This seemingly simple calculation forms the basis for many complex mathematical operations and real-world applications.
Beyond the Basics: Exploring Higher Powers
Understanding 5<sup>3</sup> provides a foundation for exploring higher powers. Let's consider some examples:
- 5<sup>4</sup> (5 to the power of 4): This means 5 × 5 × 5 × 5 = 625
- 5<sup>5</sup> (5 to the power of 5): This means 5 × 5 × 5 × 5 × 5 = 3125
- 5<sup>0</sup> (5 to the power of 0): Any number raised to the power of 0 equals 1. Therefore, 5<sup>0</sup> = 1. This is a fundamental rule in exponentiation.
- 5<sup>-1</sup> (5 to the power of -1): A negative exponent indicates a reciprocal. Therefore, 5<sup>-1</sup> = 1/5 = 0.2.
- 5<sup>1/2</sup> (5 to the power of 1/2): This is equivalent to the square root of 5, approximately 2.236. Fractional exponents introduce the concept of roots.
Real-World Applications of Exponentiation
Exponentiation is not just an abstract mathematical concept; it has numerous practical applications across various fields:
-
Compound Interest: Calculating compound interest relies heavily on exponentiation. The formula involves raising the principal amount to a power determined by the interest rate and the number of compounding periods. Understanding this principle is crucial for financial planning and investment strategies.
-
Population Growth: Exponential growth models are frequently used to predict population growth in biology and demography. The formula utilizes exponentiation to project future populations based on current growth rates.
-
Radioactive Decay: Radioactive decay follows an exponential decay model. Exponentiation is used to determine the remaining amount of a radioactive substance after a specific time period, which is critical in various scientific applications, including carbon dating.
-
Computer Science: Exponentiation is fundamental in computer science, especially in cryptography. Algorithms like RSA encryption rely heavily on exponentiation with very large numbers to ensure data security.
-
Physics and Engineering: Many physical phenomena, such as the intensity of light or sound, follow exponential relationships. Exponentiation is essential for modeling and understanding these phenomena.
Related Mathematical Concepts
Understanding 5<sup>3</sup> opens doors to related mathematical concepts:
-
Logarithms: Logarithms are the inverse of exponentiation. If 5<sup>3</sup> = 125, then the logarithm base 5 of 125 is 3 (log<sub>5</sub>125 = 3). Logarithms are used extensively in various fields, including chemistry, physics, and engineering.
-
Scientific Notation: Scientific notation uses powers of 10 to represent very large or very small numbers concisely. For instance, 125,000 can be written as 1.25 × 10<sup>5</sup>.
-
Polynomial Equations: Polynomials involve terms with variables raised to various powers. Understanding exponentiation is vital for solving and analyzing polynomial equations, a fundamental concept in algebra.
Beyond Calculation: The Conceptual Understanding
While calculating 5<sup>3</sup> is straightforward, understanding the underlying principles of exponentiation is crucial. This understanding extends beyond simple calculations and provides a foundation for grasping more advanced mathematical concepts and applying them to real-world problems. It’s about comprehending the meaning of repeated multiplication and how this concept manifests itself in various scenarios, from financial growth to radioactive decay.
Practical Tips for Calculating Higher Powers
For higher powers of 5, or any other number, calculators or computer software are highly beneficial. However, even without these tools, understanding the process of repeated multiplication allows for manual calculation, albeit potentially more time-consuming for larger exponents. Remember to break down the calculation into smaller, manageable steps to prevent errors. For example, to calculate 5<sup>6</sup>, you can first calculate 5<sup>3</sup> (which we know is 125) and then square the result: 125 × 125 = 15625.
Conclusion: The Significance of 5 to the Power of 3
While the answer to "What is 5 to the power of 3?" is simply 125, the journey to arriving at that answer and exploring the associated concepts reveals the significance of exponentiation in mathematics and its far-reaching applications in various fields. From financial modeling to understanding natural phenomena, exponentiation plays a critical role in our ability to quantify, predict, and interpret the world around us. This seemingly simple calculation serves as a gateway to a deeper understanding of the power and versatility of mathematical principles. The more you delve into these concepts, the more you'll appreciate their elegance and wide-ranging influence.
Latest Posts
Latest Posts
-
Cuantos Kilometros Hay En Una Milla
Apr 18, 2025
-
18 Ounces Is How Many Grams
Apr 18, 2025
-
What Is 6 Out Of 10 As A Grade
Apr 18, 2025
-
How Many Feet Is 162 Centimeters
Apr 18, 2025
-
How Many Feet Is 180 Meters
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 To The Power Of 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.