What Is 5 To The Power Of 5
Kalali
Apr 05, 2025 · 5 min read
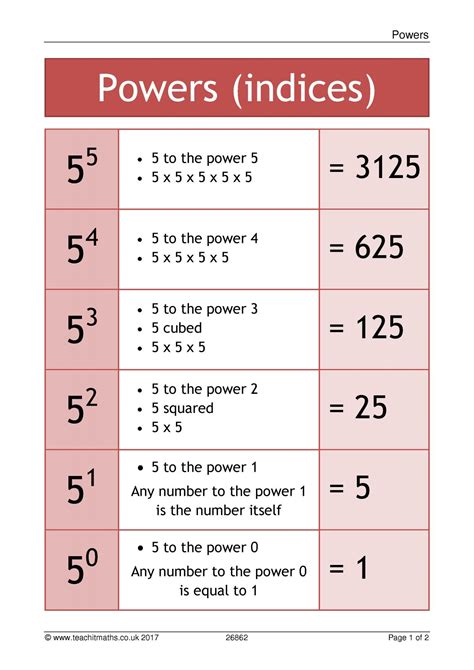
Table of Contents
What is 5 to the Power of 5? A Deep Dive into Exponentiation
The seemingly simple question, "What is 5 to the power of 5?" opens a door to a fascinating world of mathematics, specifically exponentiation. While the answer itself is straightforward enough to calculate, understanding the underlying concepts and applications provides a much richer understanding of this fundamental mathematical operation. This article will not only answer the question directly but will also explore the broader context of exponents, their properties, and their importance in various fields.
Understanding Exponentiation: Beyond Basic Multiplication
Exponentiation, often represented as b<sup>e</sup>, signifies repeated multiplication. Here, 'b' represents the base (the number being multiplied), and 'e' represents the exponent (the number of times the base is multiplied by itself). In simpler terms, 5 to the power of 5 (written as 5<sup>5</sup>) means 5 multiplied by itself five times.
Calculating 5<sup>5</sup>
The calculation is straightforward:
5<sup>5</sup> = 5 × 5 × 5 × 5 × 5 = 3125
Therefore, the answer to the question "What is 5 to the power of 5?" is 3125.
Exploring the Properties of Exponents
Understanding the properties of exponents is crucial for manipulating and solving equations involving exponential expressions. Here are some key properties:
Product of Powers:
When multiplying two numbers with the same base, you add the exponents: b<sup>m</sup> × b<sup>n</sup> = b<sup>(m+n)</sup>
For example: 5<sup>2</sup> × 5<sup>3</sup> = 5<sup>(2+3)</sup> = 5<sup>5</sup> = 3125
Quotient of Powers:
When dividing two numbers with the same base, you subtract the exponents: b<sup>m</sup> ÷ b<sup>n</sup> = b<sup>(m-n)</sup>
For example: 5<sup>5</sup> ÷ 5<sup>2</sup> = 5<sup>(5-2)</sup> = 5<sup>3</sup> = 125
Power of a Power:
When raising a power to another power, you multiply the exponents: (b<sup>m</sup>)<sup>n</sup> = b<sup>(m×n)</sup>
For example: (5<sup>2</sup>)<sup>3</sup> = 5<sup>(2×3)</sup> = 5<sup>6</sup> = 15625
Power of a Product:
When raising a product to a power, you raise each factor to that power: (ab)<sup>n</sup> = a<sup>n</sup>b<sup>n</sup>
For example: (5 × 2)<sup>3</sup> = 5<sup>3</sup> × 2<sup>3</sup> = 125 × 8 = 1000
Power of a Quotient:
When raising a quotient to a power, you raise both the numerator and the denominator to that power: (a/b)<sup>n</sup> = a<sup>n</sup>/b<sup>n</sup>
For example: (5/2)<sup>2</sup> = 5<sup>2</sup>/2<sup>2</sup> = 25/4 = 6.25
Understanding these properties allows for efficient simplification and manipulation of exponential expressions, significantly reducing the complexity of calculations.
Applications of Exponentiation: Beyond the Classroom
Exponentiation isn't just a theoretical concept confined to math textbooks. It has far-reaching applications across various fields:
Science and Engineering:
-
Exponential Growth and Decay: Many natural phenomena, such as population growth, radioactive decay, and compound interest, are modeled using exponential functions. Understanding exponentiation is crucial for analyzing and predicting these processes. For instance, bacterial growth can be represented using exponential functions, enabling scientists to model the spread of infections and develop appropriate treatments.
-
Physics: Exponents are fundamental to many physical laws and equations, including Newton's Law of Universal Gravitation and the equations governing electromagnetism. The intensity of light decreases exponentially with distance from the source – a principle vital in astronomy and optics.
-
Engineering: Exponential functions are used in various engineering disciplines, such as electrical engineering (circuit analysis), mechanical engineering (vibration analysis), and chemical engineering (reaction kinetics).
Computer Science:
-
Big O Notation: In computer science, Big O notation uses exponents to describe the time or space complexity of algorithms. This is crucial for evaluating algorithm efficiency and optimizing software performance. For example, an algorithm with O(n<sup>2</sup>) complexity has a runtime that scales quadratically with the input size (n).
-
Binary Numbers and Data Storage: Computer systems utilize binary (base-2) numbers, which inherently involve exponents of 2. Understanding exponents is critical for comprehending data storage and processing in computers.
Finance:
-
Compound Interest: The calculation of compound interest relies heavily on exponentiation. The formula for compound interest incorporates exponents to determine the future value of an investment based on the principal amount, interest rate, and time period.
-
Financial Modeling: Exponential functions are used extensively in financial modeling to predict future trends, assess risk, and make investment decisions.
Biology:
-
Population Growth: Exponential growth models are crucial for studying population dynamics in various species. Understanding population growth rates helps predict future population sizes and manage resources effectively.
-
DNA Replication: DNA replication involves exponential growth in the number of DNA strands during cell division. This principle is fundamental to understanding genetics and cellular processes.
Beyond 5<sup>5</sup>: Exploring Larger Exponents
While 5<sup>5</sup> is relatively easy to calculate, consider the implications of much larger exponents. The value of numbers grows incredibly rapidly as the exponent increases. This rapid growth highlights the power and significance of exponentiation in representing vast quantities and phenomena. This rapid growth is also what makes exponential functions so useful in modelling phenomena that change rapidly over time.
Conclusion: The Significance of a Simple Calculation
The seemingly simple calculation of 5<sup>5</sup> = 3125 opens a gateway to a vast and significant mathematical concept. Exponentiation is not merely a mathematical operation; it's a fundamental tool used across diverse fields, from understanding natural phenomena to designing efficient algorithms and modeling complex financial systems. By grasping the principles and properties of exponentiation, we gain a deeper understanding of the world around us and the power of mathematical modeling. The seemingly simple answer of 3125 represents a gateway to a much larger and more significant concept within mathematics and its practical applications. The importance of understanding exponents, therefore, cannot be overstated. It forms a cornerstone of advanced mathematical concepts and is essential for anyone seeking a deeper understanding of the quantitative world around them.
Latest Posts
Latest Posts
-
1 6 M In Feet And Inches
Apr 05, 2025
-
How Many Inches Is 124 Cm
Apr 05, 2025
-
Can A Sedimentary Rock Become An Igneous Rock
Apr 05, 2025
-
How Many Feet Is 104 In
Apr 05, 2025
-
How Tall Is 54 In In Feet
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 To The Power Of 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
