What Is 6 Out Of 8 In Percentage
Kalali
Apr 27, 2025 · 5 min read
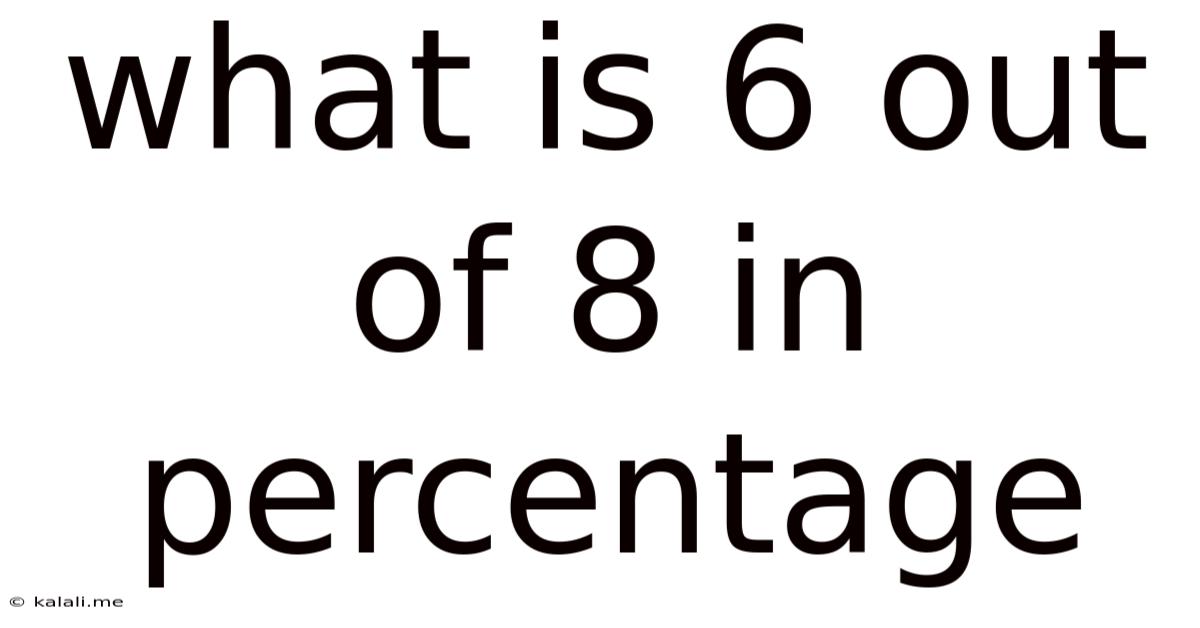
Table of Contents
What is 6 out of 8 in Percentage? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating discounts and taxes to understanding statistics and financial reports. This comprehensive guide will delve into how to determine "6 out of 8" as a percentage, explain the underlying concepts, and provide practical examples to solidify your understanding. We'll explore different methods, addressing potential confusion and offering tips for accurate and efficient calculations. This article aims to be your definitive resource for mastering percentage calculations, particularly focusing on the specific problem of converting fractions to percentages.
Understanding the Basics: Fractions, Decimals, and Percentages
Before we tackle the specific problem, let's establish a strong foundation. Three interconnected concepts are central to this process: fractions, decimals, and percentages.
-
Fractions: A fraction represents a part of a whole. In our example, "6 out of 8" is represented as the fraction 6/8. The top number (6) is the numerator, representing the part, and the bottom number (8) is the denominator, representing the whole.
-
Decimals: Decimals are another way to represent parts of a whole. They use a base-ten system, with the decimal point separating the whole number from the fractional part. To convert a fraction to a decimal, you divide the numerator by the denominator.
-
Percentages: Percentages express a fraction or decimal as a portion of 100. The "%" symbol represents "per hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Method 1: Converting the Fraction to a Decimal, Then to a Percentage
This is arguably the most straightforward method. We'll break it down step-by-step:
-
Convert the fraction to a decimal: Divide the numerator (6) by the denominator (8): 6 ÷ 8 = 0.75
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.75 × 100 = 75
-
Add the percentage symbol: The final answer is 75%.
Therefore, 6 out of 8 is equal to 75%.
Method 2: Simplifying the Fraction First
Sometimes, simplifying the fraction before converting to a decimal can make the calculation easier. This is particularly helpful when dealing with larger numbers.
-
Simplify the fraction: Find the greatest common divisor (GCD) of the numerator (6) and the denominator (8). The GCD of 6 and 8 is 2. Divide both the numerator and denominator by the GCD: 6 ÷ 2 = 3 and 8 ÷ 2 = 4. This simplifies the fraction to 3/4.
-
Convert the simplified fraction to a decimal: Divide the numerator (3) by the denominator (4): 3 ÷ 4 = 0.75
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.75 × 100 = 75
-
Add the percentage symbol: The final answer remains 75%.
Method 3: Using Proportions
This method is more conceptually based and helps build a deeper understanding of percentages. It involves setting up a proportion:
-
We know that 6 out of 8 is equivalent to 'x' out of 100 (where 'x' represents the percentage).
-
This can be written as a proportion: 6/8 = x/100
-
To solve for 'x', cross-multiply: 8x = 600
-
Divide both sides by 8: x = 75
-
Therefore, 6 out of 8 is 75%.
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in numerous real-life situations. Here are a few examples:
-
Sales and Discounts: A store offers a 25% discount on an item. If the original price is $80, the discount amount is 25% of $80, which is (25/100) * $80 = $20. The final price would be $80 - $20 = $60. This is directly related to our example; if you bought 6 out of 8 items, you effectively received a 25% discount compared to buying all 8.
-
Test Scores: If you answered 6 out of 8 questions correctly on a quiz, your score is 75%. This helps you understand your performance and identify areas for improvement.
-
Financial Calculations: Percentage calculations are essential for understanding interest rates, tax rates, and investment returns. For example, calculating simple interest involves using percentages.
-
Data Analysis: Percentages are frequently used in representing data in graphs, charts, and reports. Understanding them allows for easier interpretation and comparison of data.
-
Cooking and Baking: Recipes often use percentages for ingredients, particularly in baking where precise measurements are crucial.
-
Survey Results: When analyzing survey results, percentages provide a concise way to represent the proportion of respondents who chose a particular option.
Addressing Common Mistakes and Challenges
While percentage calculations seem straightforward, several common mistakes can lead to inaccurate results:
-
Incorrectly Converting Fractions to Decimals: Ensure you divide the numerator by the denominator correctly.
-
Forgetting to Multiply by 100: Remember that the final step is to multiply the decimal by 100 to obtain the percentage.
-
Misinterpreting the Problem: Carefully read the question and identify the correct numerator and denominator.
-
Rounding Errors: When dealing with decimals, be mindful of rounding errors which can affect the final result, especially in complex calculations. It's often better to work with fractions until the final conversion to a percentage.
Advanced Concepts and Further Exploration
For those wanting a deeper understanding, explore these advanced concepts:
-
Percentage Increase and Decrease: Learn how to calculate percentage changes, which is crucial in finance and statistics.
-
Compound Interest: This concept builds upon simple interest and is vital for understanding long-term investments.
-
Percentage Points: Understand the difference between a percentage change and percentage points.
-
Working with Percentages of Percentages: This involves nested percentage calculations, often encountered in financial modeling.
Conclusion:
Determining that 6 out of 8 represents 75% is a relatively simple calculation, yet understanding the underlying principles of fractions, decimals, and percentages is critical for applying this knowledge to more complex scenarios. By mastering these foundational concepts and avoiding common mistakes, you'll be well-equipped to tackle various percentage problems with confidence and accuracy. This skill is invaluable across numerous disciplines, making it a worthwhile investment of your time and effort. Remember to practice regularly, using various methods, to solidify your understanding and build fluency in percentage calculations.
Latest Posts
Latest Posts
-
Cuanto Es 30 Pulgadas En Pies
Apr 27, 2025
-
Cuanto Es 23 Grados Centigrados En Fahrenheit
Apr 27, 2025
-
What Is 30 Dollars With 40 Percent Off
Apr 27, 2025
-
6 Pies 5 Pulgadas A Metros
Apr 27, 2025
-
What Percent Is 5 Out Of 8
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Is 6 Out Of 8 In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.