What Is 8/10 As A Percentage
Kalali
Apr 22, 2025 · 6 min read
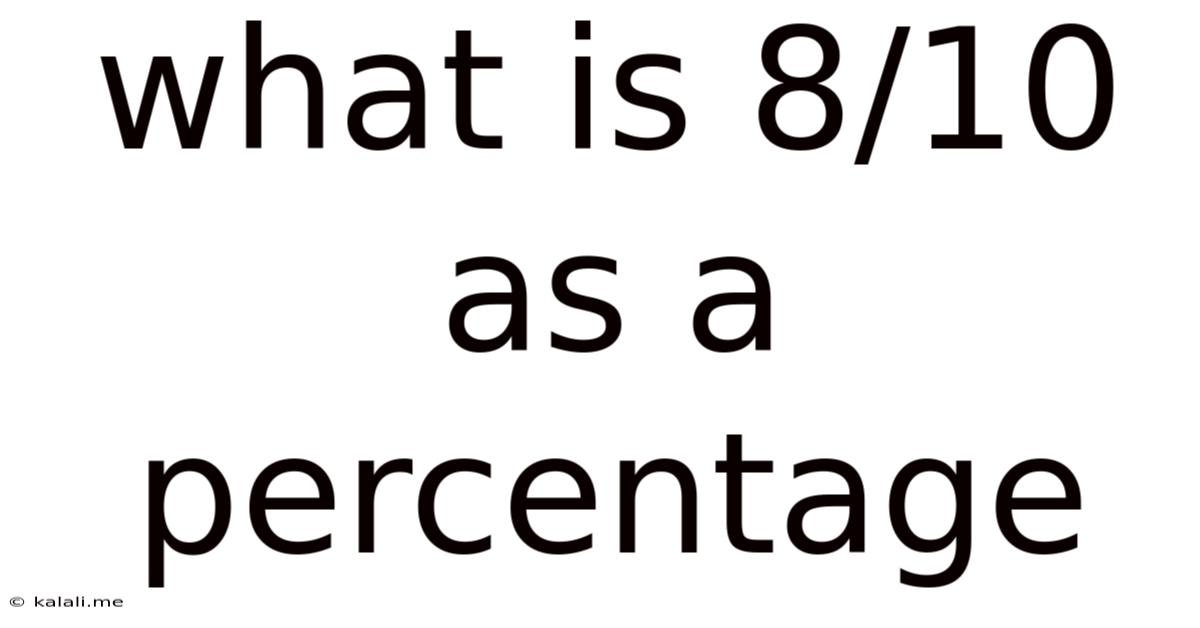
Table of Contents
What is 8/10 as a Percentage? A Comprehensive Guide to Fraction-to-Percentage Conversion
Understanding how to convert fractions to percentages is a fundamental skill in mathematics, with applications spanning various fields from finance and statistics to everyday life. This article dives deep into converting the fraction 8/10 into a percentage, exploring the underlying principles, different methods of calculation, and real-world examples. We’ll also touch upon related concepts and offer practical tips to improve your understanding and calculation skills. This in-depth guide will ensure you not only know the answer but also fully grasp the process.
Meta Description: Learn how to convert the fraction 8/10 into a percentage. This comprehensive guide explains various methods, provides real-world examples, and explores related mathematical concepts, equipping you with a solid understanding of fraction-to-percentage conversion.
Understanding Fractions and Percentages
Before diving into the specific conversion of 8/10, let's establish a clear understanding of fractions and percentages.
A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of parts in the whole. For example, in the fraction 8/10, 8 represents the number of parts and 10 represents the total number of equal parts.
A percentage, denoted by the symbol %, represents a fraction of 100. It indicates how many parts out of 100 constitute a particular portion of a whole. Percentages are widely used to express proportions, rates, and changes. For instance, 50% means 50 out of 100, or one-half.
Method 1: Direct Conversion using the Decimal Equivalent
The most straightforward method to convert 8/10 to a percentage involves converting the fraction to its decimal equivalent first. This involves dividing the numerator by the denominator:
8 ÷ 10 = 0.8
Once we have the decimal equivalent (0.8), we multiply it by 100 to express it as a percentage:
0.8 x 100 = 80%
Therefore, 8/10 is equal to 80%. This method is particularly useful for simple fractions where the division is easy to perform mentally or with a basic calculator.
Method 2: Proportion Method
This method leverages the concept of proportions to find the equivalent percentage. We can set up a proportion:
8/10 = x/100
Where 'x' represents the percentage we want to find. To solve for x, we cross-multiply:
10x = 800
Then, we divide both sides by 10:
x = 80
Therefore, 8/10 is equal to 80%. This method is particularly helpful in understanding the underlying relationship between fractions and percentages. It clearly demonstrates that a percentage is simply a fraction with a denominator of 100.
Method 3: Simplifying the Fraction First
Sometimes, simplifying the fraction before converting to a percentage can make the calculation easier. In this case, 8/10 can be simplified by dividing both the numerator and denominator by their greatest common divisor, which is 2:
8 ÷ 2 = 4 10 ÷ 2 = 5
This simplifies the fraction to 4/5. Now, we can convert 4/5 to a decimal:
4 ÷ 5 = 0.8
And then multiply by 100 to get the percentage:
0.8 x 100 = 80%
This method demonstrates that simplifying the fraction doesn't alter its value; it merely presents it in a simpler form, making the conversion process more manageable.
Real-World Applications of 8/10 (80%)
Understanding the conversion of 8/10 to 80% has practical applications in many areas:
- Academic Performance: If a student answers 8 out of 10 questions correctly on a test, their score is 80%.
- Sales and Discounts: A store offering an 80% discount means customers pay only 20% of the original price.
- Financial Investments: An investment that yields an 80% return signifies a substantial profit.
- Surveys and Polls: If 8 out of 10 respondents agree with a particular statement, the agreement level is 80%.
- Statistical Analysis: In data analysis, 80% might represent a significant portion of a dataset or a key threshold.
- Project Completion: If 8 out of 10 project milestones are achieved, the project is 80% complete.
Expanding the Understanding: Converting Other Fractions to Percentages
The principles discussed above apply to converting any fraction to a percentage. Let's consider a few more examples:
- 1/4: 1 ÷ 4 = 0.25 x 100 = 25%
- 3/5: 3 ÷ 5 = 0.6 x 100 = 60%
- 7/8: 7 ÷ 8 = 0.875 x 100 = 87.5%
- 1/2: 1 ÷ 2 = 0.5 x 100 = 50%
These examples illustrate the versatility of the conversion methods and their applicability to different fractions. Remember that the core principle remains consistent: divide the numerator by the denominator and multiply the result by 100 to obtain the percentage.
Dealing with Improper Fractions and Mixed Numbers
While the examples above focus on proper fractions (where the numerator is smaller than the denominator), the same principles apply to improper fractions (where the numerator is larger than the denominator) and mixed numbers (a combination of a whole number and a fraction).
For example, consider the improper fraction 12/10:
12 ÷ 10 = 1.2 x 100 = 120%
This signifies that the value exceeds 100%, which is perfectly valid in certain contexts.
Similarly, a mixed number like 1 2/5 can be converted to an improper fraction first: (5*1 + 2)/5 = 7/5. Then convert to percentage:
7 ÷ 5 = 1.4 x 100 = 140%
Practical Tips for Efficient Conversion
- Mastering basic division: Proficiency in division is crucial for efficient fraction-to-percentage conversion. Practice with various fractions to improve your speed and accuracy.
- Utilizing calculators: Calculators are valuable tools, particularly for complex fractions. Familiarize yourself with their functionalities.
- Understanding decimal places: Accuracy in decimal placement is vital when multiplying by 100. Pay attention to the decimal point.
- Simplifying fractions: Simplifying fractions before conversion often simplifies the subsequent calculations.
- Practice makes perfect: Consistent practice with diverse fraction-to-percentage conversions solidifies your understanding and improves your skills.
Conclusion: Beyond the Basics of 8/10
This comprehensive guide has not only provided the answer to "What is 8/10 as a percentage?" (80%) but also equipped you with the fundamental understanding and practical skills to confidently convert any fraction to its percentage equivalent. Remember that mastering this skill is not just about knowing the formula; it's about understanding the underlying mathematical concepts and their wide-ranging applications in various aspects of life. Continue practicing, and you'll find yourself effortlessly converting fractions to percentages in no time. This essential skill will serve you well in academic pursuits, professional endeavors, and everyday situations.
Latest Posts
Latest Posts
-
What Is Lcm Of 9 And 12
Apr 22, 2025
-
48 Feet Is How Many Inches
Apr 22, 2025
-
108 Minutes Is How Many Hours
Apr 22, 2025
-
How Much Is 20 Fl Oz
Apr 22, 2025
-
What Is 40 Out Of 50 As A Percentage
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about What Is 8/10 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.