What Is The Decimal For 5/12
Kalali
Apr 25, 2025 · 5 min read
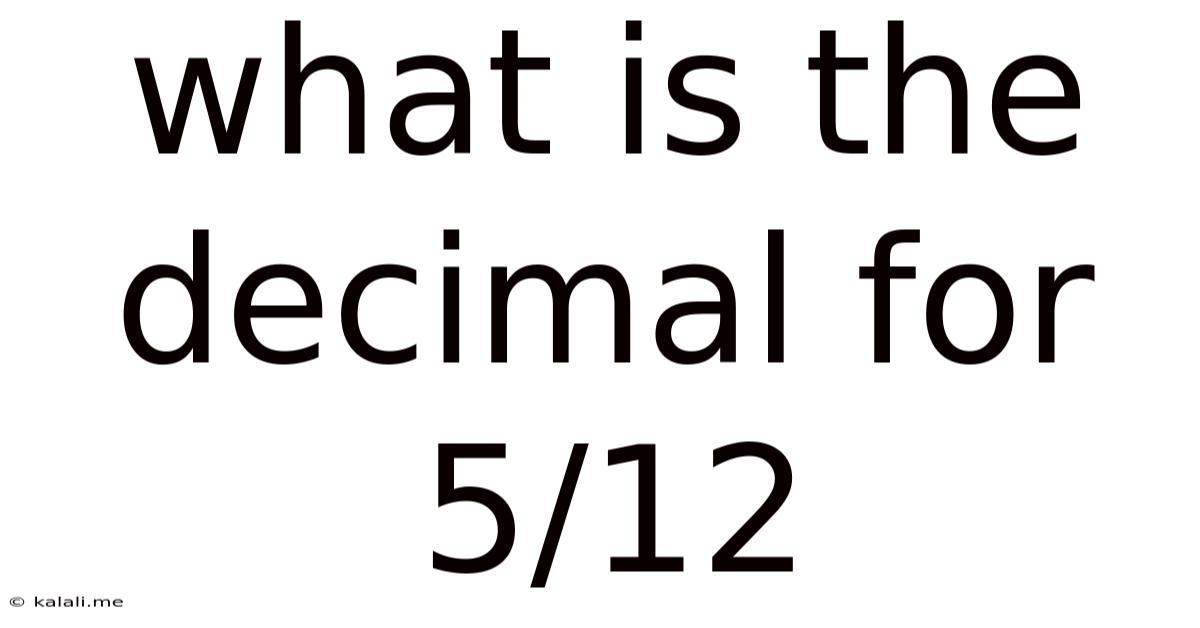
Table of Contents
What is the Decimal for 5/12? A Deep Dive into Fraction-to-Decimal Conversion
Finding the decimal equivalent of a fraction is a fundamental skill in mathematics, with applications spanning various fields from finance and engineering to everyday calculations. This article delves into the process of converting the fraction 5/12 into its decimal representation, exploring different methods and highlighting the underlying mathematical principles. We'll also touch upon the broader context of fraction-to-decimal conversion, offering practical tips and examples. Understanding this seemingly simple conversion lays the groundwork for more complex mathematical operations.
Meta Description: Learn how to convert the fraction 5/12 into its decimal equivalent. This comprehensive guide explores various methods, including long division and calculator use, providing a clear understanding of the process and its applications.
Understanding Fractions and Decimals
Before diving into the conversion of 5/12, let's briefly review the concepts of fractions and decimals. A fraction represents a part of a whole, expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). For example, in the fraction 5/12, 5 is the numerator and 12 is the denominator. This signifies 5 parts out of a total of 12 equal parts.
A decimal is a number expressed in base-10, using a decimal point to separate the whole number part from the fractional part. Decimals are a convenient way to represent fractions, especially when performing calculations. The decimal system is based on powers of 10, with each digit to the right of the decimal point representing a decreasing power of 10 (tenths, hundredths, thousandths, etc.).
Method 1: Long Division
The most fundamental method for converting a fraction to a decimal is through long division. This method involves dividing the numerator (5) by the denominator (12).
-
Set up the long division: Write the numerator (5) inside the long division symbol and the denominator (12) outside. Since 12 is larger than 5, we add a decimal point and a zero to the numerator (5.0).
-
Perform the division: 12 goes into 50 four times (12 x 4 = 48). Write the 4 above the 0 in the quotient. Subtract 48 from 50, leaving a remainder of 2.
-
Add another zero: Bring down another zero to the remainder (20).
-
Continue the division: 12 goes into 20 one time (12 x 1 = 12). Write the 1 in the quotient next to the 4. Subtract 12 from 20, leaving a remainder of 8.
-
Repeat the process: Bring down another zero (80). 12 goes into 80 six times (12 x 6 = 72). Write the 6 in the quotient. Subtract 72 from 80, leaving a remainder of 8.
-
Observe the pattern: Notice that the remainder 8 repeats. This indicates that the decimal representation of 5/12 is a repeating decimal.
-
Express the decimal: The decimal representation of 5/12 is 0.416666... This can be written as 0.416̅, where the bar indicates the repeating digit.
Therefore, using long division, we find that the decimal equivalent of 5/12 is approximately 0.4167 (rounded to four decimal places).
Method 2: Using a Calculator
A simpler, albeit less instructive, method involves using a calculator. Simply divide the numerator (5) by the denominator (12). Most calculators will display the result as a decimal, either a terminating or repeating decimal. In this case, the calculator will show a repeating decimal, 0.416666..., which again is approximately 0.4167 when rounded.
Understanding Repeating Decimals
The decimal representation of 5/12 is a repeating decimal, meaning a digit or sequence of digits repeats indefinitely. This is a characteristic of many fractions, particularly those with denominators that are not factors of powers of 10 (i.e., 10, 100, 1000, etc.). Understanding repeating decimals is crucial for accurate calculations and representation of fractional values.
Practical Applications
The conversion of fractions to decimals is essential in many practical situations:
-
Financial Calculations: Calculating percentages, interest rates, and proportions often requires converting fractions to decimals. For example, calculating 5/12 of a year's interest would require converting 5/12 to its decimal equivalent.
-
Engineering and Construction: Precision measurements and calculations in engineering and construction frequently involve converting fractions to decimals for greater accuracy.
-
Scientific Measurements: Scientific measurements often involve fractions, and converting them to decimals facilitates comparisons and calculations.
-
Everyday Calculations: Many everyday calculations, such as splitting a bill or measuring ingredients, may involve fractions, and converting them to decimals can simplify the process.
Further Exploration: Converting Other Fractions
The methods described above can be applied to convert any fraction to its decimal equivalent. Let's explore a few more examples:
-
1/4: Dividing 1 by 4 yields 0.25, a terminating decimal.
-
1/3: Dividing 1 by 3 yields 0.333..., a repeating decimal.
-
7/8: Dividing 7 by 8 yields 0.875, a terminating decimal.
-
2/7: Dividing 2 by 7 yields 0.285714285714..., a repeating decimal with a longer repeating sequence.
Converting Decimals Back to Fractions
It's also useful to understand the reverse process – converting decimals back into fractions. For terminating decimals, this involves writing the decimal as a fraction with a denominator that is a power of 10 and then simplifying the fraction. For repeating decimals, the process is more complex and may involve algebraic manipulation.
Conclusion
Converting the fraction 5/12 to its decimal equivalent (approximately 0.4167) involves a straightforward process, whether using long division or a calculator. Understanding this conversion is fundamental to a variety of mathematical applications and highlights the relationship between fractions and decimals. The ability to comfortably convert between these two representations is a valuable skill for anyone working with numbers, regardless of their field of study or profession. Remember, the key is to grasp the underlying mathematical principles, enabling you to confidently tackle similar conversions in the future. This understanding empowers you to approach more complex mathematical challenges with greater ease and precision.
Latest Posts
Latest Posts
-
How To Find X Intercept In Vertex Form
Apr 25, 2025
-
Greatest Common Factor Of 30 And 42
Apr 25, 2025
-
What Percent Of 80 Is 10
Apr 25, 2025
-
Study Guide For Photosynthesis And Cellular Respiration
Apr 25, 2025
-
How Many Oints In A Gallon
Apr 25, 2025
Related Post
Thank you for visiting our website which covers about What Is The Decimal For 5/12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.