What Is The Least Common Multiple Of 10 And 15
Kalali
Apr 02, 2025 · 5 min read
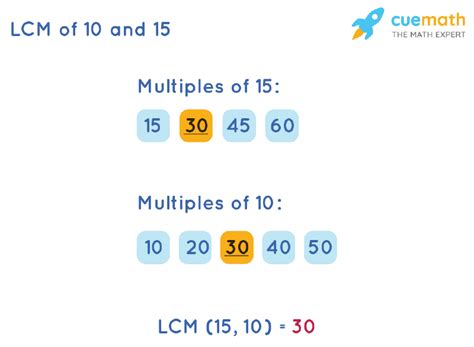
Table of Contents
What is the Least Common Multiple (LCM) of 10 and 15? A Deep Dive into Number Theory
Finding the least common multiple (LCM) might seem like a simple arithmetic problem, but understanding the concept thoroughly opens doors to more complex mathematical concepts and has practical applications in various fields. This article delves into the question: What is the least common multiple of 10 and 15? We'll explore multiple methods for calculating the LCM, discuss its significance in mathematics, and illustrate its real-world applications.
Understanding Least Common Multiple (LCM)
The least common multiple (LCM) of two or more integers is the smallest positive integer that is divisible by all the integers. In simpler terms, it's the smallest number that contains all the integers as factors. For example, the LCM of 2 and 3 is 6 because 6 is the smallest number divisible by both 2 and 3.
This concept differs from the greatest common divisor (GCD), which is the largest number that divides both integers without leaving a remainder. While seemingly opposite, LCM and GCD are intimately related, a relationship we'll explore later.
Calculating the LCM of 10 and 15: Method 1 - Listing Multiples
The most straightforward method to find the LCM of 10 and 15 involves listing the multiples of each number until a common multiple is found.
Multiples of 10: 10, 20, 30, 40, 50, 60, 70...
Multiples of 15: 15, 30, 45, 60, 75, 90...
By comparing the lists, we see that the smallest common multiple is 30. Therefore, the LCM(10, 15) = 30. This method is simple for small numbers but becomes less efficient for larger numbers.
Calculating the LCM of 10 and 15: Method 2 - Prime Factorization
A more efficient method, especially for larger numbers, involves using prime factorization. Prime factorization breaks down a number into its prime factors – numbers divisible only by 1 and themselves.
- Prime factorization of 10: 2 x 5
- Prime factorization of 15: 3 x 5
To find the LCM using prime factorization, we identify the highest power of each prime factor present in the factorizations of both numbers.
- The prime factors are 2, 3, and 5.
- The highest power of 2 is 2¹ = 2
- The highest power of 3 is 3¹ = 3
- The highest power of 5 is 5¹ = 5
Multiplying these highest powers together gives us the LCM: 2 x 3 x 5 = 30. This method is more systematic and scalable for larger numbers.
Calculating the LCM of 10 and 15: Method 3 - Using the GCD
The LCM and GCD are closely related. There's a formula that connects them:
LCM(a, b) x GCD(a, b) = a x b
Where 'a' and 'b' are the two numbers.
First, let's find the GCD of 10 and 15. The common divisors of 10 and 15 are 1 and 5. Therefore, the GCD(10, 15) = 5.
Now, using the formula:
LCM(10, 15) x 5 = 10 x 15
LCM(10, 15) = (10 x 15) / 5 = 30
This method leverages the relationship between LCM and GCD, offering another efficient approach.
Significance of LCM in Mathematics
The LCM holds significant importance in various mathematical concepts:
-
Fraction Addition and Subtraction: Finding a common denominator when adding or subtracting fractions requires determining the LCM of the denominators. This ensures accurate calculations.
-
Modular Arithmetic: LCM plays a crucial role in solving problems related to modular arithmetic, a system of arithmetic for integers where numbers "wrap around" upon reaching a certain value (the modulus).
-
Number Theory: LCM is fundamental in number theory, helping to solve problems involving divisibility, congruences, and other number-theoretic concepts.
-
Abstract Algebra: The concept of LCM extends to more abstract algebraic structures.
Real-World Applications of LCM
The practical applications of LCM extend beyond theoretical mathematics:
-
Scheduling: Imagine two buses departing from the same station, one every 10 minutes and the other every 15 minutes. The LCM (30 minutes) indicates when both buses will depart simultaneously again. This is useful in scheduling tasks, events, and transportation.
-
Manufacturing: In manufacturing processes, machines may operate at different cycles. The LCM helps determine when all machines will complete a cycle simultaneously, optimizing production efficiency.
-
Construction: In construction projects, materials may be delivered at different intervals. Determining the LCM helps plan material delivery schedules effectively.
-
Music: In music theory, the LCM is used to determine the least common multiple of rhythmic patterns, crucial for creating harmonious musical pieces.
Beyond the Basics: Extending the Concept of LCM
The LCM concept isn't limited to just two numbers. You can extend it to find the LCM of three or more numbers. For example, finding the LCM of 10, 15, and 20 involves using prime factorization or similar methods to determine the smallest number divisible by all three. The process remains similar, but the calculations become more involved.
Conclusion: Mastering LCM for Practical and Mathematical Success
Understanding the least common multiple is crucial for both mathematical proficiency and practical problem-solving. While the example of finding the LCM of 10 and 15 serves as an excellent introduction, the methods described – listing multiples, prime factorization, and using the GCD – are applicable to a wide range of numbers and scenarios. Mastering these methods not only enhances your mathematical skills but also equips you to tackle real-world challenges involving scheduling, manufacturing, and other fields effectively. The seemingly simple concept of LCM unlocks deeper mathematical understanding and practical applications, solidifying its importance in both academic and real-world contexts. By understanding the different methods and their significance, you can confidently tackle LCM problems of any complexity.
Latest Posts
Latest Posts
-
Find All Complex Zeros Of The Polynomial Function
Apr 03, 2025
-
How Many Cups To 20 Oz
Apr 03, 2025
-
What Is 230 Degrees Celsius In Fahrenheit
Apr 03, 2025
-
Cuanto Es El 25 De 100
Apr 03, 2025
-
How Many Gallons Are In 3 Liters
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Is The Least Common Multiple Of 10 And 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
