What Is The Percent Of 5/9
Kalali
Apr 25, 2025 · 4 min read
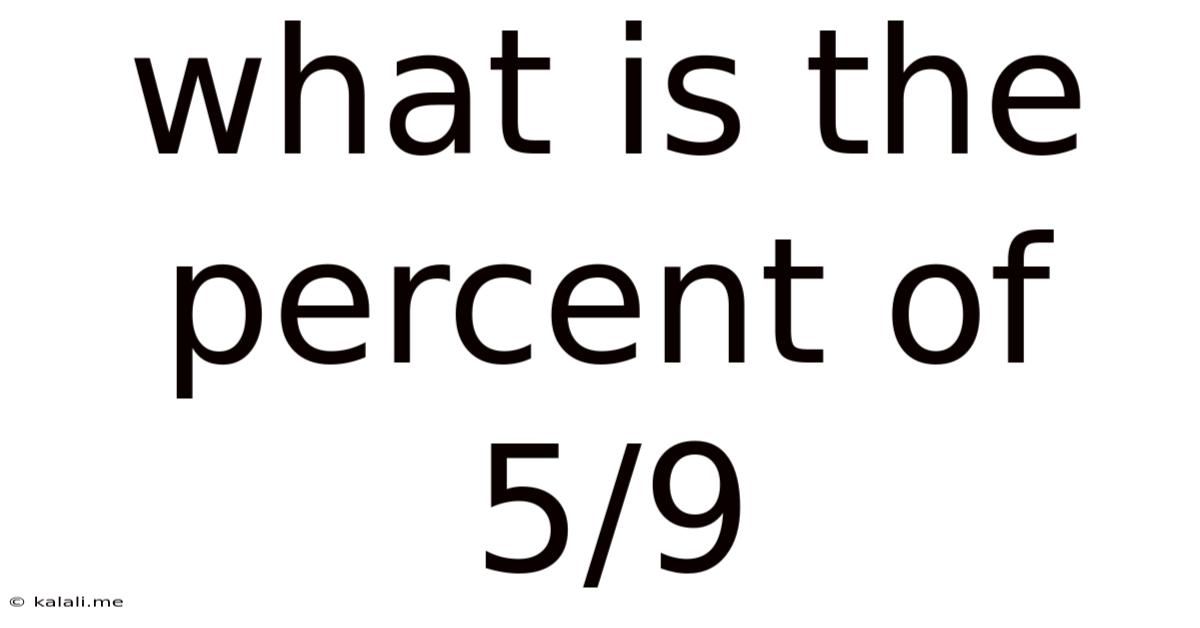
Table of Contents
What is the Percent of 5/9? A Deep Dive into Fractions, Decimals, and Percentages
This article will comprehensively explore the question, "What is the percent of 5/9?" We'll not only provide the answer but delve into the underlying mathematical concepts, offering various methods to solve similar problems and highlighting practical applications. Understanding how to convert fractions to percentages is a fundamental skill with widespread use in everyday life, from calculating discounts to understanding financial reports.
Meta Description: Learn how to convert fractions to percentages. This comprehensive guide explains how to calculate the percentage equivalent of 5/9, providing multiple methods and practical applications.
Understanding Fractions, Decimals, and Percentages
Before we tackle the specific problem of converting 5/9 to a percentage, let's briefly review the core concepts:
-
Fractions: Represent a part of a whole. They consist of a numerator (the top number) and a denominator (the bottom number). 5/9 means 5 parts out of a total of 9 parts.
-
Decimals: Represent a part of a whole using a base-10 system. They are expressed using a decimal point, separating the whole number from the fractional part.
-
Percentages: Represent a part of a whole as a fraction of 100. The symbol "%" signifies "per hundred". For instance, 25% means 25 out of 100, or 25/100.
Method 1: Direct Conversion using Division
The most straightforward method to convert a fraction to a percentage is through division:
-
Divide the numerator by the denominator: Divide 5 by 9. This gives you 0.5555... (a repeating decimal).
-
Multiply the result by 100: Multiply 0.5555... by 100. This gives you 55.555...
-
Add the percentage symbol: The final answer is approximately 55.56%. We round to two decimal places for practical purposes.
Therefore, 5/9 is approximately equal to 55.56%.
Method 2: Finding an Equivalent Fraction with a Denominator of 100
This method involves finding an equivalent fraction where the denominator is 100. This directly translates to a percentage since percentages are fractions out of 100.
However, this method isn't always practical, especially with fractions that don't easily simplify to a denominator of 100. In the case of 5/9, we can't easily find a whole number to multiply both the numerator and denominator by to achieve a denominator of 100. Let's explore why:
To get a denominator of 100, we need to find a number that, when multiplied by 9, equals 100. This number would be 100/9, which is not a whole number. Therefore, this method is less efficient for this specific problem.
Method 3: Using a Calculator
Modern calculators readily handle fraction-to-percentage conversions. Simply enter the fraction (5/9) and use the percentage function or convert it to a decimal and then multiply by 100. This offers speed and accuracy, especially when dealing with more complex fractions.
Understanding Repeating Decimals
Notice that when we divide 5 by 9, we obtain a repeating decimal (0.555...). This is a characteristic of certain fractions where the denominator contains prime factors other than 2 and 5. These repeating decimals can be expressed using a vinculum (a horizontal bar above the repeating digits), such as 0.5̅. When converting to a percentage, we typically round the decimal to a suitable number of decimal places for practical use.
Practical Applications of Percentage Conversions
The ability to convert fractions to percentages is incredibly useful in various contexts:
-
Sales and Discounts: Calculating discounts offered in stores. For instance, a 5/9 discount means a roughly 55.56% discount.
-
Financial Analysis: Understanding financial statements, calculating interest rates, and analyzing profit margins.
-
Statistics and Data Analysis: Representing data as percentages for easier interpretation and comparison.
-
Scientific Calculations: Expressing experimental results and probabilities as percentages.
-
Everyday Life: Dividing tasks, sharing resources, and understanding proportions.
Further Exploration: Working with More Complex Fractions
The techniques outlined above can be applied to more complex fractions. For example, let's consider the fraction 17/25:
-
Divide the numerator by the denominator: 17 ÷ 25 = 0.68
-
Multiply the result by 100: 0.68 × 100 = 68
-
Add the percentage symbol: 68%
Therefore, 17/25 is equal to 68%. This demonstrates the versatility of the direct division method. Another example: Let’s find the percentage of 7/8:
-
Divide the numerator by the denominator: 7 ÷ 8 = 0.875
-
Multiply the result by 100: 0.875 × 100 = 87.5
-
Add the percentage symbol: 87.5%
Therefore, 7/8 equals 87.5%.
Conclusion: Mastering Fraction-to-Percentage Conversions
Converting fractions to percentages is a fundamental mathematical skill with wide-ranging practical applications. While calculators provide a quick solution, understanding the underlying principles of fractions, decimals, and percentages is crucial. The direct division method offers a reliable and versatile approach for solving various problems, providing a strong foundation for tackling more complex mathematical challenges. Remember to round your answers appropriately depending on the context and level of precision needed. Mastering this conversion strengthens your mathematical abilities and enhances your comprehension of quantitative data encountered daily.
Latest Posts
Latest Posts
-
Are All Square Roots Irrational Numbers
Apr 25, 2025
-
31 Centimeters Is How Many Inches
Apr 25, 2025
-
What Is The Lcm Of 14 And 8
Apr 25, 2025
-
Cuales Son Los Multiplos De 8
Apr 25, 2025
-
How Much Is 80 In In Feet
Apr 25, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 5/9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.