What Is The Percent Of 7 10
Kalali
Apr 26, 2025 · 5 min read
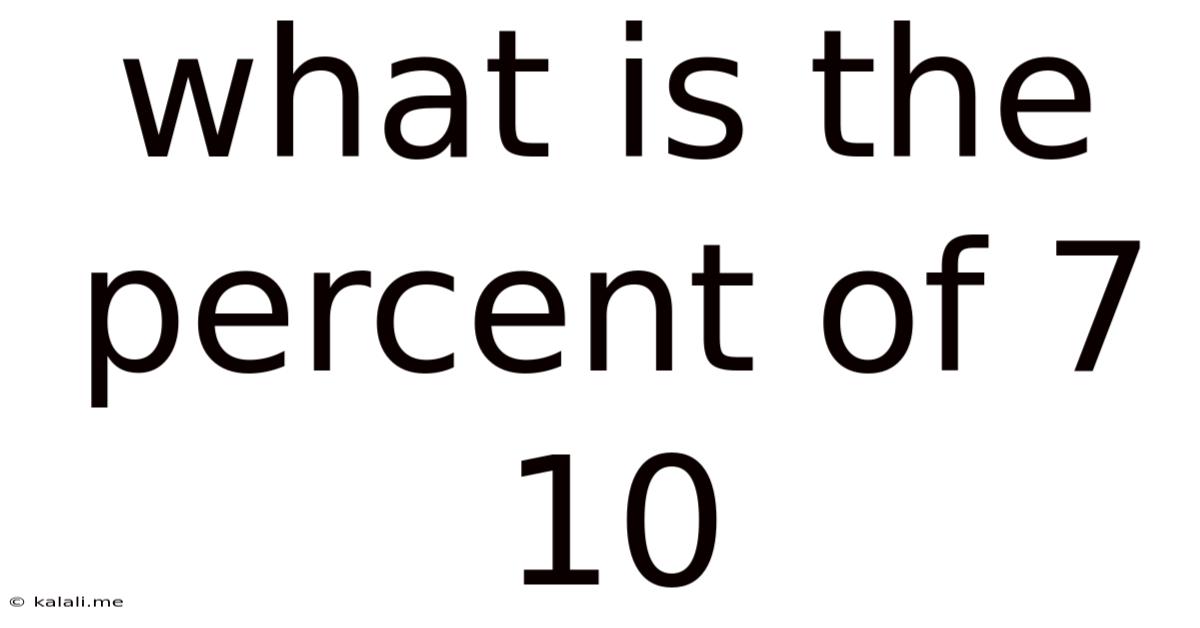
Table of Contents
What is the Percent of 7/10? A Deep Dive into Fractions, Decimals, and Percentages
What is 7/10 as a percentage? This seemingly simple question opens the door to a broader understanding of fundamental mathematical concepts crucial for everyday life, from calculating discounts to understanding statistics. This article will not only answer that question directly but also explore the interconnectedness of fractions, decimals, and percentages, offering a comprehensive guide for beginners and a helpful refresher for those needing a quick recap.
Understanding Fractions, Decimals, and Percentages: The Trifecta of Representation
Before diving into the specific calculation, let's establish a solid foundation. These three concepts – fractions, decimals, and percentages – are different ways of representing the same underlying value, a portion of a whole.
-
Fractions: Fractions represent a part of a whole using a numerator (the top number) and a denominator (the bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of parts the whole is divided into. In our example, 7/10 means we have 7 parts out of a total of 10 parts.
-
Decimals: Decimals represent a part of a whole using a base-10 system. The digits to the right of the decimal point represent tenths, hundredths, thousandths, and so on. Decimals are particularly useful for calculations involving multiplication and division.
-
Percentages: Percentages represent a part of a whole as a fraction of 100. The percentage symbol (%) signifies "per hundred." Percentages are commonly used to express proportions, rates, and changes.
Converting Fractions to Percentages: A Step-by-Step Guide
The process of converting a fraction to a percentage involves two primary steps:
-
Convert the fraction to a decimal: To do this, divide the numerator by the denominator. In our example, 7/10:
7 ÷ 10 = 0.7
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol (%).
0.7 × 100 = 70%
Therefore, 7/10 is equal to 70%.
Real-World Applications: Where Percentages Matter
Understanding percentage calculations is essential in numerous everyday scenarios:
-
Discounts and Sales: Stores often advertise discounts as percentages. For example, a 20% discount on a $100 item means you save $20 (20% of $100).
-
Taxes: Sales taxes and income taxes are expressed as percentages. Calculating the tax amount requires understanding how to find a percentage of a number.
-
Interest Rates: Interest rates on loans and savings accounts are expressed as percentages. Knowing how to calculate interest is crucial for financial planning.
-
Statistics and Data Analysis: Percentages are frequently used to represent proportions in data sets, making it easier to understand trends and patterns. For example, understanding survey results often involves interpreting percentages.
-
Grade Calculations: In many educational systems, grades are expressed as percentages, representing the proportion of correct answers or overall performance.
-
Tip Calculations: Calculating a tip at a restaurant often involves finding a percentage of the bill amount.
-
Ingredient Proportions: Recipes sometimes express ingredient quantities as percentages of the total amount.
Beyond the Basics: Advanced Percentage Calculations
While converting simple fractions like 7/10 to a percentage is straightforward, other calculations might require more involved steps. Let's explore some common scenarios:
-
Finding a Percentage of a Number: To find X% of Y, you multiply Y by (X/100). For example, to find 15% of 200, you calculate 200 * (15/100) = 30.
-
Finding the Percentage Increase or Decrease: This involves calculating the difference between two numbers and then expressing that difference as a percentage of the original number. For example, if a price increases from $50 to $60, the percentage increase is [(60-50)/50] * 100 = 20%.
-
Calculating Percentages with Fractions and Decimals: Problems might involve converting between fractions, decimals, and percentages within the same calculation. Mastering these conversions is crucial for solving complex percentage problems.
Practical Examples: Applying Percentage Calculations
Let's illustrate these concepts with a few practical examples:
Example 1: Discount Calculation
A store offers a 25% discount on a shirt that originally costs $40. What is the final price after the discount?
-
Calculate the discount amount: 25% of $40 = 0.25 * $40 = $10
-
Subtract the discount from the original price: $40 - $10 = $30
Therefore, the final price of the shirt is $30.
Example 2: Tax Calculation
You buy a book for $20, and the sales tax is 8%. What is the total cost including tax?
-
Calculate the tax amount: 8% of $20 = 0.08 * $20 = $1.60
-
Add the tax to the original price: $20 + $1.60 = $21.60
The total cost of the book, including tax, is $21.60.
Example 3: Percentage Change
The number of students enrolled in a class increased from 20 to 25. What is the percentage increase?
-
Calculate the difference: 25 - 20 = 5
-
Divide the difference by the original number: 5/20 = 0.25
-
Multiply by 100 to get the percentage: 0.25 * 100 = 25%
The number of students increased by 25%.
Troubleshooting Common Mistakes
Common mistakes in percentage calculations include:
-
Incorrect order of operations: Remember to follow the order of operations (PEMDAS/BODMAS) when performing calculations involving multiple steps.
-
Confusion between percentage and decimal: Always ensure you're working with the correct decimal representation of the percentage. For example, 25% is equivalent to 0.25, not 25.
-
Rounding errors: Be mindful of rounding errors, especially when working with multiple decimal places.
Conclusion: Mastering Percentages for a More Numerate Life
Understanding percentages is a fundamental skill with far-reaching applications. From managing personal finances to interpreting data, proficiency in percentage calculations empowers you to make informed decisions and navigate the numerical aspects of daily life with confidence. While the conversion of 7/10 to 70% might seem simple at first glance, the underlying principles illuminate a broader world of mathematical concepts essential for success in various fields. Remember to practice regularly, utilizing diverse examples, to solidify your understanding and develop your computational fluency.
Latest Posts
Latest Posts
-
How To Find The Perimeter Of A Composite Figure
Apr 26, 2025
-
How Big Is 32 Inches In Feet
Apr 26, 2025
-
How To Turn A Mixed Fraction Into A Whole Number
Apr 26, 2025
-
What Is 20 As A Fraction
Apr 26, 2025
-
How Many Miles Is 18000 Feet
Apr 26, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 7 10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.