What Is The Percentage Of 0.75
Kalali
Mar 30, 2025 · 5 min read
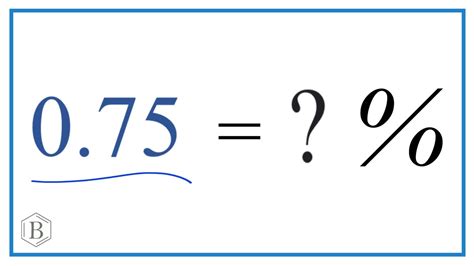
Table of Contents
What is the Percentage of 0.75? A Comprehensive Guide
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts in a store to comprehending financial reports. This comprehensive guide delves into the question: "What is the percentage of 0.75?" and explores the broader concept of decimal-to-percentage conversion. We'll not only answer the direct question but also provide you with the tools and knowledge to tackle similar conversions with ease.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Percentages are widely used because they offer a standardized way to compare proportions and fractions. For instance, saying "75% of students passed the exam" is clearer and more immediately understandable than saying "¾ of students passed."
Converting Decimals to Percentages
The process of converting a decimal to a percentage is straightforward and involves multiplying the decimal by 100 and adding the "%" symbol. This is because a percentage is essentially a decimal expressed as a fraction of 100.
The Formula:
Decimal × 100 = Percentage
Calculating the Percentage of 0.75
Now, let's address the core question: What is the percentage of 0.75?
Applying the formula:
0.75 × 100 = 75
Therefore, 0.75 is equal to 75%.
Practical Applications of Percentage Conversions
Understanding decimal-to-percentage conversions is crucial in numerous real-world scenarios:
1. Financial Calculations:
- Interest rates: Banks and financial institutions express interest rates as percentages (e.g., a 5% interest rate on a savings account). Understanding percentage calculations is essential for comparing different financial products and making informed decisions.
- Discounts and Sales: Stores often advertise discounts as percentages (e.g., a 20% discount on all items). Converting the percentage to a decimal allows you to easily calculate the actual price reduction.
- Tax calculations: Sales tax, income tax, and other taxes are often expressed as percentages of the total amount. Knowing how to calculate percentages is vital for accurate tax computations.
- Investment returns: Investment returns are typically expressed as percentages. Calculating percentage changes in investment values helps you track your portfolio's performance.
2. Academic and Statistical Analysis:
- Grade calculations: Grades in many educational systems are expressed as percentages (e.g., a grade of 85%). Understanding percentage conversions is crucial for calculating final grades and evaluating academic performance.
- Data representation: Percentages are frequently used to represent data in charts, graphs, and reports to make complex information easier to understand. For example, a pie chart may show the percentage of respondents who chose different options in a survey.
- Statistical analysis: Percentages are fundamental in statistical analysis for expressing probabilities, proportions, and frequencies.
3. Everyday Life:
- Tip calculations: When dining out, you might calculate a tip as a percentage of the total bill (e.g., a 15% tip).
- Recipe adjustments: Scaling recipes up or down often involves adjusting ingredient amounts by a percentage.
- Comparing prices: Percentages help in comparing the prices of different products, particularly when considering unit prices or discounts.
Beyond 0.75: Mastering Percentage Conversions
While we've specifically addressed 0.75, let's generalize the process to handle various decimal-to-percentage conversions:
Example 1: Converting 0.25 to a percentage
0.25 × 100 = 25%
Example 2: Converting 0.6 to a percentage
0.6 × 100 = 60%
Example 3: Converting 1.25 to a percentage
1.25 × 100 = 125% (Note: Percentages can exceed 100%)
Example 4: Converting 0.05 to a percentage
0.05 × 100 = 5%
Converting Percentages to Decimals
The reverse process—converting a percentage to a decimal—is equally important. To do this, divide the percentage by 100.
The Formula:
Percentage ÷ 100 = Decimal
Example 1: Converting 75% to a decimal
75 ÷ 100 = 0.75
Example 2: Converting 20% to a decimal
20 ÷ 100 = 0.20
Example 3: Converting 150% to a decimal
150 ÷ 100 = 1.50
Advanced Percentage Calculations
Beyond basic conversions, many more complex percentage calculations are frequently encountered:
- Calculating a percentage of a number: This involves multiplying the number by the percentage (expressed as a decimal). For example, 20% of 50 is 50 × 0.20 = 10.
- Finding the percentage increase or decrease: This involves calculating the difference between two numbers, dividing that difference by the original number, and then multiplying by 100 to express the result as a percentage.
- Calculating percentage change over time: This is essential for tracking trends and analyzing growth or decline in various quantities.
- Working with compound percentages: This involves calculating percentages of percentages, which is commonly seen in financial calculations involving compound interest.
Tools and Resources for Percentage Calculations
While manual calculations are straightforward, various online calculators and software tools can simplify complex percentage calculations. Many spreadsheet programs (like Microsoft Excel or Google Sheets) also have built-in functions for handling percentages.
Conclusion: Mastering Percentages for Success
Understanding percentages is an indispensable skill in both academic and professional settings, and in daily life. This guide has provided a comprehensive overview of percentage calculations, specifically addressing the question, "What is the percentage of 0.75?" By mastering these fundamental concepts and practicing the techniques outlined, you'll be well-equipped to handle a wide range of percentage-related problems with confidence and efficiency. Remember to practice regularly, using diverse examples to solidify your understanding and build fluency in these essential mathematical skills. The ability to confidently handle percentages will significantly enhance your problem-solving skills and provide you with a valuable tool for navigating various aspects of life.
Latest Posts
Latest Posts
-
How Many Oz In 2 2 Lbs
Apr 01, 2025
-
What Is 30 Off Of 30
Apr 01, 2025
-
1 2 3 Cup Equals How Many Ounces
Apr 01, 2025
-
Write 94 As A Fraction In Simplest Form
Apr 01, 2025
-
How Long Is 60 Inches In Cm
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 0.75 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
