What Is The Percentage Of 13/15
Kalali
Apr 16, 2025 · 5 min read
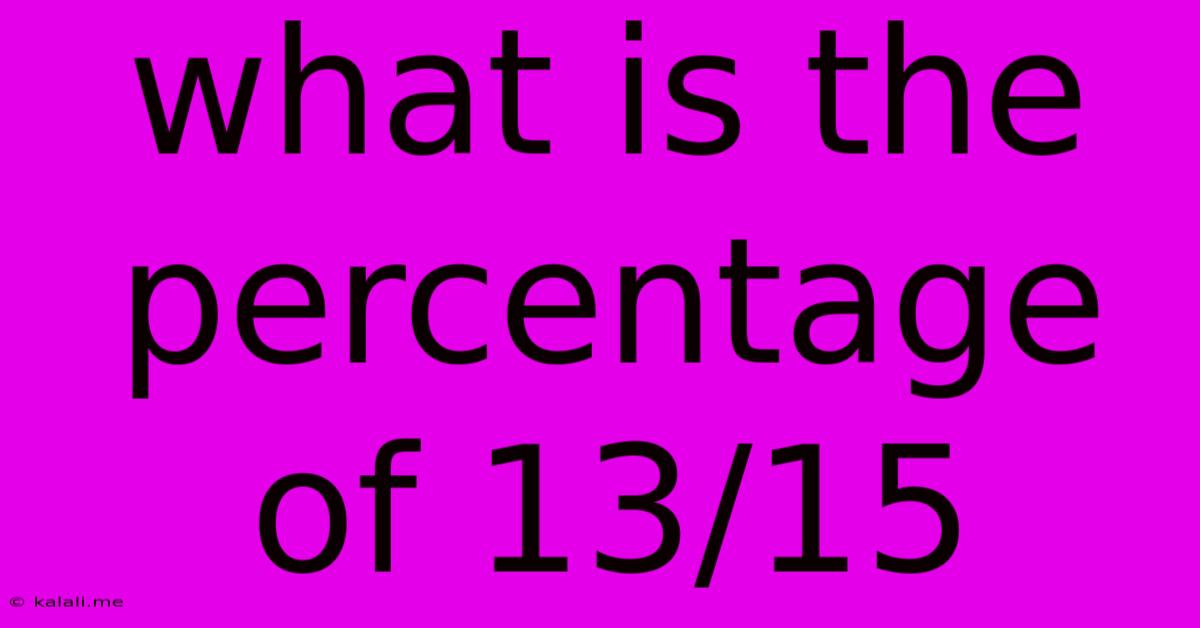
Table of Contents
What is the Percentage of 13/15? A Deep Dive into Fractions, Decimals, and Percentages
This article will explore the simple yet crucial task of converting the fraction 13/15 into a percentage. We’ll not only provide the answer but delve into the underlying mathematical principles, exploring different methods of calculation and highlighting practical applications. Understanding this conversion is fundamental to various fields, from everyday budgeting to advanced statistical analysis. This comprehensive guide will equip you with the knowledge to confidently tackle similar fraction-to-percentage conversions.
Understanding the Basics: Fractions, Decimals, and Percentages
Before we tackle the specific conversion of 13/15, let's refresh our understanding of the three core concepts: fractions, decimals, and percentages.
-
Fractions: A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). In the fraction 13/15, 13 is the numerator, and 15 is the denominator. This means we have 13 parts out of a possible 15.
-
Decimals: Decimals are another way to represent parts of a whole. They use a base-ten system, with the decimal point separating the whole number from the fractional part. For instance, 0.5 represents one-half, or 5/10.
-
Percentages: Percentages represent a fraction or decimal as a portion of 100. The symbol "%" signifies "per hundred." For example, 50% means 50 out of 100, which is equivalent to 0.5 or 1/2.
Method 1: Direct Conversion using Division
The most straightforward method to convert a fraction to a percentage is through division. We divide the numerator by the denominator and then multiply the result by 100.
-
Divide the numerator by the denominator: 13 ÷ 15 ≈ 0.8667
-
Multiply the result by 100: 0.8667 x 100 = 86.67
Therefore, 13/15 is approximately 86.67%. Note that we use "approximately" because the decimal result is recurring (0.86666...). For practical purposes, rounding to two decimal places is usually sufficient.
Method 2: Finding an Equivalent Fraction with a Denominator of 100
While the direct division method is efficient, we can also convert the fraction to an equivalent fraction with a denominator of 100. This method offers a clearer visualization of the percentage. However, this method isn't always practical, especially with fractions that don't easily simplify to a denominator of 100.
Unfortunately, 13/15 doesn't easily simplify to a denominator of 100. To achieve this, we would need to find a number that, when multiplied by 15, results in 100. Since this isn't a whole number, this method is less efficient for this specific fraction. We can, however, demonstrate the principle using a simpler example: Converting 1/4 to a percentage.
-
Find the multiplier: To get a denominator of 100, we multiply 4 by 25 (100/4 = 25).
-
Multiply both the numerator and denominator: (1 x 25) / (4 x 25) = 25/100
-
Express as a percentage: 25/100 = 25%
This method showcases the equivalence between fractions and percentages. The proportion remains the same; only the denominator changes for easier visualization.
Method 3: Using a Calculator
Modern calculators are designed to simplify these conversions. Most calculators have a percentage function (%) or can directly handle fraction-to-decimal conversions. Simply input 13/15 and press the percentage button or convert to decimal and multiply by 100. This method provides a quick and accurate result, particularly useful when dealing with more complex fractions.
Understanding the Significance of the Result: 86.67%
The result, 86.67%, signifies that 13 out of 15 represents 86.67 parts out of every 100 parts. This percentage can be applied in various contexts:
-
Test Scores: If you answered 13 out of 15 questions correctly on a test, your score would be 86.67%.
-
Sales Targets: If you aimed to sell 15 units and sold 13, you achieved 86.67% of your target.
-
Project Completion: If a project involved 15 tasks, and 13 are completed, the project is 86.67% finished.
-
Statistical Analysis: In statistical analysis, percentages are frequently used to represent proportions within a dataset. Understanding this conversion is key to interpreting data effectively.
Rounding and Accuracy:
The significance of rounding depends on the context. For informal calculations or estimations, rounding to the nearest whole number (87%) might suffice. However, for precise financial calculations or scientific applications, retaining more decimal places is essential to maintain accuracy.
Practical Applications of Fraction-to-Percentage Conversions
The ability to convert fractions to percentages is a valuable skill applicable across various fields:
-
Finance: Calculating interest rates, discounts, and profit margins often involves converting fractions to percentages.
-
Statistics: Representing data using percentages facilitates easier interpretation and comparison.
-
Business: Tracking sales performance, market share, and customer satisfaction frequently requires converting fractions to percentages.
-
Science: In experimental settings, percentages are often used to express the proportion of successful trials or the concentration of a substance.
-
Everyday Life: Calculating tips, discounts, and understanding proportions in recipes all involve working with percentages.
Beyond the Basics: Working with More Complex Fractions
While 13/15 is a relatively straightforward fraction, the principles outlined above apply to more complex fractions. The key is to maintain accuracy during the division step and to understand the significance of rounding based on the context.
Conclusion: Mastering Fraction-to-Percentage Conversions
Converting fractions to percentages is a fundamental mathematical skill with wide-ranging applications. Understanding the different methods—direct division, finding equivalent fractions, and utilizing calculators—empowers you to tackle these conversions confidently and accurately. Remember to consider the level of precision required based on the context, and always double-check your calculations to ensure accuracy. This comprehensive guide has equipped you with the tools to effectively work with fractions, decimals, and percentages, making you more adept at tackling various mathematical and real-world problems. The ability to translate fractions into percentages is not just about solving a mathematical problem; it's about enhancing your understanding of proportions and applying that understanding to numerous practical scenarios.
Latest Posts
Latest Posts
-
What Is The Diameter Of A Quarter
Jul 02, 2025
-
Spoon Gets Hot In A Bowl Of Soup
Jul 02, 2025
-
How Many Cups In A 16 Oz Sour Cream
Jul 02, 2025
-
How Many 1 4 Teaspoons Are In 1 Teaspoon
Jul 02, 2025
-
How Many Tbsp In A Dry Ounce
Jul 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 13/15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.