What Is The Percentage Of 28 Out Of 40
Kalali
Mar 07, 2025 · 5 min read
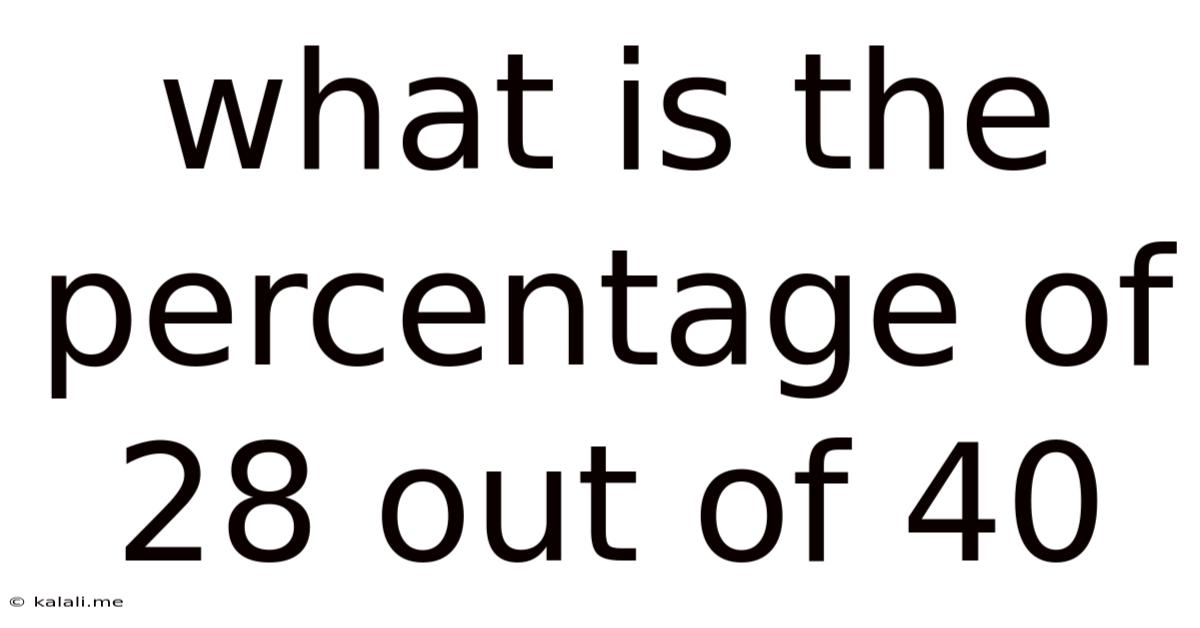
Table of Contents
What is the Percentage of 28 out of 40? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with applications across numerous fields, from academic assignments and everyday budgeting to complex financial analyses and scientific research. Understanding how to determine the percentage one number represents of another is crucial for interpreting data, making informed decisions, and effectively communicating quantitative information. This comprehensive guide will not only answer the question, "What is the percentage of 28 out of 40?" but will also delve into the underlying principles of percentage calculations, offering various methods and examples to solidify your understanding.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Therefore, 25%, for instance, signifies 25 out of 100, which can also be expressed as the fraction 25/100 or the decimal 0.25. Percentages provide a standardized way to compare proportions and make data more readily understandable.
Calculating the Percentage: 28 out of 40
Let's address the central question: What percentage does 28 represent out of 40? There are several ways to calculate this:
Method 1: Using the Fraction Method
This is the most straightforward approach. We express the given numbers as a fraction and then convert that fraction into a percentage.
-
Form a fraction: The fraction representing 28 out of 40 is written as 28/40.
-
Simplify the fraction (optional): We can simplify this fraction by finding the greatest common divisor (GCD) of 28 and 40. The GCD of 28 and 40 is 4. Dividing both the numerator and the denominator by 4, we get 7/10.
-
Convert the fraction to a decimal: To convert the simplified fraction 7/10 to a decimal, we divide the numerator (7) by the denominator (10): 7 ÷ 10 = 0.7
-
Convert the decimal to a percentage: To express the decimal as a percentage, we multiply by 100 and add the percent sign (%): 0.7 × 100 = 70%.
Therefore, 28 out of 40 is 70%.
Method 2: Using the Proportion Method
This method involves setting up a proportion to solve for the unknown percentage.
-
Set up a proportion: We can set up a proportion as follows:
x/100 = 28/40where 'x' represents the unknown percentage. -
Cross-multiply: Cross-multiplying gives us:
40x = 2800 -
Solve for x: Dividing both sides by 40, we get:
x = 70
Therefore, 28 out of 40 is 70%.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply divide 28 by 40 and then multiply the result by 100. This directly gives you the percentage.
28 ÷ 40 × 100 = 70%
Real-World Applications of Percentage Calculations
Understanding percentage calculations is incredibly valuable in numerous real-world scenarios:
-
Academic Performance: Calculating grades, understanding test scores, and assessing overall academic progress often involve percentages. For example, scoring 28 out of 40 on a test translates to a 70% score.
-
Financial Management: Percentages are crucial for managing personal finances. Calculating interest rates, understanding discounts, analyzing investment returns, and tracking expenses all rely heavily on percentage calculations. For instance, a 10% discount on a $100 item means a saving of $10.
-
Business and Economics: Percentages are fundamental in business. Profit margins, sales growth, market share, inflation rates, and unemployment rates are all expressed as percentages. Analyzing these metrics helps businesses make data-driven decisions.
-
Data Analysis and Statistics: Percentages are widely used to represent data in a clear and concise manner. Graphs, charts, and statistical reports frequently utilize percentages to illustrate trends, proportions, and relationships within datasets.
-
Science and Research: In scientific research, percentages are used to represent experimental results, analyze data, and draw conclusions. For example, expressing the effectiveness of a new drug or the success rate of a particular treatment method often involves percentages.
Beyond the Basics: More Complex Percentage Calculations
While calculating the percentage of 28 out of 40 is relatively straightforward, other percentage problems can be more complex. Here are a few examples:
-
Finding a percentage of a number: For example, what is 20% of 50? The calculation is 20/100 * 50 = 10.
-
Finding the original amount: For example, if 30% of a number is 15, what is the original number? Let 'x' be the original number. Then, 0.30x = 15. Solving for x gives x = 15/0.30 = 50.
-
Calculating percentage increase or decrease: For example, if a value increases from 50 to 60, the percentage increase is calculated as [(60-50)/50] * 100% = 20%. Similarly, a decrease from 60 to 50 represents a 16.67% decrease.
Tips for Accurate Percentage Calculations
-
Double-check your work: Always verify your calculations to avoid errors. Using multiple methods can help ensure accuracy.
-
Understand the context: Ensure you understand the context of the problem before attempting to solve it.
-
Use appropriate tools: Utilize calculators or software when necessary for efficiency and accuracy, particularly with complex calculations.
-
Practice regularly: Consistent practice is key to mastering percentage calculations. Solve various problems to build your confidence and understanding.
Conclusion: Mastering Percentage Calculations
The ability to calculate percentages is a fundamental skill with far-reaching applications. Understanding the different methods for calculating percentages and practicing regularly will enable you to confidently tackle various percentage-related problems, whether simple or complex. As demonstrated, determining that 28 out of 40 represents 70% is a straightforward calculation, but the underlying principles extend to a wide range of situations, making it a valuable skill in both personal and professional contexts. By mastering this fundamental skill, you'll be better equipped to interpret data, make informed decisions, and effectively communicate quantitative information.
Latest Posts
Latest Posts
-
How Many Bottles Is 64 Oz Of Water
Jul 02, 2025
-
How Many Ounces In Pound Of Meat
Jul 02, 2025
-
What Is The Diameter Of A Quarter
Jul 02, 2025
-
Spoon Gets Hot In A Bowl Of Soup
Jul 02, 2025
-
How Many Cups In A 16 Oz Sour Cream
Jul 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 28 Out Of 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.