What Is The Percentage Of 36 Out Of 50
Kalali
Apr 27, 2025 · 5 min read
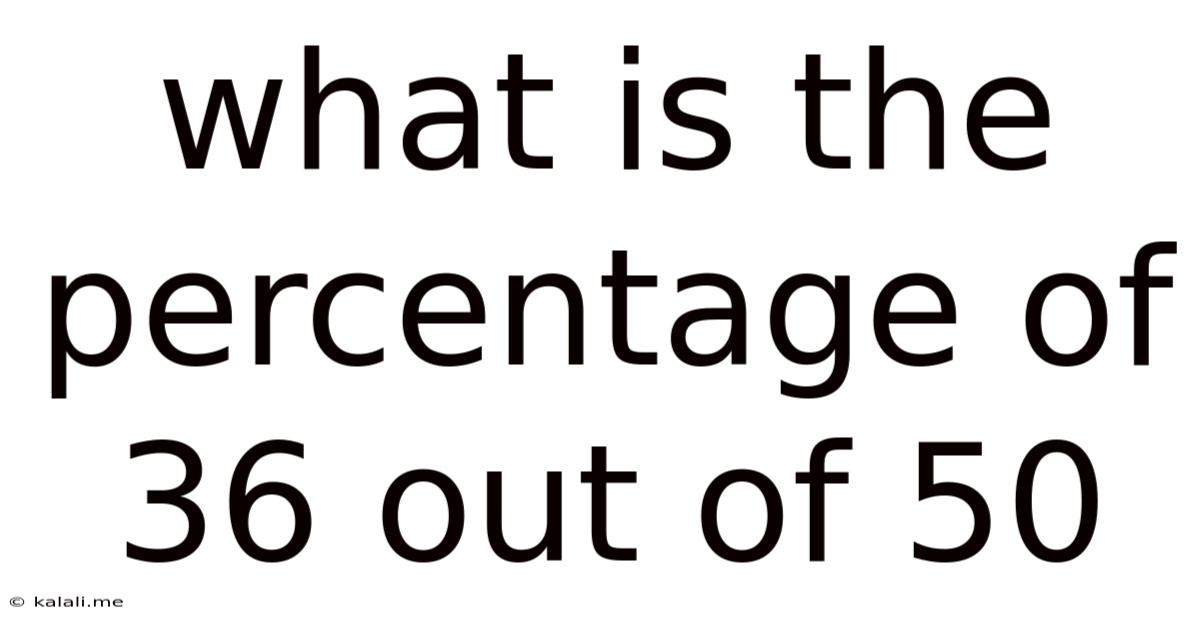
Table of Contents
What is the Percentage of 36 out of 50? A Deep Dive into Percentage Calculations and Applications
Calculating percentages is a fundamental skill with broad applications across various fields, from everyday budgeting to complex statistical analysis. This article will delve into how to calculate the percentage of 36 out of 50, explaining the process step-by-step and exploring the practical uses of percentage calculations. We'll also touch on related concepts and provide examples to solidify your understanding. Understanding percentage calculations is crucial for interpreting data, making informed decisions, and excelling in numerous academic and professional pursuits.
Understanding Percentages: A Foundation
A percentage represents a fraction of 100. It expresses a proportion or ratio as a number out of 100. The symbol "%" is used to denote percentages. For instance, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating the Percentage of 36 out of 50
To calculate the percentage of 36 out of 50, we follow a simple three-step process:
-
Formulate the Fraction: Express the given numbers as a fraction. In this case, it's 36/50.
-
Convert the Fraction to a Decimal: Divide the numerator (36) by the denominator (50). 36 ÷ 50 = 0.72
-
Convert the Decimal to a Percentage: Multiply the decimal by 100 and add the "%" symbol. 0.72 x 100 = 72%.
Therefore, 36 out of 50 is 72%.
Alternative Methods for Percentage Calculation
While the above method is straightforward, alternative approaches can be equally effective, particularly when dealing with more complex scenarios or when using calculators or spreadsheets.
-
Using Proportions: You can set up a proportion to solve for the percentage. Let 'x' represent the percentage:
36/50 = x/100
Cross-multiply: 36 * 100 = 50 * x
Solve for x: 3600 = 50x => x = 3600/50 = 72
Therefore, x = 72%, confirming our previous calculation.
-
Using a Calculator: Most calculators have a percentage function. Simply enter 36 ÷ 50 and then multiply by 100 to obtain the percentage directly.
-
Using Spreadsheets (e.g., Excel, Google Sheets): Spreadsheets provide built-in functions for percentage calculations. For instance, in Excel or Google Sheets, you could use the formula
=36/50*100to directly calculate the percentage.
Practical Applications of Percentage Calculations
Percentage calculations are ubiquitous in daily life and professional settings. Here are some examples:
-
Grade Calculations: If you scored 36 out of 50 on a test, your score is 72%, as we've already calculated. This allows for easy comparison with other scores and assessment of performance.
-
Sales and Discounts: Stores frequently offer discounts expressed as percentages. For example, a 20% discount on a $50 item would reduce the price by $10 (20% of $50). Calculating the final price after a discount requires percentage calculations.
-
Financial Analysis: Percentages are crucial in finance. Interest rates, return on investment (ROI), and profit margins are all expressed as percentages. Understanding these percentages is vital for making sound financial decisions.
-
Data Analysis and Statistics: Percentages are fundamental to statistical analysis. They are used to represent proportions within datasets, to calculate probabilities, and to interpret survey results.
-
Scientific Research: Percentage change is often used in scientific research to compare results between different groups or over time. For example, the percentage increase or decrease in the population of a species over a period of years.
Beyond the Basics: Percentage Increase and Decrease
Often, we need to calculate the percentage increase or decrease between two values. This requires a slightly different approach.
Let's say a value increased from 50 to 72. To calculate the percentage increase:
-
Find the difference: 72 - 50 = 22
-
Divide the difference by the original value: 22 / 50 = 0.44
-
Convert to a percentage: 0.44 * 100 = 44%
Therefore, the value increased by 44%.
Similarly, if a value decreased from 50 to 36, the percentage decrease would be:
-
Find the difference: 50 - 36 = 14
-
Divide the difference by the original value: 14 / 50 = 0.28
-
Convert to a percentage: 0.28 * 100 = 28%
Therefore, the value decreased by 28%.
Percentage Points vs. Percentage Change: It's crucial to differentiate between percentage points and percentage change. A percentage point represents an absolute change in percentage values, whereas percentage change represents the relative change. For example, if an interest rate increases from 5% to 10%, the increase is 5 percentage points, but a 100% percentage change.
Advanced Applications: Compound Interest and Growth Rates
Percentage calculations are fundamental to understanding compound interest and growth rates. Compound interest involves earning interest on both the principal amount and accumulated interest, leading to exponential growth. Understanding percentage growth and decay is crucial when modeling various phenomena like population growth, radioactive decay, or investment returns.
Conclusion: Mastering Percentages for Real-World Success
Understanding percentage calculations is a vital life skill with far-reaching implications. From everyday tasks like budgeting and shopping to complex scenarios in finance, science, and data analysis, the ability to accurately calculate and interpret percentages is invaluable. Mastering these techniques empowers you to make informed decisions, analyze data effectively, and navigate the quantitative aspects of various fields successfully. The simple calculation of 36 out of 50 as 72% serves as a foundational stepping stone to understanding more complex percentage-related problems and their applications in the real world. By applying the methods and concepts outlined in this article, you can confidently tackle percentage calculations in any context.
Latest Posts
Latest Posts
-
What Is The Decimal For 4 25
Apr 27, 2025
-
40 Out Of 60 Is What Percent
Apr 27, 2025
-
How Many Cups Are In 24 Fl Oz
Apr 27, 2025
-
What Is 0 05 As A Percentage
Apr 27, 2025
-
What Is 38 Centimeters In Inches
Apr 27, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 36 Out Of 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.