What Is The Percentage Of 45 Out Of 50
Kalali
Mar 08, 2025 · 5 min read
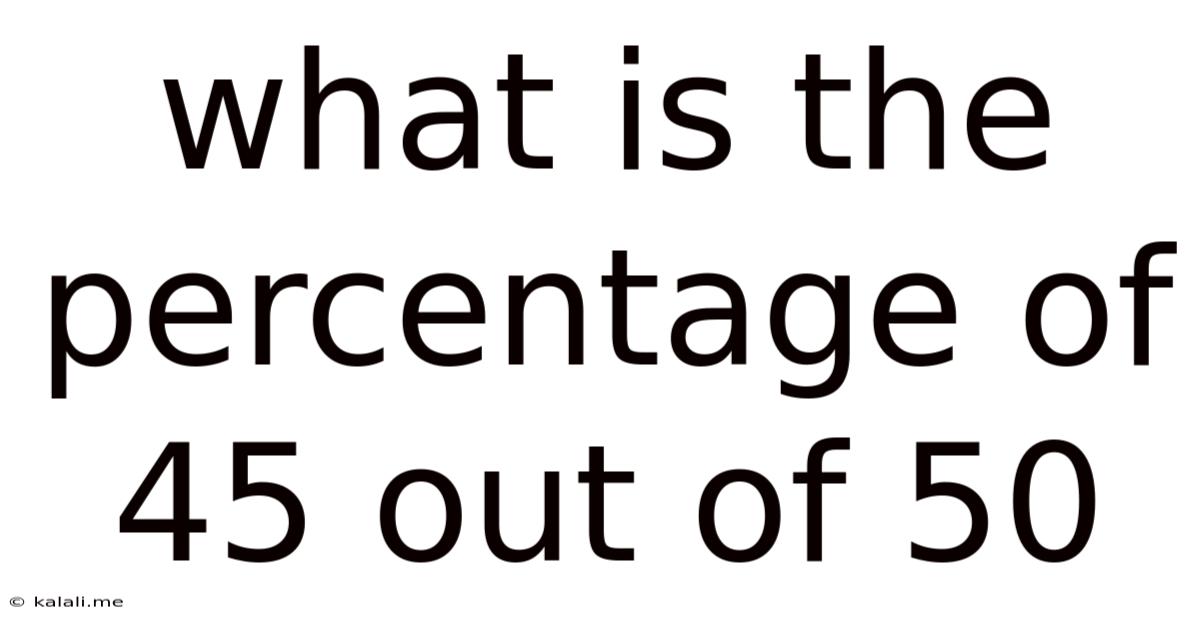
Table of Contents
What is the Percentage of 45 out of 50? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various fields, from everyday budgeting to complex scientific analyses. Understanding how to determine the percentage one number represents of another is crucial for interpreting data, making informed decisions, and communicating effectively. This comprehensive guide will delve into the specifics of calculating the percentage of 45 out of 50, providing a step-by-step explanation, exploring different calculation methods, and showcasing the versatility of percentage calculations in real-world scenarios.
Understanding Percentages: The Basics
Before we dive into the specifics of our example, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Key Concepts:
- Part: This represents the smaller number you are comparing (in our case, 45).
- Whole: This represents the larger number you are comparing the part to (in our case, 50).
- Percentage: This is the result expressed as a number followed by the "%" symbol.
Calculating the Percentage: Method 1 (Fraction to Percentage)
The most straightforward method to calculate the percentage of 45 out of 50 involves converting the ratio to a fraction and then to a percentage.
Step 1: Formulate the Fraction:
Express the problem as a fraction: 45/50. This fraction represents the "part" (45) over the "whole" (50).
Step 2: Convert the Fraction to a Decimal:
Divide the numerator (45) by the denominator (50): 45 ÷ 50 = 0.9
Step 3: Convert the Decimal to a Percentage:
Multiply the decimal by 100 and add the "%" symbol: 0.9 x 100 = 90%.
Therefore, 45 out of 50 is 90%.
Calculating the Percentage: Method 2 (Proportion Method)
This method uses proportions to solve for the unknown percentage. It's particularly helpful when dealing with more complex percentage problems.
Step 1: Set up a Proportion:
We can set up a proportion as follows:
45/50 = x/100
Where 'x' represents the unknown percentage we want to find.
Step 2: Cross-Multiply:
Cross-multiply to solve for 'x':
50x = 4500
Step 3: Solve for x:
Divide both sides by 50:
x = 4500 ÷ 50 = 90
Therefore, x = 90%, confirming our previous result.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is essential across a multitude of real-world scenarios. Here are just a few examples:
1. Academic Performance:
Imagine a student scored 45 out of 50 on a test. Using the methods above, we determine their score is 90%, indicating strong performance. This information is crucial for evaluating progress and identifying areas for improvement.
2. Sales and Discounts:
A store offers a 10% discount on an item originally priced at $50. To calculate the discount amount, we find 10% of $50 (0.10 x $50 = $5). The discounted price would then be $45. Conversely, if an item is priced at $45 after a 10% discount, we can work backward to find the original price.
3. Financial Investments:
Investors frequently monitor the percentage change in their investments. If an investment grew from $50 to $90, the percentage increase is calculated using the same methods. (90-50)/50 * 100 = 80% increase.
4. Statistics and Data Analysis:
Percentages are fundamental to interpreting statistical data. For instance, understanding the percentage of respondents in a survey who favor a particular opinion is crucial for drawing meaningful conclusions. In public health, percentage changes in disease rates are used to track the effectiveness of interventions.
5. Everyday Budgeting:
Percentage calculations aid in budgeting and tracking expenses. Determining the percentage of your income spent on rent, food, or entertainment provides valuable insights into your spending habits and helps make informed financial decisions. For instance, if your monthly income is $2000, and you spend $500 on rent, that represents 25% (500/2000 * 100) of your income.
Beyond the Basics: More Complex Percentage Problems
While our example focuses on a straightforward calculation, percentage problems can become more complex. For example:
- Finding the original value after a percentage increase or decrease: Requires working backward from the final value and the percentage change.
- Calculating compound interest: Involves applying the percentage increase repeatedly over multiple periods.
- Working with percentages of percentages: Requires understanding how to calculate successive percentage changes.
These more advanced scenarios require a strong grasp of fundamental percentage principles, often using algebraic equations to solve for unknown variables.
Tips for Accurate Percentage Calculations:
- Double-check your work: Errors in basic arithmetic can significantly impact your results. Use a calculator to avoid mistakes.
- Understand the context: Ensure you are correctly identifying the "part" and the "whole" in the problem.
- Use consistent units: Make sure all your numbers are in the same units (e.g., dollars, percentage points).
- Practice regularly: The more you practice percentage calculations, the more proficient you'll become.
Conclusion: Mastering Percentage Calculations
Calculating the percentage of 45 out of 50, as shown above, yields a result of 90%. This seemingly simple calculation has far-reaching applications in diverse fields, highlighting the importance of mastering these fundamental mathematical concepts. From academic assessments to financial planning and data analysis, the ability to accurately and efficiently calculate percentages is an indispensable skill. Understanding the various methods and practicing their application will significantly enhance your problem-solving capabilities and your ability to interpret numerical information effectively. The more you engage with percentage calculations, the more intuitive and effortless they will become, empowering you to tackle more complex challenges with confidence. Remember to always double-check your work and apply these techniques thoughtfully in various real-world contexts.
Latest Posts
Latest Posts
-
What Is The Average Iq For A 5 Year Old
Jul 12, 2025
-
How Many 12 Oz Cups In A Gallon
Jul 12, 2025
-
How Much Older Is John The Baptist Than Jesus
Jul 12, 2025
-
How Many Teaspoons In A Pound Of Sugar
Jul 12, 2025
-
How Do You Pass Level 12 On Bloxorz
Jul 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 45 Out Of 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.