What Is The Percentage Of 5/16
Kalali
Apr 12, 2025 · 5 min read
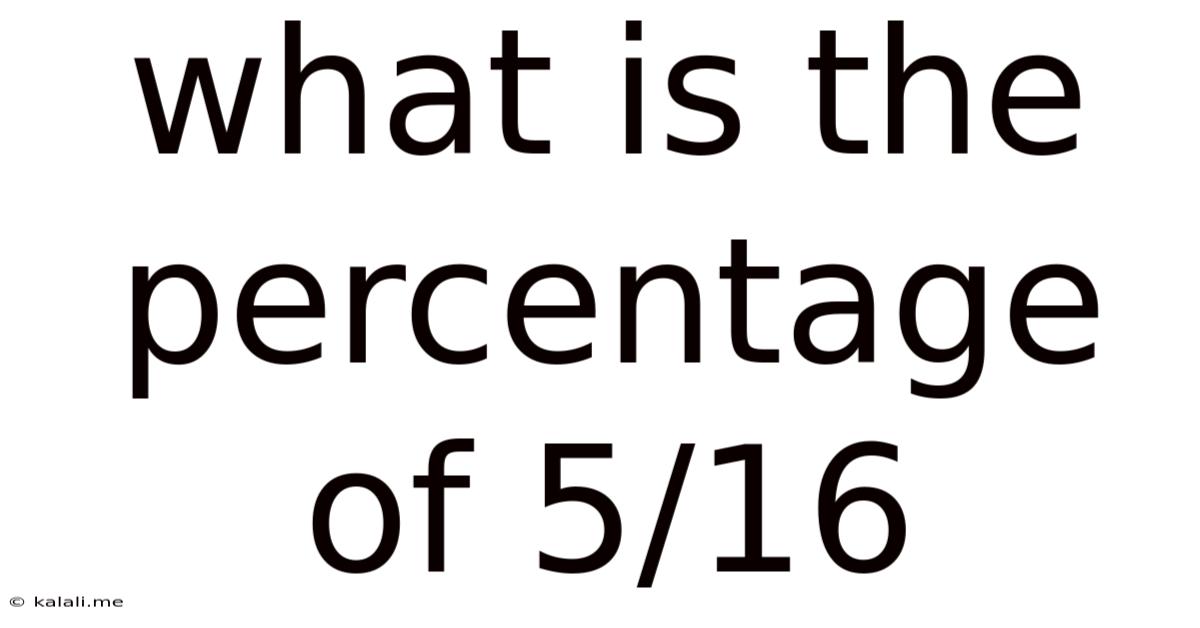
Table of Contents
What is the Percentage of 5/16? A Deep Dive into Fraction to Percentage Conversion
Understanding fractions and their percentage equivalents is a fundamental skill in mathematics, applicable across numerous fields from everyday budgeting to advanced scientific calculations. This article explores the conversion of the fraction 5/16 to a percentage, providing a step-by-step guide, different calculation methods, and real-world applications. We'll also delve into the broader context of fraction-to-percentage conversions, offering you a comprehensive understanding of this essential mathematical concept.
Meta Description: Learn how to convert the fraction 5/16 into a percentage. This comprehensive guide provides multiple methods, real-world examples, and explores the broader concept of fraction-to-percentage conversions.
The question, "What is the percentage of 5/16?" is essentially asking us to express the fraction 5/16 as a percentage. Percentages represent parts of a whole, where the whole is considered 100%. To find the percentage equivalent of a fraction, we need to convert the fraction into a decimal and then multiply by 100%.
Method 1: Direct Conversion using Division
The most straightforward method involves dividing the numerator (5) by the denominator (16) and then multiplying the result by 100%.
-
Divide the numerator by the denominator: 5 ÷ 16 = 0.3125
-
Multiply the result by 100%: 0.3125 × 100% = 31.25%
Therefore, 5/16 is equal to 31.25%.
Method 2: Using Equivalent Fractions
Another approach utilizes the concept of equivalent fractions. We aim to find an equivalent fraction with a denominator of 100. However, this method isn't always practical, especially with fractions that don't easily convert to a denominator of 100. Let's explore a slightly modified version:
We can try to find a fraction that's easily convertible to a percentage. While 100 is the ideal denominator, we can sometimes use simpler conversions. This method is less efficient for 5/16 but illustrative for other fractions.
This method isn't directly applicable to 5/16 because 16 doesn't divide evenly into 100. It's more useful for fractions with denominators that are factors of 100 (like 4, 25, 50, etc.).
Method 3: Using a Calculator
Modern calculators provide a quick and efficient way to convert fractions to percentages. Simply input the fraction as 5/16 and the calculator will directly display the decimal equivalent (0.3125). Multiplying this by 100 gives you the percentage (31.25%). This method is particularly helpful when dealing with complex fractions.
Understanding the Result: 31.25%
The result, 31.25%, signifies that 5/16 represents 31.25 parts out of a total of 100 parts. This percentage is a useful representation for comparisons and calculations. For example, if you have 16 apples and you take 5, you've taken 31.25% of the apples.
Real-World Applications of Fraction to Percentage Conversions
The ability to convert fractions to percentages is essential in many real-world situations:
-
Finance: Calculating interest rates, discounts, profit margins, and tax percentages all involve converting fractions to percentages. For example, a 5/16 discount on a product can be expressed as a 31.25% discount.
-
Science: Expressing experimental results, calculating probabilities, and representing data often necessitates converting fractions to percentages. For instance, if 5 out of 16 experiments were successful, the success rate is 31.25%.
-
Everyday Life: Determining tips in restaurants, calculating sale prices, understanding survey results, and even sharing portions of food or resources involves converting fractions to percentages.
-
Engineering: In engineering, percentages are widely used to express tolerances, efficiency, and other critical parameters. Understanding fraction to percentage conversion allows engineers to readily interpret and communicate these parameters.
-
Statistics: Percentages are vital for presenting statistical data concisely and effectively. Converting fractions allows for clear representation of proportions and probabilities in various analyses.
Expanding the Concept: Different Fraction Types and their Percentage Equivalents
The method described above applies to simple fractions. Let's briefly explore other types of fractions:
-
Improper Fractions: Improper fractions (where the numerator is larger than the denominator) will result in percentages greater than 100%. For example, 16/5 = 320%.
-
Mixed Numbers: Mixed numbers (a whole number and a fraction) require converting the mixed number to an improper fraction before proceeding with the conversion to a percentage. For example, 1 5/16 would first be converted to 21/16, then divided by 16 and multiplied by 100 to get 131.25%.
-
Decimal Fractions: If you start with a decimal fraction, simply multiply the decimal by 100% to get the percentage. For instance, 0.3125 x 100% = 31.25%.
Beyond the Calculation: Importance of Understanding the Concept
While the calculation itself is relatively straightforward, understanding the underlying concept of representing parts of a whole is crucial. This understanding facilitates not only accurate calculations but also effective interpretation and application of percentages in various contexts.
Troubleshooting Common Mistakes
-
Incorrect Order of Operations: Ensure you divide the numerator by the denominator before multiplying by 100%.
-
Decimal Point Errors: Pay close attention to the placement of the decimal point when multiplying by 100%.
-
Rounding Errors: While 31.25% is the exact answer, in some contexts, rounding might be necessary. Understand the implications of rounding and choose appropriate rounding rules based on the context.
Conclusion: Mastering Fraction to Percentage Conversions
The conversion of 5/16 to 31.25% is a simple yet fundamental mathematical operation with wide-ranging applications. Understanding this conversion, along with the broader concepts of fractions and percentages, empowers you to tackle a variety of numerical problems and effectively interpret data presented in different formats. By mastering this skill, you enhance your mathematical proficiency and your ability to navigate various quantitative situations in daily life and professional settings. Remember the importance of understanding the underlying concepts as much as the calculation steps, and always double-check your work to minimize errors.
Latest Posts
Latest Posts
-
Is Hardness A Chemical Or Physical Property
Apr 18, 2025
-
Determine The Order Of The Following Matrix
Apr 18, 2025
-
How To Do Logarithms Without A Calculator
Apr 18, 2025
-
Chromatids Line Up Along The Equator
Apr 18, 2025
-
Corresponding Parts Of Congruent Triangles Are Congruent
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 5/16 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.