What Is The Percentage Of 6/8
Kalali
Mar 09, 2025 · 5 min read
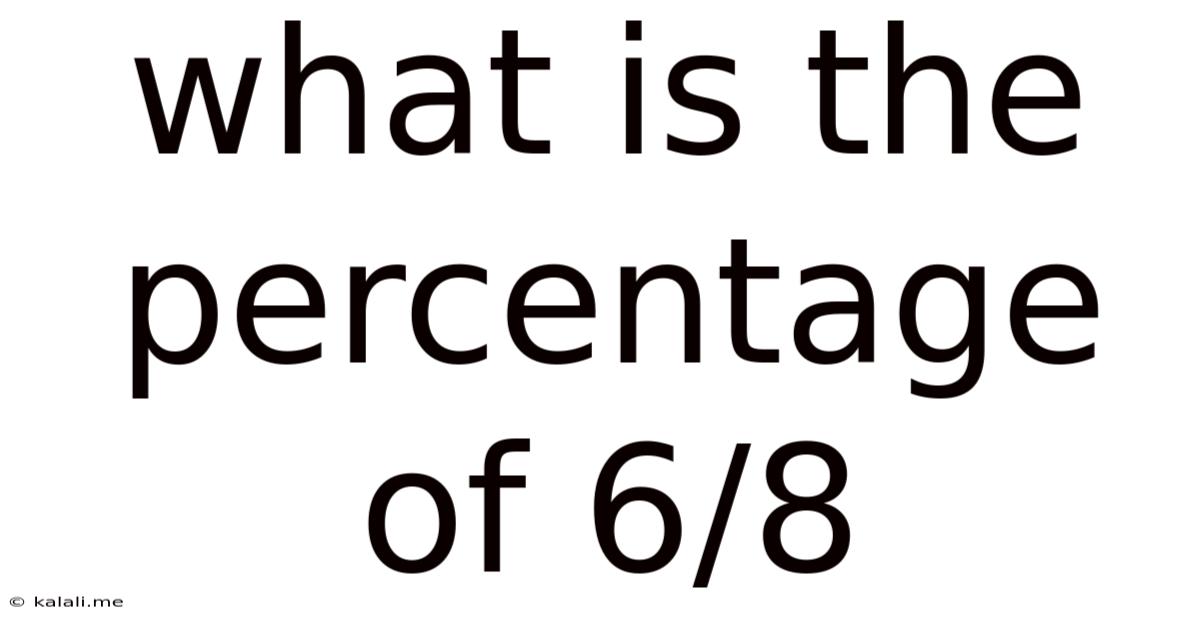
Table of Contents
What is the Percentage of 6/8? A Deep Dive into Fractions, Decimals, and Percentages
The seemingly simple question, "What is the percentage of 6/8?" opens a door to a fundamental understanding of mathematical concepts vital for everyday life and advanced studies. This article will not only answer the question directly but also explore the underlying principles of fractions, decimals, and percentages, providing you with a comprehensive grasp of these interconnected ideas. We'll cover various methods for solving this problem and explore how to apply these techniques to similar calculations.
Understanding Fractions
A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). In the fraction 6/8, 6 is the numerator and 8 is the denominator. This means we are considering 6 parts out of a total of 8 equal parts.
Before we calculate the percentage, it's crucial to simplify the fraction. Simplifying a fraction means reducing it to its lowest terms by dividing both the numerator and the denominator by their greatest common divisor (GCD). The GCD of 6 and 8 is 2. Therefore, we divide both numbers by 2:
6 ÷ 2 = 3 8 ÷ 2 = 4
This simplifies the fraction 6/8 to 3/4. This simplified fraction represents the same proportion as 6/8; it's just expressed more concisely.
Converting Fractions to Decimals
To find the percentage, we first need to convert the fraction into a decimal. This is done by dividing the numerator by the denominator:
3 ÷ 4 = 0.75
Therefore, the decimal equivalent of 3/4 (and 6/8) is 0.75.
Converting Decimals to Percentages
The final step is converting the decimal to a percentage. To do this, we multiply the decimal by 100 and add the percentage symbol (%):
0.75 × 100 = 75%
Therefore, the percentage equivalent of 6/8 is 75%.
Alternative Methods: Working Directly with the Original Fraction
While simplifying the fraction first makes the calculation easier, we can also directly convert 6/8 to a percentage without simplifying:
- Convert to a decimal: 6 ÷ 8 = 0.75
- Convert to a percentage: 0.75 × 100 = 75%
This demonstrates that the simplification step is a matter of convenience, not necessity. However, simplifying fractions is generally recommended as it simplifies subsequent calculations and improves readability.
Real-World Applications: Why Understanding Percentages Matters
Understanding how to convert fractions to percentages has numerous real-world applications:
-
Calculating Discounts: If a store offers a 25% discount on an item, you can easily calculate the savings by converting the percentage to a fraction (25/100 = 1/4) and multiplying it by the original price.
-
Analyzing Financial Statements: Financial reports frequently use percentages to express ratios like profit margins, debt-to-equity ratios, and return on investment (ROI).
-
Understanding Statistics: Percentages are fundamental to interpreting statistical data, such as survey results, election polls, and scientific studies.
-
Evaluating Grades: Academic grades are often expressed as percentages, allowing for easy comparison of performance.
-
Cooking and Baking: Many recipes use fractions and percentages to specify ingredient amounts. Understanding these conversions can ensure accurate measurements.
Expanding on the Concept: Working with More Complex Fractions
The principles discussed above apply to more complex fractions as well. Let's consider the fraction 15/25:
- Simplify the fraction: The GCD of 15 and 25 is 5. Dividing both by 5 gives us 3/5.
- Convert to a decimal: 3 ÷ 5 = 0.6
- Convert to a percentage: 0.6 × 100 = 60%
Therefore, 15/25 is equivalent to 60%.
Handling Improper Fractions and Mixed Numbers
Improper fractions (where the numerator is larger than the denominator) and mixed numbers (a whole number and a fraction) require an extra step before conversion to a percentage:
Improper Fraction Example: 11/4
- Convert to a mixed number: 11 ÷ 4 = 2 with a remainder of 3. This becomes 2 3/4.
- Convert the fraction to a decimal: 3 ÷ 4 = 0.75
- Add the whole number: 2 + 0.75 = 2.75
- Convert to a percentage: 2.75 × 100 = 275%
Mixed Number Example: 3 1/2
- Convert to an improper fraction: (3 × 2) + 1 = 7/2
- Convert to a decimal: 7 ÷ 2 = 3.5
- Convert to a percentage: 3.5 × 100 = 350%
Advanced Applications and Further Learning
Understanding percentages and their relationship to fractions and decimals forms the basis for many advanced mathematical concepts. Further exploration might include:
-
Ratio and Proportion: Percentages are closely related to ratios and proportions, which are used extensively in various fields like engineering, physics, and finance.
-
Compound Interest: Calculating compound interest relies heavily on the understanding of percentages and exponential growth.
-
Statistical Analysis: Advanced statistical methods require a deep understanding of percentages, probabilities, and distributions.
Conclusion: Mastering Percentages – A Lifelong Skill
The seemingly simple question of "What is the percentage of 6/8?" has led us on a journey through the fundamentals of fractions, decimals, and percentages. Mastering these concepts is not just about solving mathematical problems; it's about acquiring a crucial life skill applicable across numerous disciplines. Whether you're shopping for a sale, analyzing financial data, or interpreting research findings, the ability to work confidently with fractions, decimals, and percentages is invaluable. This article has provided a comprehensive guide, equipping you with the knowledge and tools to tackle such calculations with ease and confidence. Remember to practice regularly to solidify your understanding and further refine your skills.
Latest Posts
Latest Posts
-
How Many 16 9 Oz Bottles Make A Half Gallon
Jun 30, 2025
-
How Many Times Does 3 Go Into 24
Jun 30, 2025
-
What Year Was I Born If Im 15
Jun 30, 2025
-
What Is The Easter Bunny Favorite Color
Jun 30, 2025
-
How Much Is 100 Gallons Of Diesel
Jun 30, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 6/8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.