What Percent Is 18 Out Of 30
Kalali
Apr 23, 2025 · 5 min read
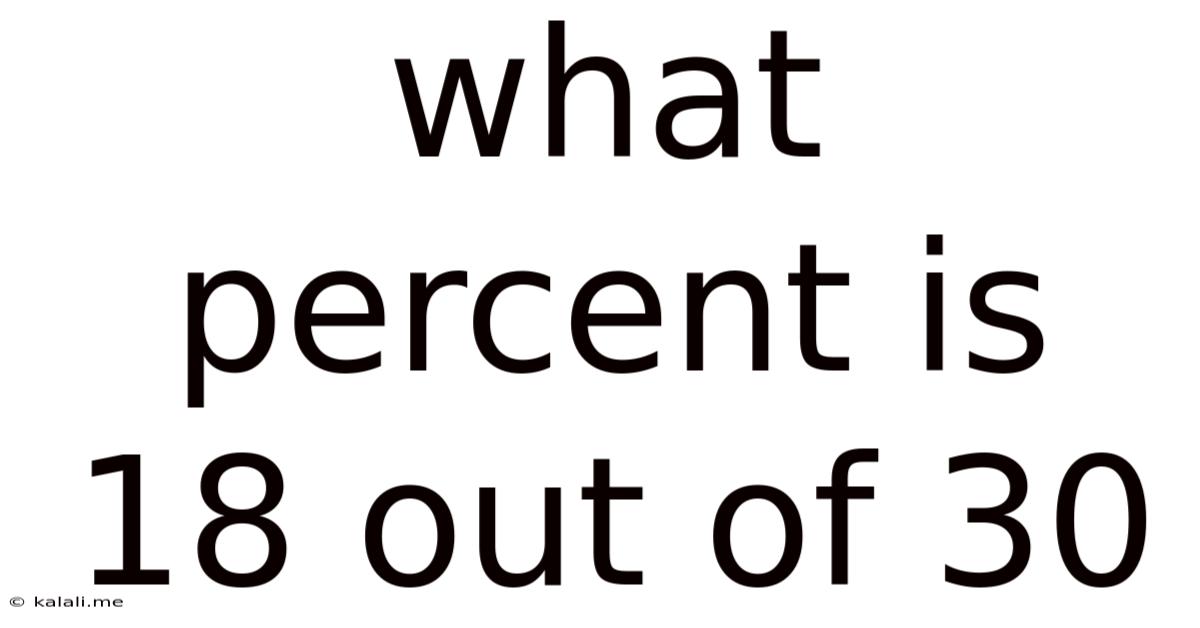
Table of Contents
What Percent is 18 out of 30? A Deep Dive into Percentage Calculations and Applications
This seemingly simple question – "What percent is 18 out of 30?" – opens the door to a much broader understanding of percentages, their applications, and how to confidently tackle similar problems. This article will not only answer the initial question but also explore the underlying concepts, provide multiple methods for solving percentage problems, and illustrate real-world applications of this fundamental mathematical skill.
Meta Description: Learn how to calculate percentages easily! This comprehensive guide explains how to determine what percent 18 is out of 30, covering various calculation methods and real-world applications. Master percentage calculations with our step-by-step examples.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The word "percent" literally means "out of one hundred" ("per cent" – Latin for "out of one hundred"). Therefore, 18 out of 30 represents a portion of the whole, and calculating the percentage involves determining what that portion is as a number out of 100.
Understanding the fundamental relationship between fractions, decimals, and percentages is crucial. They are all different ways of expressing the same numerical value. For example:
- Fraction: 18/30
- Decimal: 0.6
- Percentage: 60%
Method 1: Using the Formula
The most straightforward method to calculate a percentage is using the basic percentage formula:
(Part / Whole) x 100% = Percentage
In our case:
Part = 18 Whole = 30
Therefore:
(18 / 30) x 100% = 60%
Therefore, 18 out of 30 is 60%.
Method 2: Simplifying the Fraction
Before applying the formula, simplifying the fraction can make the calculation easier. We can simplify 18/30 by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 6:
18 ÷ 6 = 3 30 ÷ 6 = 5
This simplifies the fraction to 3/5. Now, we can apply the formula:
(3 / 5) x 100% = 60%
Method 3: Using Decimal Conversion
Another approach is to convert the fraction to a decimal first and then multiply by 100%:
18 / 30 = 0.6
0.6 x 100% = 60%
Method 4: Proportion Method
This method uses proportions to solve for the unknown percentage. We can set up a proportion as follows:
18/30 = x/100
To solve for x (the percentage), we can cross-multiply:
30x = 1800
x = 1800 / 30
x = 60
Therefore, x = 60%, confirming our previous results.
Real-World Applications of Percentage Calculations
Understanding percentages is essential in numerous real-world situations, including:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns. For example, a 60% discount on a $100 item would reduce its price by $60 (60% of $100).
-
Statistics: Analyzing data, interpreting survey results, and understanding probabilities. For example, if 18 out of 30 students passed an exam, the pass rate is 60%.
-
Science: Expressing concentrations, experimental results, and error margins.
-
Shopping: Calculating discounts, sales tax, and comparing prices.
-
Everyday Life: Calculating tips, understanding nutrition labels (percentage of daily value), and interpreting various statistics presented in news and media.
Advanced Percentage Problems: Finding the Whole or the Part
While the initial question focused on finding the percentage, percentage problems can also involve finding the whole or the part when the percentage and either the part or the whole are known. Let's look at examples:
Example 1: Finding the Whole
-
Problem: 60% of a number is 18. What is the number?
-
Solution: Let's represent the unknown number as 'x'. We can set up the equation:
0.60x = 18
x = 18 / 0.60
x = 30
Therefore, the whole number is 30.
Example 2: Finding the Part
-
Problem: What is 40% of 30?
-
Solution: We can use the formula:
(Percentage/100) x Whole = Part
(40/100) x 30 = 12
Therefore, 40% of 30 is 12.
Percentage Increase and Decrease
Another important application of percentages involves calculating percentage increases and decreases.
Example 1: Percentage Increase
-
Problem: A price increased from $20 to $30. What is the percentage increase?
-
Solution:
- Find the difference: $30 - $20 = $10
- Divide the difference by the original price: $10 / $20 = 0.5
- Multiply by 100% to express as a percentage: 0.5 x 100% = 50%
Therefore, the price increased by 50%.
Example 2: Percentage Decrease
-
Problem: A price decreased from $30 to $20. What is the percentage decrease?
-
Solution:
- Find the difference: $30 - $20 = $10
- Divide the difference by the original price: $10 / $30 = 0.3333...
- Multiply by 100% to express as a percentage: 0.3333... x 100% ≈ 33.33%
Therefore, the price decreased by approximately 33.33%.
Common Mistakes to Avoid When Calculating Percentages
-
Incorrect Formula: Ensure you use the correct formula and substitute the values correctly.
-
Decimal Errors: Be careful when working with decimals, especially when multiplying and dividing. Use a calculator if needed to ensure accuracy.
-
Confusing Part and Whole: Always identify the part and the whole clearly before applying the formula.
-
Rounding Errors: When rounding percentages, be consistent and round appropriately based on the context.
Conclusion
Calculating percentages is a fundamental skill with wide-ranging applications. By understanding the basic concepts, mastering different calculation methods, and avoiding common errors, you can confidently tackle percentage problems in various contexts, from everyday finance to complex statistical analysis. Remember to always double-check your work and use a calculator when necessary to maintain accuracy. The ability to quickly and accurately calculate percentages is a valuable asset in many areas of life, both personal and professional. Mastering this skill empowers you to make informed decisions and interpret data effectively.
Latest Posts
Latest Posts
-
How Many Inches Is 30 5 Cm
Apr 23, 2025
-
One To The Power Of Two
Apr 23, 2025
-
2 5 Meters Is How Many Inches
Apr 23, 2025
-
Compare The Nitrogen Carbon And Oxygen Cycles
Apr 23, 2025
-
Each Hemoglobin Molecule Can Transport Two Molecules Of Oxygen
Apr 23, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 18 Out Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.