What Percent Is 19 Of 20
Kalali
Apr 24, 2025 · 5 min read
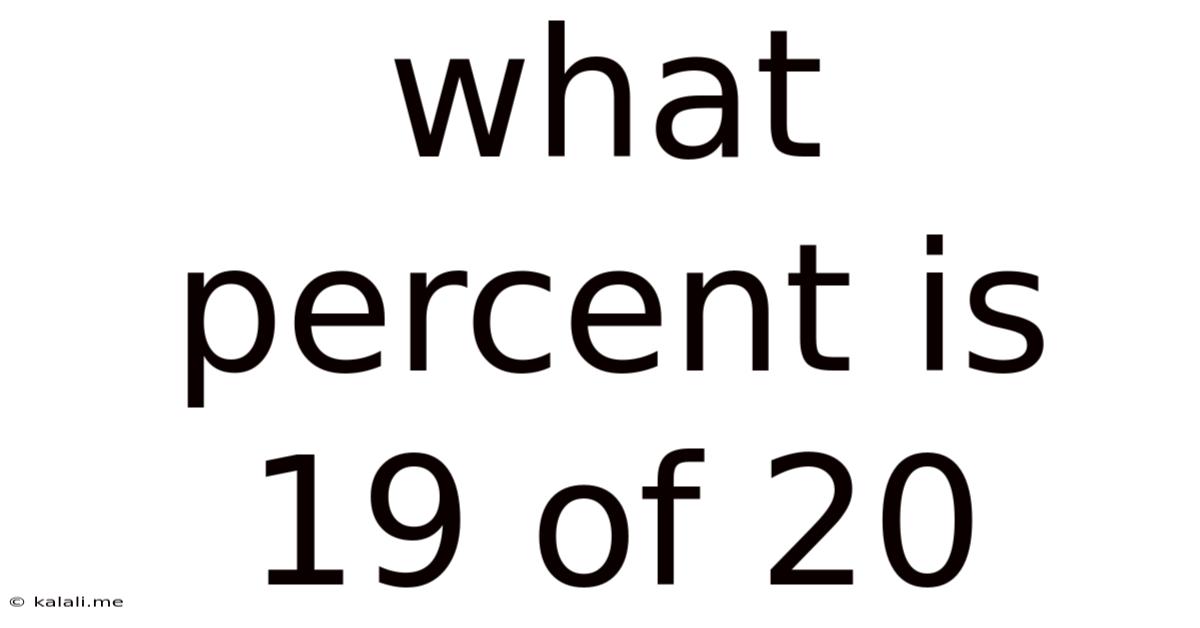
Table of Contents
What Percent is 19 of 20? A Deep Dive into Percentage Calculations and Their Applications
Meta Description: Uncover the answer to "What percent is 19 of 20?" and delve into the world of percentage calculations. This comprehensive guide explains the process, provides real-world examples, and explores advanced percentage applications. Learn how to calculate percentages, understand percentage increase/decrease, and master related concepts.
Finding the percentage one number represents of another is a fundamental skill with widespread applications in various fields, from everyday budgeting to complex financial analysis. This article will not only answer the question, "What percent is 19 of 20?", but also provide a thorough understanding of percentage calculations and their practical uses. We'll explore different methods, explain the underlying concepts, and offer examples to solidify your understanding.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" is used to denote percentages. Essentially, it represents a portion of a whole. For instance, 50% means 50 out of 100, which is equivalent to ½ or 0.5. Understanding this fundamental concept is crucial for tackling percentage problems.
Calculating "What Percent is 19 of 20?"
To determine what percent 19 is of 20, we can use the following formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 19
- Whole: 20
Substituting these values into the formula:
(19 / 20) * 100% = 95%
Therefore, 19 is 95% of 20.
Alternative Methods for Percentage Calculation
While the above method is straightforward, there are alternative approaches that can be equally effective, especially when dealing with more complex scenarios.
Method 1: Using Proportions
This method involves setting up a proportion:
19/20 = x/100
To solve for 'x' (the percentage), cross-multiply:
20x = 1900
x = 1900 / 20
x = 95
Therefore, x = 95%, confirming our previous result.
Method 2: Decimal Conversion
First, divide the part by the whole:
19 / 20 = 0.95
Then, multiply the decimal by 100%:
0.95 * 100% = 95%
This method is particularly useful when working with calculators or spreadsheets.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in everyday life and professional settings. Here are some examples:
-
Sales and Discounts: Retailers frequently advertise discounts as percentages. For example, a 20% discount on a $100 item means a reduction of $20 (20% of $100). Understanding percentage discounts allows consumers to calculate the final price accurately.
-
Taxes and Interest: Calculating sales tax, income tax, and interest rates all involve percentage calculations. Knowing how to compute these percentages is essential for managing personal finances effectively. For example, if the sales tax is 6%, you can easily calculate the tax amount on any purchase.
-
Grade Calculation: Many educational systems use percentages to represent student performance. A grade of 85% indicates that a student correctly answered 85 out of 100 questions or achieved a similar proportion of the total possible score.
-
Financial Analysis: In business and finance, percentages are crucial for analyzing financial statements, calculating profit margins, understanding growth rates, and assessing investment returns. Key performance indicators (KPIs) are frequently expressed as percentages.
-
Data Analysis and Statistics: Percentages are widely used in data analysis and statistics to represent proportions within data sets, such as the percentage of respondents who favored a particular option in a survey.
Percentage Increase and Decrease
Understanding percentage increase and decrease is crucial for analyzing changes over time.
Percentage Increase: This represents the increase in a value expressed as a percentage of the original value. The formula is:
[(New Value - Original Value) / Original Value] * 100%
Percentage Decrease: This represents the decrease in a value expressed as a percentage of the original value. The formula is:
[(Original Value - New Value) / Original Value] * 100%
Example of Percentage Increase:
If the price of a product increases from $50 to $60, the percentage increase is:
[(60 - 50) / 50] * 100% = 20%
Example of Percentage Decrease:
If the price of a product decreases from $100 to $80, the percentage decrease is:
[(100 - 80) / 100] * 100% = 20%
Advanced Percentage Applications
Beyond the basic calculations, percentages are used in more complex scenarios:
-
Compound Interest: This involves earning interest on both the principal amount and accumulated interest. Understanding compound interest is crucial for long-term financial planning and investment strategies.
-
Inflation and Deflation: Inflation (increase in prices) and deflation (decrease in prices) are expressed as percentages and significantly impact economic conditions.
-
Statistical Analysis: Percentages are used extensively in statistical analysis to represent probabilities, confidence intervals, and other important measures.
-
Financial Modeling: Complex financial models rely heavily on percentage calculations to predict future outcomes, assess risk, and make informed investment decisions.
Tips for Mastering Percentage Calculations
-
Practice regularly: The best way to master percentage calculations is through consistent practice. Solve various problems to build confidence and familiarity.
-
Understand the underlying concepts: Ensure you thoroughly grasp the basic principles of percentages, fractions, and ratios.
-
Use different methods: Experiment with different calculation methods to find the approach that works best for you.
-
Utilize calculators and spreadsheets: These tools can significantly simplify complex percentage calculations.
-
Check your work: Always double-check your answers to ensure accuracy.
Conclusion
The question "What percent is 19 of 20?" might seem simple, but it opens the door to a vast world of percentage calculations with significant practical applications. By understanding the underlying principles and mastering different calculation methods, you can confidently tackle various percentage problems in various contexts. From everyday budgeting to advanced financial analysis, a solid understanding of percentages is an invaluable skill. The ability to calculate percentages accurately and efficiently will enhance your problem-solving capabilities and improve your understanding of quantitative information. Remember to practice regularly and utilize available resources to build your proficiency. With consistent effort, you will quickly master this essential skill.
Latest Posts
Latest Posts
-
A Pi Bond Between Two Atoms Of Differing Electronegativity
Apr 25, 2025
-
Cuanto Es 500 Miligramos En Litros
Apr 25, 2025
-
How Many Gallons Of Water In The Ocean
Apr 25, 2025
-
Cuanto Es 1 78 Metros En Pies
Apr 25, 2025
-
How Many Ml In A Third Of A Cup
Apr 25, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 19 Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.