What Percent Is 3 Out Of 15
Kalali
Apr 19, 2025 · 5 min read
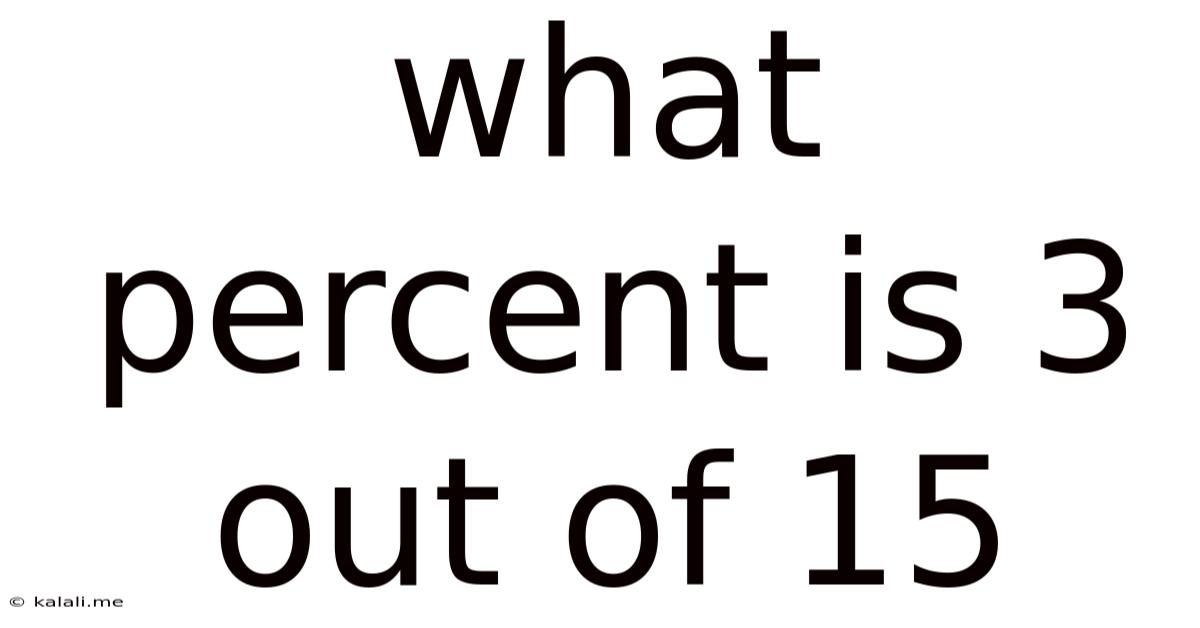
Table of Contents
What Percent is 3 Out of 15? A Deep Dive into Percentage Calculations and Their Applications
What percent is 3 out of 15? The answer might seem simple at first glance, but understanding the underlying principles of percentage calculations opens doors to a vast array of applications in everyday life, from calculating discounts and sales tax to understanding financial reports and statistical data. This article will not only answer the initial question but also explore the various methods for calculating percentages, different scenarios where these calculations are useful, and the importance of percentage literacy in the modern world.
Understanding Percentages: The Basics
A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 1% represents 1/100, 50% represents 50/100 (or 1/2), and 100% represents 100/100 (or 1). Understanding this fundamental concept is crucial for grasping percentage calculations.
Method 1: The Fraction Method – Solving "What percent is 3 out of 15?"
The most straightforward approach to solving "What percent is 3 out of 15?" is to represent the problem as a fraction: 3/15. To convert this fraction into a percentage, we need to find an equivalent fraction with a denominator of 100.
-
Simplify the Fraction: First, simplify the fraction 3/15. Both the numerator (3) and the denominator (15) are divisible by 3. Simplifying gives us 1/5.
-
Convert to a Percentage: To convert the simplified fraction 1/5 to a percentage, we need to find a fraction equivalent to 1/5 with a denominator of 100. We can achieve this by multiplying both the numerator and the denominator by 20: (1 x 20) / (5 x 20) = 20/100.
-
Express as a Percentage: Since 20/100 represents 20 out of 100, this is equivalent to 20%. Therefore, 3 out of 15 is 20%.
Method 2: The Decimal Method
Another common approach involves using decimals. This method is particularly useful when dealing with more complex percentage calculations.
-
Convert the Fraction to a Decimal: Convert the fraction 3/15 to a decimal by dividing the numerator (3) by the denominator (15): 3 ÷ 15 = 0.2
-
Convert the Decimal to a Percentage: To convert a decimal to a percentage, multiply the decimal by 100 and add the percentage symbol: 0.2 x 100 = 20%. Therefore, 3 out of 15 is 20%.
Method 3: Using Proportions
Proportions offer a more formal mathematical approach to solving percentage problems. This method is especially valuable when working with more intricate percentage calculations.
-
Set up a Proportion: We can set up a proportion as follows:
x/100 = 3/15
Where 'x' represents the percentage we're trying to find.
-
Cross-Multiply: Cross-multiplying gives us:
15x = 300
-
Solve for x: Divide both sides by 15:
x = 20
Therefore, 3 out of 15 is 20%.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is a vital life skill with applications across numerous fields:
-
Retail and Sales: Calculating discounts, sales tax, profit margins, and markups. For example, a 20% discount on a $50 item would be a reduction of $10 (20% of $50).
-
Finance: Understanding interest rates, loan repayments, investment returns, and financial statements. Interest rates are usually expressed as percentages.
-
Science and Statistics: Representing data as percentages in graphs, charts, and reports. This makes data easier to understand and compare. For example, survey results are often presented as percentages.
-
Everyday Life: Calculating tips in restaurants, figuring out the percentage of a task completed, determining the nutritional content of food, and much more.
Beyond the Basics: More Complex Percentage Problems
While "What percent is 3 out of 15?" is a relatively simple problem, percentage calculations can become more complex. Here are some examples:
-
Finding the Percentage Increase or Decrease: This involves calculating the percentage change between two values. For example, if a price increases from $100 to $120, the percentage increase is calculated as [(120 - 100) / 100] x 100% = 20%.
-
Calculating Percentage of a Percentage: This involves finding a percentage of a percentage value. For instance, finding 10% of 20% of 1000 would be calculated as (0.1 x 0.2 x 1000) = 20.
-
Working with Multiple Percentages: Situations involving multiple percentages, such as calculating the final price after applying multiple discounts or taxes.
-
Inverse Percentage Problems: Determining the original value when given the final value and a percentage change. For example, if a price has been reduced by 20% to $80, the original price can be calculated as $80 / (1 - 0.2) = $100.
Improving Your Percentage Calculation Skills
Mastering percentage calculations requires consistent practice and understanding. Here are some tips:
-
Practice Regularly: Work through various percentage problems of increasing complexity. Online resources and workbooks offer ample opportunities for practice.
-
Use Different Methods: Familiarize yourself with multiple methods of calculation (fraction, decimal, proportion) to select the most appropriate method depending on the specific problem.
-
Understand the Concepts: Ensure you have a solid grasp of the fundamental concepts of fractions, decimals, and ratios.
-
Check Your Answers: Always verify your calculations to ensure accuracy. Use a calculator when necessary, but strive to understand the underlying mathematical processes.
Conclusion:
While seemingly straightforward, the question "What percent is 3 out of 15?" serves as a springboard to understand the broader significance of percentage calculations. Proficiency in percentage calculations is a critical skill for navigating various aspects of daily life, from personal finance to professional endeavors. By mastering different calculation methods and regularly practicing, you can build a strong foundation for confidently tackling percentage problems of all complexities, empowering you to make informed decisions and interpret data effectively. The answer to the initial question, 20%, is only the beginning of a journey into a world of numerical understanding and application.
Latest Posts
Latest Posts
-
What Is 30 100 As A Percent
Apr 22, 2025
-
How To Find The Range In A Dot Plot
Apr 22, 2025
-
As Altitude Increases What Happens To Air Pressure
Apr 22, 2025
-
How Big Is 36 Inches In Cm
Apr 22, 2025
-
What Is 0 125 In A Fraction
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 3 Out Of 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.