What Percent Of 120 I S6
Kalali
Apr 04, 2025 · 5 min read
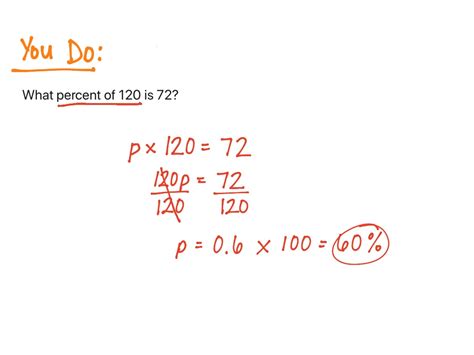
Table of Contents
What Percent of 120 is 6? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many areas of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will not only answer the question "What percent of 120 is 6?" but will also equip you with the knowledge and tools to tackle similar percentage problems with confidence. We'll explore different methods of calculation, delve into the underlying concepts, and provide practical examples to solidify your understanding.
Understanding the Basics of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Key Concepts:
- Part: This is the specific amount we're considering (in our case, 6).
- Whole: This is the total amount we're comparing the part to (in our case, 120).
- Percentage: This is the ratio of the part to the whole, expressed as a number out of 100.
Method 1: Using the Percentage Formula
The most common way to calculate a percentage is using the following formula:
(Part / Whole) x 100% = Percentage
Let's apply this to our problem: "What percent of 120 is 6?"
- Part: 6
- Whole: 120
Substituting these values into the formula:
(6 / 120) x 100% = 0.05 x 100% = 5%
Therefore, 6 is 5% of 120.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can represent our problem as follows:
6 / 120 = x / 100
Where 'x' represents the percentage we're trying to find. To solve for 'x', we can cross-multiply:
6 * 100 = 120 * x
600 = 120x
x = 600 / 120
x = 5
Therefore, 6 is 5% of 120.
Method 3: Using Decimal Conversion
This method involves converting the fraction (Part/Whole) into a decimal and then multiplying by 100%.
- Convert the fraction to a decimal: 6 / 120 = 0.05
- Multiply the decimal by 100%: 0.05 x 100% = 5%
This confirms that 6 is 5% of 120.
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are a few examples:
- Discounts: A store offers a 20% discount on an item priced at $100. The discount amount is (20/100) x $100 = $20. The final price is $100 - $20 = $80.
- Taxes: A sales tax of 8% is added to a purchase of $50. The tax amount is (8/100) x $50 = $4. The total cost is $50 + $4 = $54.
- Grade Calculations: A student scores 45 out of 60 on a test. Their percentage score is (45/60) x 100% = 75%.
- Financial Analysis: Investors use percentages to track returns on investments, growth rates, and other financial metrics.
- Data Analysis: Percentages are widely used in data analysis to represent proportions, trends, and comparisons.
Solving More Complex Percentage Problems
The methods described above can be adapted to solve more complex percentage problems. For example:
- Finding the whole: If 15% of a number is 30, what is the number? We can set up the equation: (15/100) x X = 30. Solving for X gives us X = 200.
- Finding the part: What is 25% of 80? We can calculate this as (25/100) x 80 = 20.
- Finding the percentage increase or decrease: If a value increases from 50 to 60, the percentage increase is calculated as [(60-50)/50] x 100% = 20%.
Tips and Tricks for Mastering Percentage Calculations
- Practice Regularly: The more you practice, the more comfortable you'll become with percentage calculations.
- Use a Calculator: For more complex calculations, a calculator can save you time and effort.
- Understand the Underlying Concepts: A strong understanding of the underlying concepts will help you approach different problems effectively.
- Break Down Complex Problems: Break down complex problems into smaller, more manageable steps.
- Check Your Answers: Always double-check your answers to ensure accuracy.
Advanced Percentage Concepts
Beyond the basic calculations, understanding advanced percentage concepts such as compound interest, percentage points, and weighted averages can significantly enhance your analytical skills and problem-solving abilities. These advanced concepts are frequently encountered in finance, statistics, and various other fields.
- Compound Interest: This refers to interest earned on both the principal amount and accumulated interest. Understanding compound interest is essential for planning long-term investments and savings.
- Percentage Points: This term signifies the arithmetic difference between two percentages. For example, if interest rates increase from 5% to 8%, this is a 3 percentage point increase, not a 60% increase. This distinction is crucial for clear and accurate communication in financial contexts.
- Weighted Averages: When dealing with data sets where different components hold varying levels of significance, weighted averages are used. This method accurately reflects the contribution of each component to the overall average.
Conclusion
Understanding percentages is a crucial skill applicable across various aspects of daily life and professional endeavors. Mastering different calculation methods, from the basic formula to setting up proportions and decimal conversions, allows for efficient and accurate problem-solving. By understanding and practicing these methods, you can confidently tackle a wide range of percentage problems and enhance your analytical and numerical skills. Regular practice and a focus on the underlying concepts will build your expertise, enabling you to confidently navigate the world of percentages and their diverse applications. Remember to always check your work and utilize tools like calculators to ensure accuracy, particularly when dealing with complex calculations. The ability to effectively work with percentages is a valuable asset that can significantly contribute to both personal and professional success.
Latest Posts
Latest Posts
-
How Many Degrees Fahrenheit Is 180 C
Apr 05, 2025
-
What Is A 10 Out Of 16
Apr 05, 2025
-
How Much Is 1 2 A Pint
Apr 05, 2025
-
Does Pcl3 Violate The Octet Rule
Apr 05, 2025
-
What Is 27 Out Of 30
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 120 I S6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
