What Percent Of 30 Is 27
Kalali
Apr 09, 2025 · 5 min read
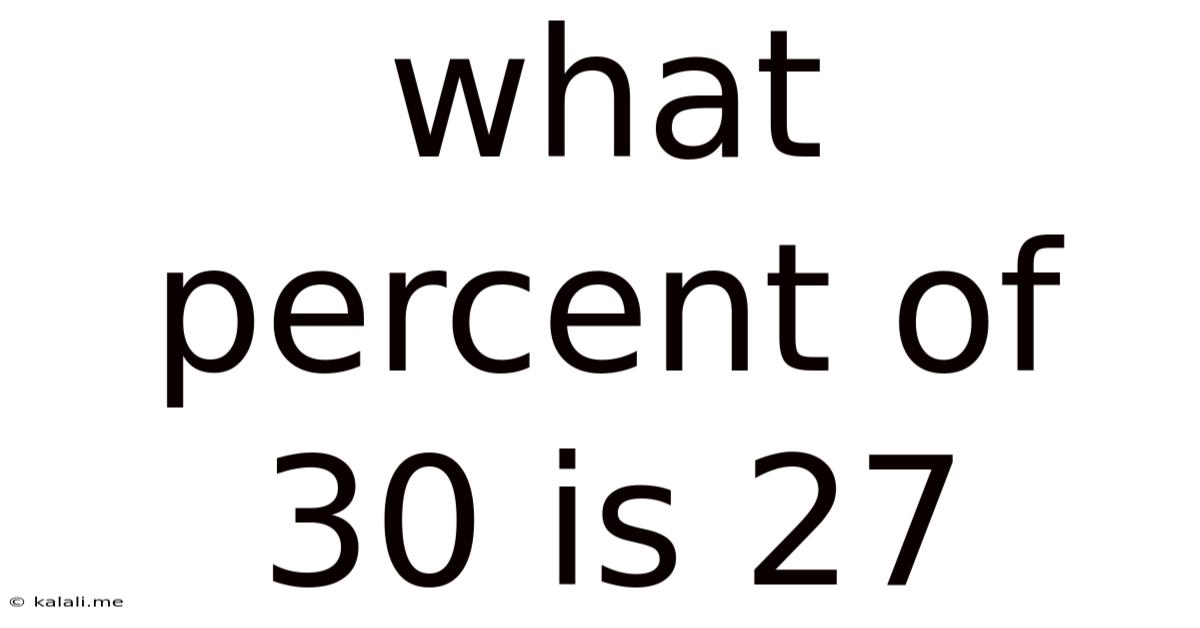
Table of Contents
What Percent of 30 is 27? A Deep Dive into Percentage Calculations and Their Applications
What percent of 30 is 27? This seemingly simple question opens the door to a broader understanding of percentages, their practical applications, and how to solve similar problems efficiently. This article will not only answer this question but also explore the underlying mathematical concepts and demonstrate how these calculations are used in various real-world scenarios. We’ll delve into different methods for solving percentage problems, ensuring you gain a comprehensive grasp of the subject.
Meta Description: Discover how to calculate percentages easily! This in-depth guide explains how to determine what percent of 30 is 27, covering various methods and real-world applications of percentage calculations. Learn to solve similar problems with confidence.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred." The symbol used to represent percentage is %. Therefore, 25% means 25 out of 100, or 25/100, which simplifies to 1/4. Understanding this fundamental concept is crucial for solving any percentage problem.
Percentages are incredibly useful for representing proportions and making comparisons. They're used extensively in finance (interest rates, discounts), statistics (data representation), and everyday life (tips, sales tax).
Method 1: The Proportion Method
This method is a classic approach to solving percentage problems. We set up a proportion, equating two ratios:
- Ratio 1: The part to the whole (27 to 30)
- Ratio 2: The percentage to 100 (x to 100, where x is the unknown percentage)
The proportion looks like this:
27/30 = x/100
To solve for x, we cross-multiply:
30x = 2700
Now, divide both sides by 30:
x = 2700 / 30 = 90
Therefore, 27 is 90% of 30.
Method 2: The Decimal Method
This method involves converting the percentage to a decimal and then performing a simple calculation.
First, we express the problem as an equation:
x * 30 = 27
Where 'x' represents the decimal equivalent of the percentage we're looking for. To find x, we divide both sides by 30:
x = 27 / 30 = 0.9
Now, to convert the decimal 0.9 back into a percentage, we multiply by 100:
0.9 * 100 = 90%
Again, we confirm that 27 is 90% of 30.
Method 3: Using a Calculator
Modern calculators simplify percentage calculations significantly. Most calculators have a percentage button (%). The steps generally involve:
- Divide the part (27) by the whole (30): 27 ÷ 30 = 0.9
- Multiply the result by 100: 0.9 x 100 = 90
- The answer is 90%.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in various aspects of our lives. Here are some examples:
-
Finance: Calculating interest earned on savings accounts, determining loan payments, understanding discounts on products, calculating taxes, and analyzing investment returns all involve percentage calculations. For instance, if a bank offers a 5% annual interest rate on a savings account, you can use percentage calculations to determine the interest earned on your deposit after a year.
-
Retail: Discounts and sales are often expressed as percentages. A "20% off" sale means you'll pay 80% of the original price. Understanding these percentages helps consumers make informed purchasing decisions. Similarly, sales tax is calculated as a percentage of the purchase price.
-
Statistics: Percentages are essential in presenting and interpreting statistical data. For example, survey results are often expressed as percentages to show the proportion of respondents who chose a particular answer. This allows for easy comparison and visualization of data.
-
Science: In scientific research, percentages are used to represent concentrations of solutions, error margins in experiments, and changes in measurements over time.
-
Everyday Life: Tipping in restaurants is typically calculated as a percentage of the bill. Understanding percentages helps you determine the appropriate tip amount. Similarly, calculating the percentage of a task completed can help you track progress towards a goal.
Solving More Complex Percentage Problems
While the example of "What percent of 30 is 27?" is relatively straightforward, percentage problems can become more complex. Let's consider a few variations:
-
Finding the whole: If 20% of a number is 10, what is the number? This requires reversing the calculation. We set up the equation: 0.20 * x = 10. Solving for x, we get x = 10 / 0.20 = 50.
-
Finding the part: What is 15% of 80? This involves a simple multiplication: 0.15 * 80 = 12.
-
Percentage increase/decrease: If a price increases from $50 to $60, what is the percentage increase? First, find the difference: $60 - $50 = $10. Then, divide the difference by the original price and multiply by 100: ($10 / $50) * 100 = 20%.
Understanding these variations allows you to tackle a wider range of percentage-related problems.
Mastering Percentage Calculations: Tips and Tricks
-
Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations.
-
Use different methods: Try solving the same problem using different methods (proportion, decimal, calculator) to reinforce your understanding.
-
Visual aids: Diagrams and charts can help visualize percentage problems and make them easier to understand.
-
Break down complex problems: Divide complex percentage problems into smaller, more manageable steps.
-
Check your work: Always check your answer to ensure it's reasonable and accurate.
Conclusion: The Power of Percentages
Understanding percentages is a fundamental skill with wide-ranging applications. From managing personal finances to interpreting scientific data, mastering percentage calculations empowers you to navigate various aspects of life more effectively. By understanding the different methods and applying the tips and tricks discussed, you can confidently tackle any percentage problem you encounter. The seemingly simple question, "What percent of 30 is 27?" serves as a gateway to a deeper understanding of this essential mathematical concept. Remember to practice regularly and explore the diverse applications of percentages to truly master this vital skill.
Latest Posts
Latest Posts
-
Cuanto Es 40 Fahrenheit En Centigrados
Apr 18, 2025
-
What Is Half A Pint In Cups
Apr 18, 2025
-
What Is The Reciprocal Of 3 4
Apr 18, 2025
-
Cuanto Mide Un Acre En Pies
Apr 18, 2025
-
What Temp Is 180 Celsius In Fahrenheit
Apr 18, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 30 Is 27 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.