What Percent Of 45 Is 9
Kalali
Apr 24, 2025 · 5 min read
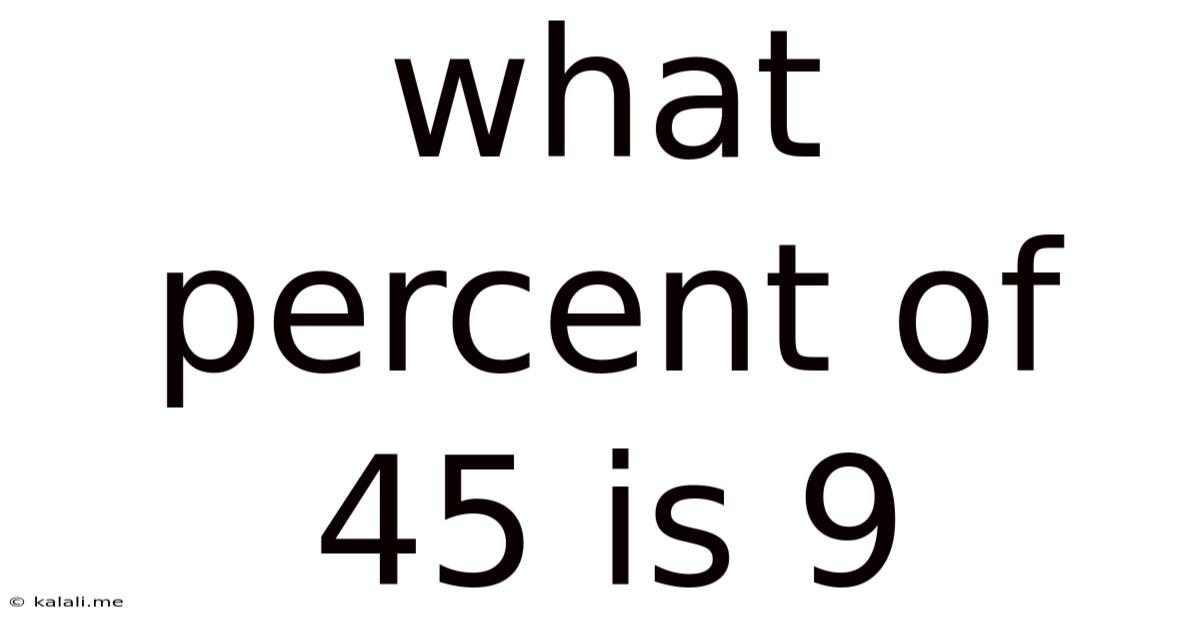
Table of Contents
What Percent of 45 is 9? A Deep Dive into Percentage Calculations
This seemingly simple question – "What percent of 45 is 9?" – opens the door to a broader understanding of percentage calculations, a fundamental skill applicable across various fields, from finance and statistics to everyday life. This article will not only answer the question directly but also explore the different methods for solving percentage problems, delve into the underlying mathematical principles, and provide practical examples to solidify your understanding. We'll also touch upon the importance of percentages in various real-world scenarios.
Understanding Percentages: The Foundation
A percentage is a fraction or ratio expressed as a number out of 100. The word "percent" literally means "out of one hundred" (per centum in Latin). Therefore, 25% means 25 out of 100, which can be written as the fraction 25/100 or the decimal 0.25. Understanding this fundamental concept is crucial for tackling percentage problems effectively.
Method 1: Using the Proportion Method
This method is arguably the most intuitive and widely used for solving percentage problems. It involves setting up a proportion, which is an equation stating that two ratios are equal. We can represent the problem "What percent of 45 is 9?" as follows:
- Part/Whole = Percentage/100
In this case:
- Part: 9 (the number we're interested in)
- Whole: 45 (the total number)
- Percentage: x (the unknown percentage we need to find)
Substituting these values into our proportion equation gives us:
9/45 = x/100
To solve for x, we cross-multiply:
9 * 100 = 45 * x
900 = 45x
Now, divide both sides by 45:
x = 900 / 45
x = 20
Therefore, 9 is 20% of 45.
Method 2: Using the Decimal Method
This method involves converting the percentage to a decimal and then performing a simple multiplication. To find what percentage 9 is of 45, we can set up the equation:
9 = x * 45
To solve for x (the decimal equivalent of the percentage), we divide both sides by 45:
x = 9 / 45
x = 0.2
To convert the decimal 0.2 to a percentage, we multiply by 100:
0.2 * 100 = 20%
Thus, using the decimal method, we again confirm that 9 is 20% of 45.
Method 3: Using the Formula Method
A more direct formula can be derived from the proportion method:
Percentage = (Part / Whole) * 100
Plugging in our values:
Percentage = (9 / 45) * 100
Percentage = 0.2 * 100
Percentage = 20%
This formula provides a concise and efficient way to calculate percentages directly.
Practical Applications of Percentage Calculations
The ability to calculate percentages is incredibly valuable in numerous real-world scenarios. Here are a few examples:
-
Calculating Discounts: Stores frequently offer discounts on items. Understanding percentages allows you to quickly determine the final price after a discount. For instance, a 25% discount on a $100 item would result in a savings of $25 (25% of $100), making the final price $75.
-
Understanding Taxes: Sales tax is a percentage added to the price of goods and services. Knowing how to calculate percentages helps you determine the total cost including tax.
-
Analyzing Financial Statements: Percentages are extensively used in financial analysis to compare data over time or across different entities. For example, calculating the percentage change in revenue or profit year-over-year provides valuable insights into a company's financial performance.
-
Evaluating Grades: Academic grades are often expressed as percentages, representing the proportion of correct answers or the overall performance in a course.
-
Tracking Progress: Percentages are excellent for monitoring progress towards a goal. For instance, if you're saving money for a down payment on a house, calculating the percentage saved relative to the target amount helps you track your progress.
-
Understanding Statistics: Percentages are fundamental in statistical analysis for representing proportions, probabilities, and trends in data.
Advanced Percentage Problems and Concepts
While the problem "What percent of 45 is 9?" is relatively straightforward, percentage calculations can become more complex. Here are a few examples of more advanced concepts:
-
Finding the Whole: Sometimes you know the percentage and the part, but you need to find the whole. For example, if 20% of a number is 9, what is the number? You can solve this using the formula: Whole = Part / (Percentage/100). In this case, Whole = 9 / (20/100) = 45.
-
Finding the Percentage Increase or Decrease: These calculations determine the percentage change between two values. The formula for percentage increase is: [(New Value - Old Value) / Old Value] * 100. The formula for percentage decrease is similar, but the result will be negative.
-
Compound Interest: Compound interest involves earning interest on both the principal amount and accumulated interest. Calculating compound interest requires understanding exponential growth and percentage calculations.
-
Percentage Points vs. Percentages: It's important to distinguish between percentage points and percentages. A change from 10% to 20% is a 10 percentage point increase, but it represents a 100% increase ( (20-10)/10 *100 = 100%). This distinction is crucial for accurate interpretation of data.
Conclusion: Mastering Percentage Calculations
Understanding percentages is a vital life skill with far-reaching applications. This article has explored different methods for solving percentage problems, ranging from simple proportions to more advanced formulas. Mastering these techniques will not only help you solve problems efficiently but will also enhance your ability to analyze data, understand financial concepts, and make informed decisions in various aspects of your life. Remember to practice regularly and apply these concepts to real-world scenarios to solidify your understanding and build confidence in your ability to handle percentage calculations with ease. By understanding the underlying principles and utilizing the different methods outlined, you can confidently tackle any percentage problem, no matter its complexity. The seemingly simple question "What percent of 45 is 9?" thus serves as a gateway to a much wider world of numerical literacy and problem-solving.
Latest Posts
Latest Posts
-
How Much Is 24 Fluid Ounces
Apr 24, 2025
-
How Much Is 10 Degrees Fahrenheit In Celsius
Apr 24, 2025
-
Which Leukocytes Release Histamine During The Inflammatory Response
Apr 24, 2025
-
What Is 50 Degrees Fahrenheit In Centigrade
Apr 24, 2025
-
8 Cups Is How Many Pints
Apr 24, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 45 Is 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.