What Percentage Is 10 Out Of 50
Kalali
Mar 05, 2025 · 6 min read
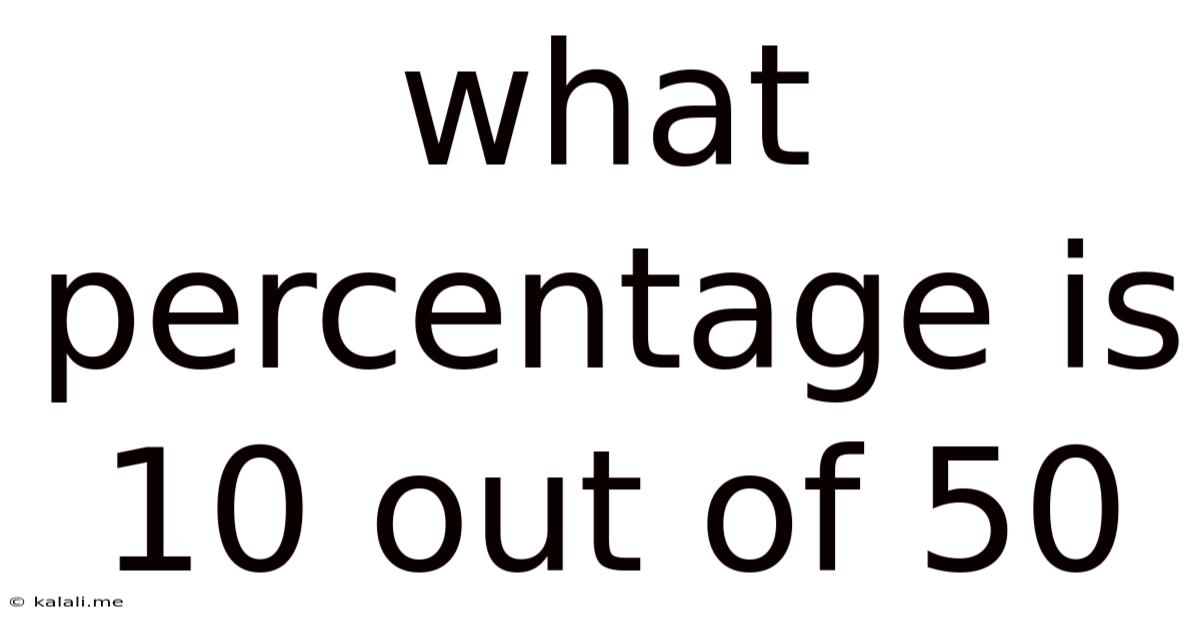
Table of Contents
What Percentage is 10 out of 50? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill in many areas of life, from everyday budgeting and shopping to more complex academic and professional tasks. Understanding how to calculate percentages allows you to analyze data, compare values, and make informed decisions. This comprehensive guide will walk you through calculating what percentage 10 out of 50 represents, explaining the process step-by-step and providing practical examples to solidify your understanding. We'll also explore different methods and delve into the broader application of percentage calculations.
Understanding Percentages
Before diving into the specifics of calculating 10 out of 50 as a percentage, let's establish a firm grasp of the concept of percentages. A percentage is simply a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of 100" (per centum in Latin). Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Method 1: The Fraction Method
This is the most straightforward method for calculating percentages. It involves converting the given numbers into a fraction and then multiplying by 100.
Step 1: Express the numbers as a fraction.
In our case, we want to find what percentage 10 is out of 50. This can be expressed as the fraction 10/50.
Step 2: Simplify the fraction (optional but recommended).
Simplifying the fraction makes the calculation easier. Both 10 and 50 are divisible by 10, so we simplify 10/50 to 1/5.
Step 3: Convert the fraction to a decimal.
To convert the fraction 1/5 to a decimal, divide the numerator (1) by the denominator (5): 1 ÷ 5 = 0.2
Step 4: Multiply the decimal by 100 to get the percentage.
0.2 x 100 = 20%
Therefore, 10 out of 50 is 20%.
Method 2: The Proportion Method
This method uses proportions to solve for the unknown percentage. It's particularly helpful when dealing with more complex percentage problems.
Step 1: Set up a proportion.
We can set up a proportion as follows:
10/50 = x/100
Where 'x' represents the unknown percentage we want to find.
Step 2: Cross-multiply.
Cross-multiplying gives us:
50x = 1000
Step 3: Solve for x.
Divide both sides of the equation by 50:
x = 1000/50 = 20
Therefore, x = 20%, confirming our result from the fraction method.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the calculation significantly.
Step 1: Divide the part by the whole.
Divide 10 by 50: 10 ÷ 50 = 0.2
Step 2: Multiply by 100.
Multiply the result by 100: 0.2 x 100 = 20
Step 3: Add the percentage symbol.
The answer is 20%.
This method is quick and efficient, especially for more complex calculations.
Real-World Applications: Understanding the Significance of 20%
Understanding that 10 out of 50 represents 20% has numerous practical applications. Let's explore a few:
Academic Performance:
Imagine a student scoring 10 out of 50 on a quiz. Knowing this represents 20% allows the student to understand their performance relative to the total possible points. This informs them of areas needing improvement and helps track progress over time.
Business and Finance:
In business, understanding percentages is crucial. For example, if a company sells 10 out of 50 units of a product, knowing this equates to 20% helps analyze sales performance, predict future demand, and make informed decisions about inventory management and marketing strategies.
Data Analysis:
In data analysis, percentages are frequently used to represent proportions within datasets. For example, if a survey of 50 people shows 10 prefer a particular brand, knowing this is 20% allows for comparisons with other brands and helps understand market preferences.
Everyday Life:
Percentages are ubiquitous in everyday life. Discounts, sales tax, tips, and interest rates are all expressed as percentages. Understanding percentage calculations empowers you to make informed decisions in these situations, ensuring you're getting the best deals and managing your finances effectively.
Beyond 10 out of 50: Mastering Percentage Calculations
The principles outlined above can be applied to calculate any percentage. Let's consider some variations:
- What percentage is 25 out of 50? Using the same methods, we find this to be 50%.
- What percentage is 5 out of 50? This equates to 10%.
- What is 30% of 50? To solve this, we multiply 50 by 0.3 (30% expressed as a decimal), which equals 15.
Advanced Percentage Problems and Their Solutions
While the example of 10 out of 50 is relatively simple, percentage problems can become more complex. Here are a few examples and their solutions:
Example 1: Finding the Original Value
A store offers a 20% discount on an item, and the discounted price is $40. What was the original price?
Solution:
Let x be the original price. The discount is 20% of x, which is 0.2x. The discounted price is x - 0.2x = 0.8x. We know 0.8x = $40. Solving for x, we get x = $40 / 0.8 = $50. The original price was $50.
Example 2: Percentage Increase or Decrease
A company's sales increased from 50 units to 60 units. What is the percentage increase?
Solution:
The increase in sales is 60 - 50 = 10 units. To find the percentage increase, divide the increase by the original value and multiply by 100: (10/50) x 100 = 20%. The sales increased by 20%.
Example 3: Calculating Percentage Change Over Multiple Periods:
A stock price increases by 10% one year and then decreases by 10% the next year. Is the final price the same as the original price?
Solution: No. Let's assume the original price is $100. After a 10% increase, the price becomes $110. A 10% decrease from $110 is $11 (10% of $110), resulting in a final price of $99. The final price is lower than the original price due to the way percentage changes are calculated.
Conclusion: Mastering Percentages for Success
Understanding how to calculate percentages is a valuable skill with wide-ranging applications. Whether you're dealing with simple calculations like finding what percentage 10 out of 50 represents (20%) or tackling more complex problems, mastering percentage calculations will empower you to analyze data, make informed decisions, and succeed in various aspects of your life. Remember the different methods discussed – the fraction method, the proportion method, and using a calculator – and choose the one that best suits your needs and the complexity of the problem. With practice, percentage calculations will become second nature, allowing you to confidently navigate the numerical world around you.
Latest Posts
Latest Posts
-
Did Chester Wear A Brace On Gunsmoke
Jul 15, 2025
-
How Many Mikes Hard To Get Drunk
Jul 15, 2025
-
How Many Liters Has In Half Gallon
Jul 15, 2025
-
How Much Is Half Of A Mile
Jul 15, 2025
-
What Letter Is Halfway Through The Alphabet
Jul 15, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 10 Out Of 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.