What Percentage Is 2 Of 12
Kalali
Apr 16, 2025 · 5 min read
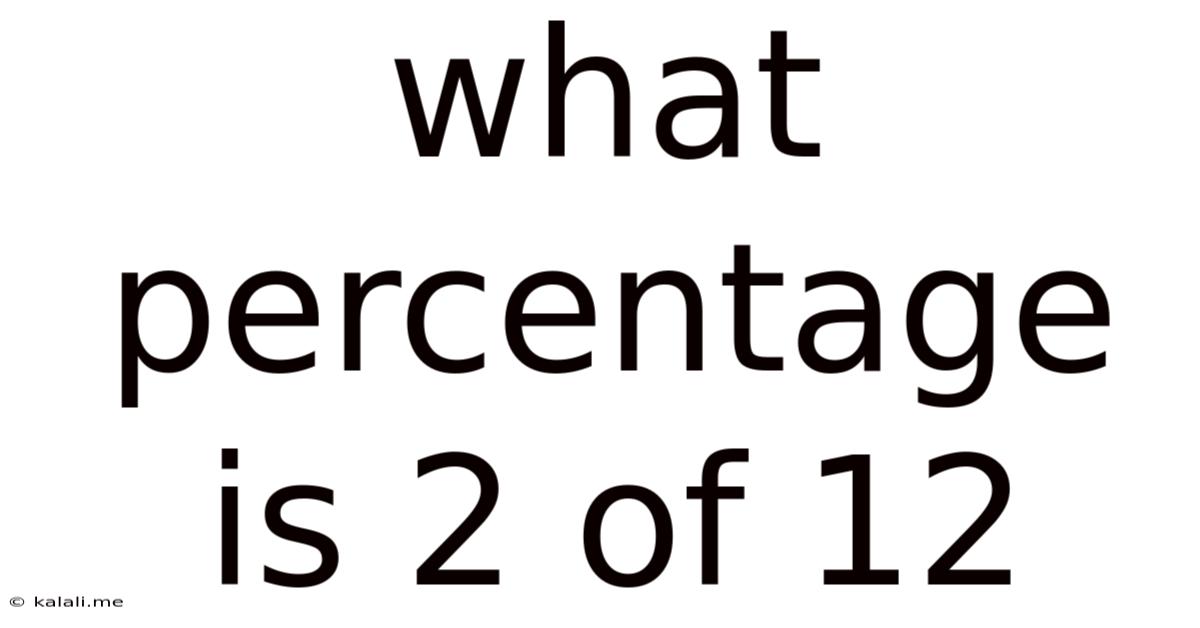
Table of Contents
What Percentage is 2 of 12? A Deep Dive into Percentage Calculations and Their Applications
This seemingly simple question, "What percentage is 2 of 12?", opens the door to a world of percentage calculations and their widespread applications in various fields. Understanding percentages is fundamental to interpreting data, making informed decisions, and solving problems in everyday life, from finance and shopping to science and statistics. This article will not only answer the initial question but delve into the underlying principles, explore different calculation methods, and highlight real-world examples to solidify your understanding.
Meta Description: Learn how to calculate percentages, understand the concept of "what percentage is 2 of 12?", and explore diverse applications of percentage calculations in everyday life and various fields. This comprehensive guide covers different methods and real-world examples.
What Percentage is 2 of 12? The Basic Calculation
The most straightforward way to determine what percentage 2 represents of 12 is to use the following formula:
(Part / Whole) * 100%
In this case:
- Part: 2
- Whole: 12
Therefore, the calculation is:
(2 / 12) * 100% = 16.67%
So, 2 is 16.67% of 12. This is the fundamental answer to the question. However, understanding the underlying concepts and exploring different approaches is crucial for broader comprehension.
Understanding the Concept of Percentages
A percentage is a way of expressing a number as a fraction of 100. It represents a portion or proportion of a whole. The symbol "%" signifies "per hundred" or "out of 100." Essentially, percentages provide a standardized way to compare different quantities or proportions. For example, scoring 80% on a test means you answered 80 out of 100 questions correctly, or 8 out of 10 if the test had only 10 questions.
The ability to convert between fractions, decimals, and percentages is crucial. Understanding these relationships enhances your ability to solve percentage problems effectively.
- Fraction to Percentage: Divide the numerator by the denominator and multiply by 100%. (e.g., 1/4 = 0.25 * 100% = 25%)
- Decimal to Percentage: Multiply the decimal by 100%. (e.g., 0.75 * 100% = 75%)
- Percentage to Decimal: Divide the percentage by 100. (e.g., 60% = 60/100 = 0.6)
- Percentage to Fraction: Express the percentage as a fraction with a denominator of 100 and simplify. (e.g., 25% = 25/100 = 1/4)
Alternative Methods for Calculating Percentages
While the basic formula is sufficient, other methods can be used, especially when dealing with more complex scenarios or mental calculations.
-
Using Proportions: Set up a proportion: 2/12 = x/100. Cross-multiply and solve for x: 12x = 200; x = 200/12 = 16.67.
-
Simplifying Fractions: Before multiplying by 100%, simplify the fraction if possible. 2/12 simplifies to 1/6. Then (1/6) * 100% = 16.67%. This simplifies the calculation.
-
Mental Estimation: For quick estimations, use benchmark percentages. Knowing that 1/6 is approximately 16.7% can help you quickly approximate the answer in various contexts.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in various aspects of life. Here are a few examples:
-
Finance: Calculating interest rates on loans, mortgages, savings accounts, and credit cards. Determining discounts on purchases, calculating taxes, and understanding investment returns all involve percentage calculations. For example, if you receive a 20% discount on a $100 item, the discount amount is 20% of $100, which is $20.
-
Shopping: Calculating discounts and sales tax, comparing prices, determining the unit price of products, and understanding value propositions. This helps consumers make informed purchasing decisions.
-
Science and Statistics: Representing data as percentages helps visualize and interpret trends. This is crucial in various fields, including medical research, environmental studies, and market analysis. Percentage changes are frequently used to show increases or decreases over time.
-
Education: Grading systems often use percentages to represent student performance. Calculating the weighted average of different assignments, based on the percentage contribution of each, is a common practice.
-
Everyday Life: Calculating tips at restaurants, understanding the nutritional content of food, measuring the completion of tasks, or comparing statistical data often involves percentage computations.
Advanced Percentage Calculations
Beyond the basic calculation, more complex percentage problems exist:
-
Percentage Increase/Decrease: Calculating the percentage change between two values. The formula is:
[(New Value - Old Value) / Old Value] * 100%. This is frequently used to track changes in prices, population, or any other quantifiable data. -
Finding the Original Value: If you know the percentage and the resulting value after a percentage increase or decrease, you can work backward to find the original value.
-
Percentage Points: This is often misused. A change of 10 percentage points means a direct numerical difference of 10, whereas a 10% increase from 10% to 20% means a 100% increase.
Practical Examples in Detail
Let's illustrate percentage calculations with detailed examples:
Example 1: Calculating a Discount
A shirt costs $50, and there's a 30% discount. What's the final price?
- Calculate the discount amount: 30% of $50 = (30/100) * $50 = $15
- Subtract the discount from the original price: $50 - $15 = $35
- The final price is $35.
Example 2: Calculating a Percentage Increase
The population of a town increased from 10,000 to 12,000. What is the percentage increase?
- Find the difference: 12,000 - 10,000 = 2,000
- Calculate the percentage increase: (2,000 / 10,000) * 100% = 20%
- The population increased by 20%.
Example 3: Finding the Original Value
A product is currently priced at $60 after a 25% increase. What was the original price?
Let 'x' be the original price.
- Set up the equation: x + 0.25x = $60
- Simplify: 1.25x = $60
- Solve for x: x = $60 / 1.25 = $48
- The original price was $48.
Conclusion:
Understanding percentage calculations is a valuable skill applicable across numerous fields. While the question "What percentage is 2 of 12?" provides a simple starting point, the principles discussed here extend to more complex scenarios. Mastering these concepts enhances your ability to analyze data, make informed decisions, and navigate various aspects of everyday life, from personal finance to professional endeavors. By using the formulas and approaches outlined in this article, you can confidently tackle various percentage problems and gain a deeper appreciation for their importance in quantitative reasoning. Remember to practice regularly to further solidify your understanding and build your skills.
Latest Posts
Latest Posts
-
What Happened To Christopher F Lima And Tim Loock
Jul 13, 2025
-
Gifts That Begin With The Letter M
Jul 13, 2025
-
What Fraction Is Equivalent To 1 2
Jul 13, 2025
-
What Is The Average Iq For A 9 Year Old
Jul 13, 2025
-
How Long Does It Take For Brandy Melville To Ship
Jul 13, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 2 Of 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.