What Percentage Is 2 Of 15
Kalali
Apr 18, 2025 · 4 min read
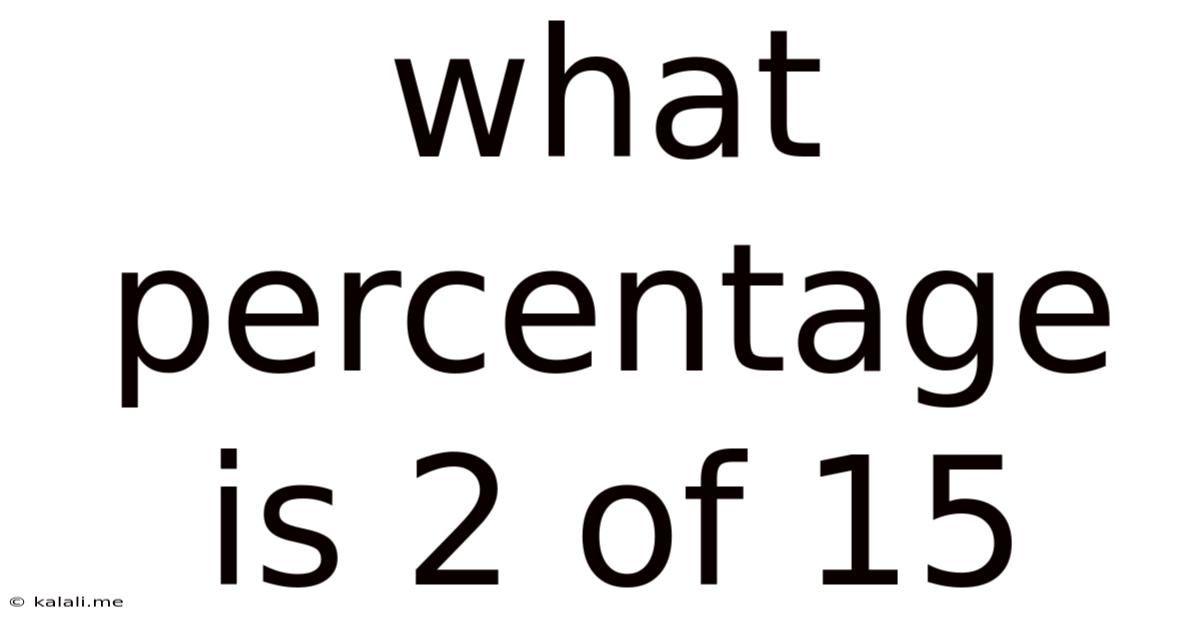
Table of Contents
What Percentage is 2 of 15? A Deep Dive into Percentage Calculations and Applications
Meta Description: This comprehensive guide explores how to calculate what percentage 2 is of 15, covering the basic formula, step-by-step solutions, real-world applications, and advanced percentage concepts. Learn to confidently tackle percentage problems and improve your mathematical skills.
Understanding percentages is a fundamental skill applicable across numerous fields, from finance and budgeting to scientific analysis and everyday decision-making. This article will not only answer the question "What percentage is 2 of 15?" but also delve into the underlying principles, explore various calculation methods, and highlight practical applications of percentage calculations.
Understanding the Basics of Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred" (from the Latin per centum). Therefore, 25% means 25 out of 100, or 25/100, which simplifies to 1/4. Percentages are a way to represent proportions, allowing for easy comparison and understanding of relative quantities.
Calculating "What Percentage is 2 of 15?"
The core formula for calculating percentages is:
(Part / Whole) x 100% = Percentage
In our case:
- Part: 2
- Whole: 15
Let's apply the formula:
(2 / 15) x 100% = 13.33% (approximately)
Therefore, 2 is approximately 13.33% of 15.
Step-by-Step Calculation:
- Divide the part by the whole: 2 ÷ 15 = 0.133333...
- Multiply the result by 100: 0.133333... x 100 = 13.3333...
- Round to the desired precision: Depending on the context, you might round to one decimal place (13.3%), two decimal places (13.33%), or more.
Alternative Calculation Methods
While the above method is straightforward, alternative approaches can enhance understanding and cater to different preferences:
-
Using proportions: You can set up a proportion: 2/15 = x/100. Solving for x gives you the percentage. Cross-multiplying (2 x 100 = 15x) and then dividing by 15 (200/15 = x) will yield the same result.
-
Using decimal conversion: Convert the fraction 2/15 to a decimal (0.1333...) and then multiply by 100 to obtain the percentage.
Real-World Applications of Percentage Calculations
Percentage calculations are ubiquitous in everyday life and professional settings. Consider these examples:
-
Finance: Calculating interest rates, discounts, profit margins, tax rates, and investment returns all rely heavily on percentage calculations. For example, if a store offers a 20% discount on a $50 item, the discount amount is (20/100) x $50 = $10.
-
Science and Statistics: Percentages are essential for expressing experimental results, analyzing data, and representing proportions in various scientific fields. For instance, researchers might report that 15% of participants in a study showed a particular outcome.
-
Retail and Sales: Understanding markups, discounts, and sales tax percentages is crucial for businesses and consumers alike. Calculating the final price of an item after a discount and sales tax involves several percentage calculations.
-
Healthcare: Doctors and nurses use percentages to describe the effectiveness of treatments, the prevalence of diseases, and the concentration of medications.
-
Education: Grading systems, test scores, and performance evaluations often use percentages to represent student achievement. For example, a student scoring 85 out of 100 on a test achieves an 85% score.
Advanced Percentage Concepts
Beyond the basic calculations, understanding advanced concepts expands your capabilities:
-
Percentage Change: This involves calculating the increase or decrease in a value expressed as a percentage. The formula is: [(New Value - Old Value) / Old Value] x 100%. This is crucial for analyzing trends and comparing data over time. For example, if sales increased from $1000 to $1200, the percentage increase is [(1200-1000)/1000] x 100% = 20%.
-
Percentage Point Difference: This is often confused with percentage change. It simply represents the arithmetic difference between two percentages. For example, if the unemployment rate rose from 5% to 7%, the percentage point difference is 2 percentage points, not a 40% increase.
-
Compounding Percentages: This is relevant for situations where a percentage change is applied repeatedly, such as with compound interest. The effect of compounding over time can be significant.
-
Weighted Averages and Percentages: When dealing with different proportions of values, weighted averages are necessary. For example, if a student receives 70% on one assignment and 90% on another, and the assignments are weighted 40% and 60% respectively, their final grade would be calculated using a weighted average.
Tips for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable you'll become with the concepts and calculations.
-
Use different methods: Trying different calculation approaches helps build a deeper understanding.
-
Understand the context: Pay close attention to the specific meaning of the percentage in any given problem.
-
Check your work: Always double-check your calculations to ensure accuracy.
-
Use calculators or software: While manual calculations are beneficial for learning, using calculators or spreadsheets can speed up more complex calculations.
Conclusion
Calculating percentages is an essential mathematical skill with broad applications. Knowing that 2 is approximately 13.33% of 15 is just the starting point. By understanding the underlying principles, various calculation methods, and advanced concepts, you can confidently tackle a wide range of percentage problems and apply this knowledge to improve your problem-solving skills in various aspects of life. Remember to practice regularly and leverage available tools to master this vital skill. The ability to quickly and accurately calculate percentages can significantly enhance your analytical capabilities and decision-making process.
Latest Posts
Latest Posts
-
Cuanto Es 13 Grados Fahrenheit En Centigrados
Apr 19, 2025
-
How Much Is 39 Degrees Celsius In Fahrenheit
Apr 19, 2025
-
37 Inches Is How Many Centimeters
Apr 19, 2025
-
75 Is What Percent Of 25
Apr 19, 2025
-
What Does The Circle In A Triangle Mean
Apr 19, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 2 Of 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.