What Percentage Is 43 Out Of 50
Kalali
Mar 09, 2025 · 5 min read
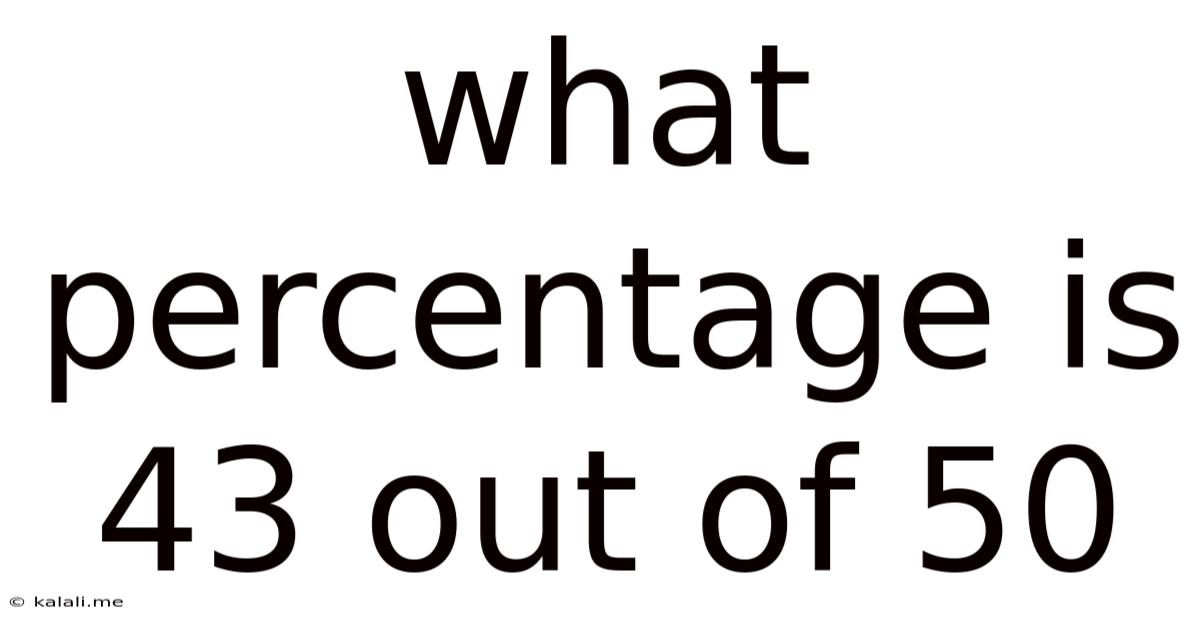
Table of Contents
What Percentage is 43 out of 50? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating grades and discounts to analyzing financial statements and comprehending statistical data. This article delves into the specifics of determining what percentage 43 represents out of 50, offering a step-by-step explanation, various calculation methods, and practical applications. We will also explore related concepts to solidify your understanding of percentage calculations.
Calculating the Percentage: A Step-by-Step Approach
The most straightforward way to find what percentage 43 is of 50 involves a simple two-step process:
Step 1: Divide the part by the whole.
In this case, 43 is the "part," and 50 is the "whole." Therefore, we perform the following division:
43 ÷ 50 = 0.86
Step 2: Multiply the result by 100 to express the answer as a percentage.
Taking the result from Step 1 (0.86) and multiplying it by 100 gives us:
0.86 × 100 = 86%
Therefore, 43 out of 50 is 86%.
Alternative Calculation Methods
While the above method is the most common and generally easiest to understand, there are alternative methods to arrive at the same result. These alternative methods can be helpful in different contexts or for those who prefer different approaches to problem-solving.
Using Proportions
We can set up a proportion to solve this problem. A proportion is an equation stating that two ratios are equal. In this case, we can set up the proportion:
43/50 = x/100
Where 'x' represents the percentage we are trying to find. To solve for x, we cross-multiply:
50x = 4300
Then, divide both sides by 50:
x = 4300 ÷ 50 = 86
Therefore, x = 86%, confirming our previous result.
Using a Calculator
Most calculators have a percentage function. Simply enter "43 ÷ 50 =" and then multiply the result by 100. Many calculators even have a dedicated percentage button that simplifies this process even further. This method is particularly useful for larger or more complex percentage calculations.
Practical Applications of Percentage Calculations
Understanding percentage calculations has numerous practical applications in various fields. Here are a few examples:
-
Academic Performance: Students frequently calculate their grades based on the percentage of correct answers obtained on exams or assignments. If a student answers 43 out of 50 questions correctly on a test, their score is 86%, as calculated above.
-
Financial Analysis: Percentages are crucial in financial analysis, used to understand profit margins, rates of return, and changes in financial statements. For instance, comparing sales figures year-over-year involves calculating percentage increases or decreases.
-
Discount Calculations: Retail stores frequently advertise discounts as percentages. For example, a "20% off" sale means you pay 80% of the original price. Understanding percentages allows you to quickly calculate the final price after a discount.
-
Statistical Analysis: Percentages are commonly used to represent data in surveys, polls, and other statistical analyses. For example, a survey might report that 86% of respondents prefer a particular product.
-
Tax Calculations: Taxes are often calculated as a percentage of income, sales, or property value. Understanding percentage calculations helps individuals and businesses accurately calculate and pay their taxes.
Understanding Related Percentage Concepts
To further enhance your understanding of percentages, it's helpful to grasp related concepts such as:
-
Percentage Increase: This refers to the increase in a value expressed as a percentage of the original value. For example, if a value increases from 50 to 65, the percentage increase is calculated as [(65-50)/50] * 100 = 30%.
-
Percentage Decrease: This represents the decrease in a value expressed as a percentage of the original value. If a value decreases from 50 to 35, the percentage decrease is calculated as [(50-35)/50] * 100 = 30%.
-
Finding the Whole When Given a Percentage and Part: Sometimes you know the percentage and the part, but need to find the whole. For instance, if 86% of a number is 43, you can set up the equation: 0.86x = 43. Solving for x gives x = 50, which is the whole.
-
Converting Fractions and Decimals to Percentages: Percentages, fractions, and decimals are all interchangeable. To convert a fraction (like 43/50) to a percentage, divide the numerator by the denominator and multiply by 100. To convert a decimal (like 0.86) to a percentage, multiply by 100.
Beyond the Basics: Advanced Percentage Applications
While calculating what percentage 43 is out of 50 is a fundamental exercise, understanding percentages extends to more complex scenarios:
-
Compound Interest: Calculating compound interest, which is interest earned on both the principal and accumulated interest, requires a strong understanding of percentage calculations.
-
Statistical Significance: In statistical analysis, percentages are used to determine the significance of findings. Understanding percentages helps researchers interpret data and draw meaningful conclusions.
-
Financial Modeling: Complex financial models rely heavily on percentage calculations to forecast future performance, assess risk, and make informed investment decisions.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is an invaluable skill with wide-ranging applications. Whether you're a student calculating your grades, a business owner analyzing your finances, or simply an individual navigating everyday life, understanding how to calculate percentages empowers you to interpret data, make informed decisions, and solve a variety of problems effectively. This article provided a detailed explanation of how to calculate what percentage 43 represents out of 50, offering several methods to ensure a comprehensive understanding. By grasping these concepts and their applications, you can confidently tackle any percentage-related challenge that comes your way. Remember to practice regularly to solidify your understanding and build confidence in your calculations.
Latest Posts
Latest Posts
-
What Is The Average Iq For 14 Year Old
Jul 14, 2025
-
Is Banana Pudding A Homo Or Heteronegous Mixture
Jul 14, 2025
-
What Year Were You Born In If You Are 15
Jul 14, 2025
-
How Much Is A Peck Of Peppers
Jul 14, 2025
-
How Does The Monster Try To Gain Control Of Victor
Jul 14, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 43 Out Of 50 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.