What Type Of Angle Is A 154 Angle
Kalali
Apr 23, 2025 · 6 min read
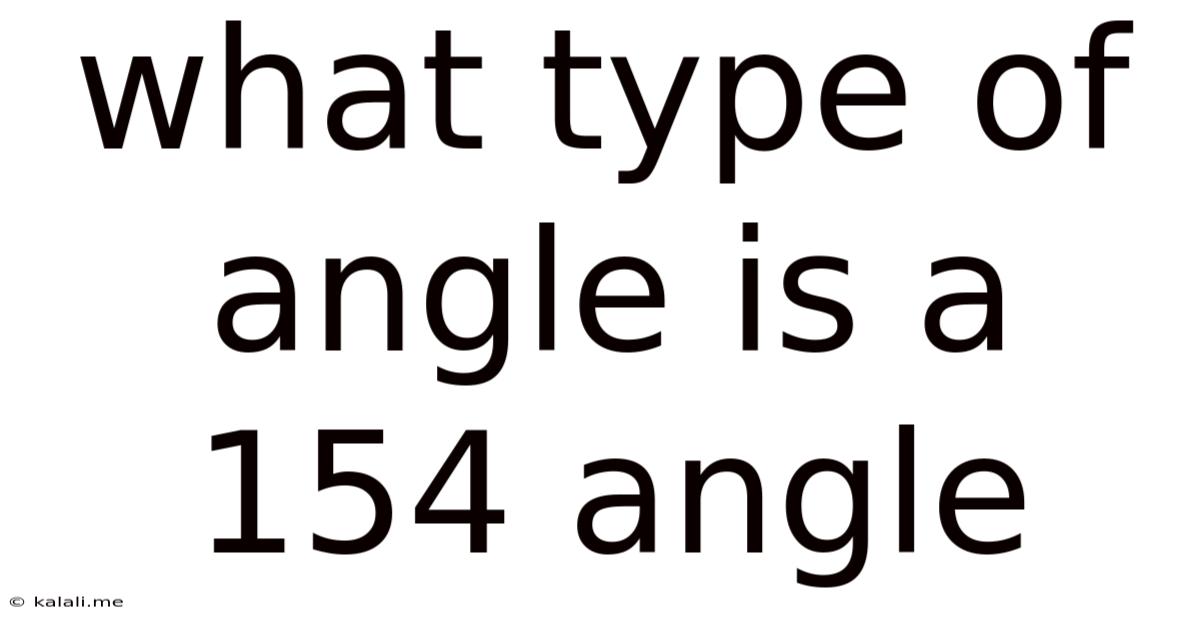
Table of Contents
Decoding the 154° Angle: Types, Properties, and Applications
A 154° angle isn't just a number; it's a geometric concept with specific properties and classifications that are crucial in various fields, from architecture and engineering to computer graphics and game development. Understanding its characteristics requires exploring its relationship to other angles and its place within the broader framework of geometry. This article delves deep into the world of the 154° angle, exploring its type, properties, and practical applications. It will also touch upon related concepts to provide a comprehensive understanding.
Meta Description: Discover the classification, properties, and real-world applications of a 154° angle. This comprehensive guide explores its relationship to other angles and its importance in various fields.
What Type of Angle is a 154° Angle?
The first step in understanding a 154° angle is classifying it based on its measure. Angles are categorized based on their size relative to standard benchmarks:
- Acute Angle: An angle measuring less than 90°.
- Right Angle: An angle measuring exactly 90°.
- Obtuse Angle: An angle measuring greater than 90° but less than 180°.
- Straight Angle: An angle measuring exactly 180°.
- Reflex Angle: An angle measuring greater than 180° but less than 360°.
- Full Angle or Perigon: An angle measuring exactly 360°.
Given its measure of 154°, a 154° angle is definitively an obtuse angle. It's larger than a right angle (90°) but smaller than a straight angle (180°). This classification is fundamental to understanding its geometric properties and how it behaves in various contexts.
Properties of a 154° Angle
Beyond its classification, several key properties define a 154° angle:
-
Supplementary Angle: The supplementary angle of a 154° angle is found by subtracting it from 180°. 180° - 154° = 26°. This means a 154° angle and a 26° angle are supplementary angles – they add up to 180°. This property is crucial in various geometric proofs and calculations.
-
Relationship to other angles: A 154° angle can be constructed using various combinations of other angles. For instance, it can be formed by combining an obtuse angle and an acute angle, or even by combining several acute angles. Understanding these relationships is essential for solving complex geometric problems.
-
Trigonometric Functions: Trigonometric functions (sine, cosine, and tangent) can be used to determine the ratios of sides in a triangle containing a 154° angle. However, it's important to note that the sine and cosine functions will produce negative values for angles greater than 90°, reflecting the angle's position in the coordinate plane.
-
Geometric Constructions: A 154° angle can be constructed using a compass and straightedge, although the process might be more involved than constructing simpler angles. This construction often involves creating intermediary angles and then combining them to achieve the desired 154° angle.
Applications of a 154° Angle
The 154° angle, while not as common as right angles or straight angles, appears in various applications:
-
Architecture and Engineering: While not frequently seen as a prominent design feature, obtuse angles, including 154° angles, can be found in structural designs, particularly in complex frameworks or where specific angles are needed to accommodate unusual terrain or existing structures. Stress analysis and load calculations for such structures require precise understanding of these angles.
-
Computer Graphics and Game Development: In computer-aided design (CAD) software and game development, precise angle calculations are crucial. Obtuse angles, such as 154°, are often used to create realistic and complex 3D models, animations, and game environments. They can determine the orientation of objects, camera angles, and lighting effects.
-
Cartography and Navigation: In geographic information systems (GIS) and navigation systems, accurate angle measurements are vital. While a 154° angle might not be a common navigational bearing, understanding angular relationships is fundamental for calculating distances, directions, and plotting routes.
-
Physics and Mechanics: The principles of angular momentum and rotational motion are extensively used in physics and mechanics. The 154° angle, like any other angle, plays a role in calculations involving rotational forces, torques, and moments of inertia. For example, the angle could represent the position of a rotating object relative to a reference point.
-
Astronomy: The study of celestial bodies relies heavily on angular measurements. While a 154° angle might not be directly associated with specific celestial events or formations, understanding angular relationships is essential for calculating distances, trajectories, and orbital mechanics.
Related Angles and Concepts
To fully grasp the significance of a 154° angle, it’s helpful to understand related concepts and angles:
-
Reference Angle: The reference angle for a 154° angle is 26°. The reference angle is the acute angle formed between the terminal side of the angle and the x-axis. This concept is particularly useful in trigonometry for simplifying calculations.
-
Coterminal Angles: Coterminal angles are angles that share the same terminal side. For example, 154° + 360° = 514°, and 154° - 360° = -206°. These angles are coterminal with 154° and share the same trigonometric values.
-
Angle Bisector: An angle bisector divides an angle into two equal parts. The bisector of a 154° angle would create two angles of 77° each.
-
Vertical Angles: When two lines intersect, they create four angles. The angles opposite each other are called vertical angles, and they are always equal. Understanding this concept can be useful when dealing with intersecting lines that form a 154° angle.
Advanced Applications and Considerations
Beyond the more common applications, a deep understanding of the 154° angle can be valuable in:
-
Robotics: Precise angular calculations are vital in robotics for controlling the movement and positioning of robotic arms and other components. The angle might be used to define the orientation of a robotic arm or the rotation of a joint.
-
Crystallography: The study of crystal structures involves analyzing the angles between crystallographic planes and directions. Obtuse angles, such as 154°, can be observed in various crystal systems.
-
Signal Processing: In digital signal processing, angles are used to represent phase shifts and other signal characteristics. An understanding of various types of angles is crucial for manipulating and interpreting signals.
Conclusion: The Significance of Understanding Angles
The 154° angle, while seemingly a simple geometric entity, holds significance across a broad spectrum of disciplines. Understanding its classification as an obtuse angle, its properties, and its relationships with other angles forms a foundational element for solving geometric problems and for understanding various applications in diverse fields. From architectural designs to computer simulations and scientific calculations, the precise measurement and manipulation of angles remain fundamental to various aspects of our technological and scientific advancements. The comprehensive understanding of angles, including angles like 154°, paves the way for innovation and progress in numerous areas. This detailed exploration highlights the importance of seemingly simple concepts in complex systems and the interconnectedness of mathematics with the real world.
Latest Posts
Latest Posts
-
Which Unit Is Used To Measure Force
Apr 23, 2025
-
Cuanto Es 6 Onzas En Ml
Apr 23, 2025
-
How To Identify A Redox Reaction
Apr 23, 2025
-
How Many Oz In 75 Ml
Apr 23, 2025
-
What Percentage Is 30 Out Of 50
Apr 23, 2025
Related Post
Thank you for visiting our website which covers about What Type Of Angle Is A 154 Angle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.