Which Function Has A Range Of Y 3
Kalali
Mar 31, 2025 · 6 min read
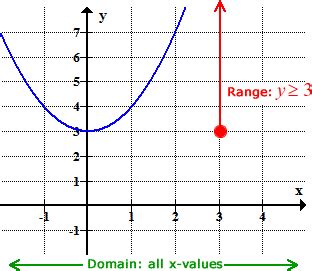
Table of Contents
Which Function Has a Range of y ≤ 3? Exploring Functions with Bounded Ranges
Determining which function possesses a specific range, like y ≤ 3, requires a deep understanding of function behavior and various mathematical concepts. This comprehensive guide explores different types of functions and their range characteristics, focusing specifically on functions whose range is less than or equal to 3. We'll delve into examples, analysis techniques, and practical applications to help you effectively identify and analyze such functions.
Understanding Function Ranges
Before we pinpoint functions with a range of y ≤ 3, let's solidify our understanding of function ranges. A function, in simple terms, is a relation where each input (x-value) corresponds to exactly one output (y-value). The domain of a function represents all possible input values, while the range encompasses all possible output values. In our case, we are interested in functions where the output (y-value) is always less than or equal to 3.
Key Concepts:
-
Bounded vs. Unbounded Ranges: A range is considered bounded if it's confined within a specific interval, like our target range y ≤ 3. Conversely, an unbounded range extends infinitely in one or both directions. For instance, the range of f(x) = x is unbounded because it encompasses all real numbers.
-
Identifying Ranges Graphically: Visually inspecting a function's graph provides a quick way to determine its range. The range represents the set of all y-values the graph covers. If the graph extends infinitely upwards, the range is unbounded in that direction. If it has a clear upper or lower limit, the range is bounded accordingly.
-
Algebraic Analysis: For functions defined algebraically, analyzing the equation can often reveal the range. This may involve considering the function's behavior, asymptotes, and transformations.
Functions with a Range of y ≤ 3: Examples and Analysis
Several function types can exhibit a range of y ≤ 3. Let's examine some key examples and how their properties contribute to their restricted range:
1. Quadratic Functions
Quadratic functions, in their standard form f(x) = ax² + bx + c, can have a maximum or minimum value depending on the sign of 'a'. If 'a' is negative (a < 0), the parabola opens downwards, resulting in a maximum value. We can manipulate the parameters (a, b, c) to ensure this maximum value is 3 or less.
Example: Consider f(x) = -x² + 3. The parabola opens downwards, and its vertex is at (0, 3). Thus, the range of this function is y ≤ 3.
Analysis: To find the range of a quadratic function, complete the square to express it in vertex form, f(x) = a(x - h)² + k, where (h, k) represents the vertex. If a < 0, the range is y ≤ k.
2. Square Root Functions
Square root functions of the form f(x) = √(g(x)) are inherently non-negative (y ≥ 0). By carefully constructing g(x), we can limit the output to y ≤ 3.
Example: Consider f(x) = √(9 - x²). This represents the upper semicircle of a circle with radius 3 centered at the origin. The range is 0 ≤ y ≤ 3.
Analysis: Analyze the expression inside the square root. Ensure that the expression is non-negative within the domain and that the maximum value of the entire function does not exceed 3.
3. Trigonometric Functions
Trigonometric functions like sine and cosine are periodic and oscillate between -1 and 1. By applying transformations, we can restrict their range to y ≤ 3.
Example: Consider f(x) = 2sin(x) + 3. The sine function oscillates between -1 and 1. Multiplying by 2 stretches the amplitude to -2 and 2, and adding 3 shifts the function vertically, resulting in a range of 1 ≤ y ≤ 5. However, we need to modify this to have a range of y ≤ 3. One possible solution is f(x) = 2sin(x) + 1 which would yield a range between -1 and 3.
Analysis: For sinusoidal functions, the amplitude and vertical shift are crucial in determining the range. Adjust these parameters to restrict the range to y ≤ 3.
4. Exponential Functions
Exponential functions of the form f(x) = abˣ, where a and b are constants, can have ranges that are either bounded below or above. However, making the range of a standard exponential function be y≤3 directly is not easily possible without restricting the domain.
5. Piecewise Functions
Piecewise functions offer tremendous flexibility in defining ranges. By carefully defining different functions for different intervals of the domain, we can construct a piecewise function with a range of y ≤ 3.
Example: Consider a piecewise function where f(x) = 3 for x ≥ 0 and f(x) = x for x < 0. In this case, the range would be y ≤ 3.
Analysis: Carefully choose sub-functions and their respective domains to ensure that the combined output never exceeds 3.
Techniques for Determining Ranges
Several techniques can be employed to determine the range of a function, especially when aiming for a specific range like y ≤ 3:
-
Graphical Analysis: Sketch the graph of the function. Observe the y-values the graph covers. This provides a visual confirmation of the range.
-
Algebraic Manipulation: Use algebraic techniques like completing the square (for quadratics), solving inequalities, or analyzing the behavior of functions to find the range.
-
Calculus: For more complex functions, calculus techniques like finding critical points and analyzing concavity can help determine the maximum or minimum values, thereby defining the range.
Applications of Functions with Bounded Ranges
Functions with bounded ranges have numerous applications in various fields:
-
Probability and Statistics: Probability density functions often have bounded ranges, representing the probability of events within a specific interval.
-
Signal Processing: Signals in communication systems are often bounded to avoid signal distortion or clipping.
-
Modeling Physical Phenomena: Many physical phenomena are modeled using functions with bounded ranges, such as the amplitude of a vibrating string or the intensity of light.
-
Computer Graphics: Bounded functions are essential in defining colors, shapes, and textures in computer graphics applications. The output often needs to be limited to certain values within the system's color space.
Conclusion
Identifying functions with a specific range, such as y ≤ 3, requires a thorough understanding of function properties and analytical techniques. We've explored several function types and demonstrated how manipulating their parameters or employing piecewise functions can yield the desired range. The techniques outlined – graphical analysis, algebraic manipulation, and calculus – provide a versatile toolkit for tackling such problems. Remember that the specific approach will depend on the function's complexity and form. By mastering these techniques, you can effectively analyze functions and their ranges, paving the way for deeper exploration of mathematical concepts and their practical applications. Further research into specific function types and their transformations can enhance your ability to handle more complex scenarios. Remember to always consider the domain in conjunction with the range analysis for a complete understanding of the function's behavior.
Latest Posts
Latest Posts
-
Which Word Has The Most Positive Connotation
Jul 15, 2025
-
How Do I Send An Evite Reminder
Jul 15, 2025
-
When Performing A Self Rescue When Should You Swim To Shore
Jul 15, 2025
-
How Many Decaliters Are In A Liter
Jul 15, 2025
-
What Note Sits In The Middle Of The Grand Staff
Jul 15, 2025
Related Post
Thank you for visiting our website which covers about Which Function Has A Range Of Y 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.