10 2 As A Mixed Number
Kalali
Apr 01, 2025 · 4 min read
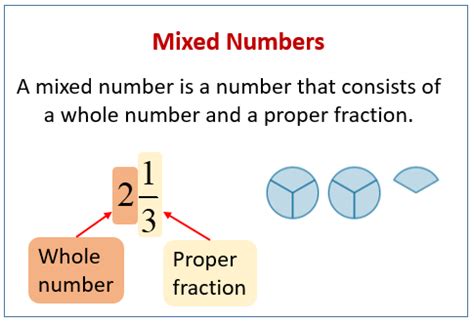
Table of Contents
10/2 as a Mixed Number: A Comprehensive Guide
Understanding fractions and their conversions is fundamental to grasping mathematical concepts. This comprehensive guide delves into the conversion of the improper fraction 10/2 into a mixed number, exploring the process step-by-step and providing practical examples to solidify your understanding. We'll also examine related concepts and troubleshooting common mistakes.
What is a Mixed Number?
Before we dive into the conversion, let's define what a mixed number is. A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (top number) is smaller than the denominator (bottom number). For example, 1 ¾, 2 ⅓, and 5 ⅛ are all mixed numbers.
Converting 10/2 to a Mixed Number
The improper fraction 10/2 represents ten divided by two. To convert this to a mixed number, we perform a simple division.
Step 1: Divide the Numerator by the Denominator
Divide the numerator (10) by the denominator (2):
10 ÷ 2 = 5
Step 2: Determine the Whole Number
The result of the division (5) becomes the whole number part of your mixed number.
Step 3: Determine the Remainder (if any)
In this case, there is no remainder since 2 divides evenly into 10. If there was a remainder, it would become the numerator of the fractional part of the mixed number. The denominator remains the same as the original fraction's denominator.
Step 4: Write the Mixed Number
Since there's no remainder, the mixed number equivalent of 10/2 is simply 5. There is no fractional part because the division resulted in a whole number.
Understanding the Concept of Improper Fractions
An improper fraction is a fraction where the numerator is greater than or equal to the denominator. Examples include 7/4, 10/2, and 15/5. Improper fractions represent values greater than or equal to one. Converting improper fractions to mixed numbers offers a clearer representation of the value, especially when working with real-world applications.
Practical Applications and Real-World Examples
Understanding the conversion of improper fractions like 10/2 to mixed numbers is essential in various practical scenarios:
- Cooking: A recipe might call for 10/2 cups of flour. Knowing that 10/2 simplifies to 5 makes the measurement much easier.
- Construction: Measuring materials in fractions is common. Converting improper fractions to mixed numbers simplifies measurements and reduces errors.
- Sewing: Pattern instructions often utilize fractions. Converting improper fractions clarifies instructions and improves accuracy.
- Data Analysis: In statistical calculations, simplifying improper fractions to mixed numbers can improve data interpretation.
Working with Remainders: A More Complex Example
Let's consider a slightly more complex example to illustrate handling remainders during the conversion: Convert 17/5 to a mixed number.
Step 1: Divide the Numerator by the Denominator
17 ÷ 5 = 3 with a remainder of 2
Step 2: Determine the Whole Number
The quotient (3) is the whole number part of the mixed number.
Step 3: Determine the Remainder and Fractional Part
The remainder (2) becomes the numerator of the fraction, and the denominator remains 5.
Step 4: Write the Mixed Number
The mixed number is 3 ⅔.
Why Convert Improper Fractions to Mixed Numbers?
Converting improper fractions to mixed numbers offers several advantages:
- Clarity: Mixed numbers often provide a more intuitive understanding of the quantity being represented. For example, 3 ⅔ is easier to visualize than 17/5.
- Ease of Calculation: In some calculations, especially addition and subtraction, working with mixed numbers can be simpler than working with improper fractions.
- Real-world Applicability: Mixed numbers are frequently used in everyday situations, making their understanding crucial for practical applications.
Common Mistakes to Avoid
While the process of converting improper fractions to mixed numbers is relatively straightforward, some common mistakes can occur:
- Incorrect Division: Ensure accurate division when converting. Double-check your calculations to avoid errors.
- Misplacing the Remainder: Remember the remainder becomes the numerator of the fractional part, and the denominator remains unchanged.
- Forgetting the Whole Number: The quotient (result of the division) is the whole number part of the mixed number; don't omit it.
Further Practice and Exploration
To solidify your understanding, try converting these improper fractions to mixed numbers:
- 12/4
- 23/7
- 35/6
- 41/9
- 50/11
Remember to always divide the numerator by the denominator, note any remainder, and express your answer as a whole number and a proper fraction.
Conclusion: Mastering Mixed Numbers
Converting improper fractions to mixed numbers is a fundamental skill in mathematics. By understanding the steps involved and practicing regularly, you can confidently navigate this process and apply it to various real-world scenarios. Remember to check your work and avoid common pitfalls for accurate results. Mastering this skill will significantly improve your mathematical abilities and problem-solving skills across different contexts. From everyday tasks to complex calculations, a strong grasp of mixed numbers provides a solid foundation for future mathematical endeavors. The simple conversion of 10/2 to 5, while seemingly basic, underscores the importance of understanding fundamental mathematical concepts. This seemingly simple conversion provides a strong foundation for more advanced mathematical concepts and applications. The ability to seamlessly convert between improper fractions and mixed numbers enhances your mathematical fluency and opens up opportunities for success in various fields.
Latest Posts
Latest Posts
-
Differentiate Between Extrusive And Intrusive Rocks
Apr 02, 2025
-
How Do You Write A Congruence Statement
Apr 02, 2025
-
What Is The Relationship Between Electron Affinity And Atomic Radius
Apr 02, 2025
-
What Is 44 50 As A Percent
Apr 02, 2025
-
Least Common Factor Of 7 And 8
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 10 2 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
