11 Is What Percent Of 20
Kalali
Apr 08, 2025 · 6 min read
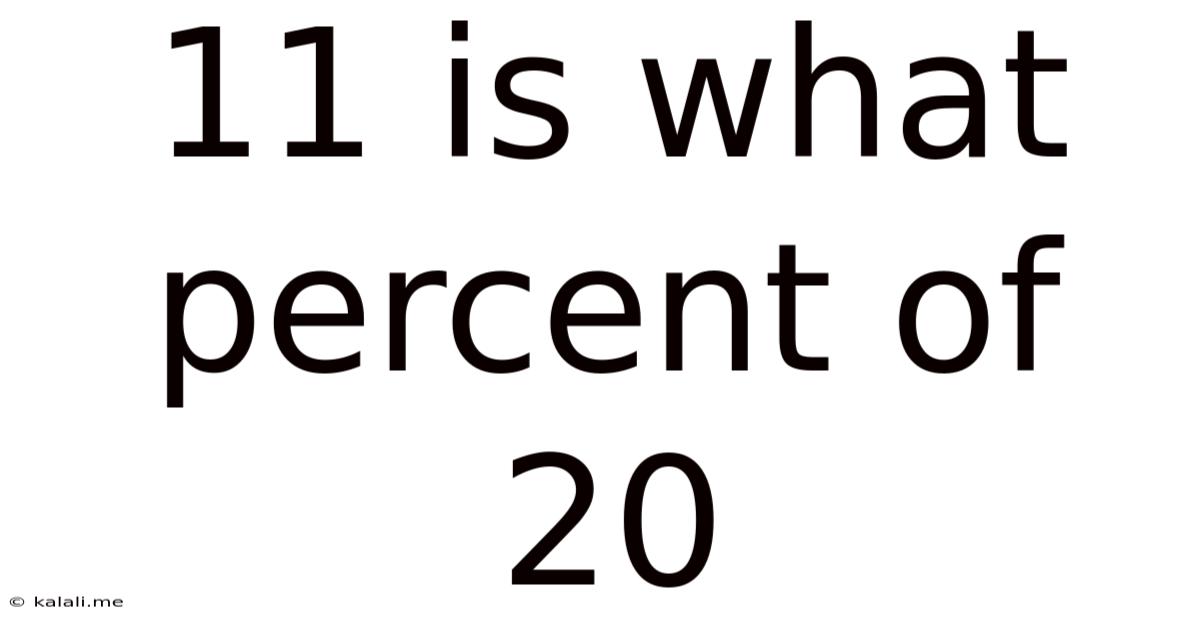
Table of Contents
11 is What Percent of 20? A Comprehensive Guide to Percentage Calculations
This seemingly simple question, "11 is what percent of 20?", opens the door to a world of percentage calculations crucial for various aspects of life, from understanding sales discounts to analyzing financial reports. This comprehensive guide will not only answer this specific question but also equip you with the knowledge and skills to tackle any percentage problem confidently. We'll explore different methods of calculation, discuss practical applications, and delve into the underlying mathematical concepts.
Meta Description: Learn how to calculate percentages with ease! This detailed guide explains how to determine what percentage 11 represents of 20, covering various methods, real-world applications, and helpful tips. Master percentage calculations today!
Understanding Percentages
Before diving into the calculation, let's clarify what a percentage represents. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." So, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
This fundamental understanding is crucial for comprehending percentage calculations. Many real-world applications utilize percentages to express proportions, rates, and changes. From calculating sales tax and discounts to understanding interest rates and statistical data, percentages are an essential tool for navigating numerical information.
Method 1: The Proportion Method
This classic method utilizes the concept of proportions to solve percentage problems. We set up a proportion where one ratio represents the given values (11 out of 20) and the other represents the unknown percentage (x out of 100).
Here's how to apply this method to our question, "11 is what percent of 20?":
-
Set up the proportion: 11/20 = x/100
-
Cross-multiply: 20x = 1100
-
Solve for x: x = 1100 / 20 = 55
Therefore, 11 is 55% of 20.
This method is straightforward and easily understandable, making it ideal for beginners. The visual representation of the proportion helps solidify the understanding of the relationship between the parts and the whole.
Method 2: The Decimal Method
This method converts the fraction representing the parts to a decimal and then multiplies by 100 to express it as a percentage.
Applying this to our problem:
-
Express the fraction: 11/20
-
Convert to a decimal: 11 ÷ 20 = 0.55
-
Multiply by 100 to get the percentage: 0.55 * 100 = 55%
This method is efficient and relies on basic arithmetic operations, making it suitable for quick calculations. The conversion to decimal provides a clear numerical representation of the proportion. This is a highly useful method when dealing with calculators or computer programs.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process even further. The specific steps might vary depending on the calculator model, but the general approach involves entering the fraction (11/20) or the division (11 ÷ 20) and then using the percentage function (often denoted as "%"). The calculator will directly output the result: 55%. This method is particularly convenient for more complex percentage problems.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in numerous real-world scenarios. Here are some examples:
-
Sales and Discounts: Calculating discounts offered by stores, understanding the final price after a discount is applied. For instance, a 20% discount on a $100 item translates to a $20 discount, resulting in a final price of $80.
-
Taxes: Determining the amount of sales tax or income tax payable based on a given percentage.
-
Interest Rates: Calculating simple or compound interest on loans, investments, or savings accounts. Understanding interest rates is crucial for making informed financial decisions. Knowing how to calculate percentage changes in interest rates allows for informed comparison of financial products.
-
Financial Analysis: Analyzing financial statements, understanding profit margins, and calculating percentage changes in revenue or expenses. For example, a company's profit margin is often expressed as a percentage of revenue. Tracking this percentage helps gauge the company's financial health.
-
Statistics and Data Analysis: Expressing proportions and ratios in datasets as percentages to make them easier to understand and compare. This is particularly relevant in surveys, polls, and other statistical studies.
-
Grades and Scores: Converting raw scores in exams or tests to percentages to represent the proportion of correct answers.
-
Tips and Gratuities: Calculating appropriate tips or gratuities in restaurants or other service industries based on a given percentage of the total bill. A 15% tip on a $50 meal amounts to a $7.50 tip.
-
Commission: Calculating commission earned by sales representatives based on a percentage of sales revenue. A sales representative earning a 5% commission on $10,000 in sales earns $500.
-
Growth and Decrease: Calculating percentage change to understand the growth or decline in a particular quantity. For example, population growth is often reported as a percentage increase over a given period.
Beyond the Basics: More Complex Percentage Problems
While the "11 is what percent of 20?" problem provides a foundation, percentage calculations can become more complex. Consider scenarios involving:
-
Percentage Increase/Decrease: Calculating the percentage increase or decrease between two values. For example, if a stock price increases from $10 to $12, the percentage increase is 20%.
-
Successive Percentages: Calculating the overall effect of multiple percentage changes applied successively. For example, a 10% increase followed by a 10% decrease does not result in the original value; the final result is slightly less.
-
Percentage Points: Understanding the difference between a percentage change and percentage points. A change from 10% to 20% is a 10 percentage point increase, but a 100% percentage increase.
-
Finding the Original Value: Determining the original value before a percentage increase or decrease. For example, if a price increased by 15% to reach $115, the original price was $100.
Mastering Percentage Calculations: Tips and Tricks
-
Practice Regularly: The more you practice, the more comfortable and efficient you’ll become with percentage calculations.
-
Understand the Fundamentals: Ensure a solid grasp of fractions, decimals, and basic arithmetic operations.
-
Use Multiple Methods: Experiment with different calculation methods to find the one that best suits your understanding and the problem at hand.
-
Utilize Online Resources: Numerous online calculators and tutorials can assist in solving percentage problems and reinforcing your understanding.
-
Break Down Complex Problems: Divide complex percentage problems into smaller, more manageable steps.
-
Check Your Work: Always double-check your answers to ensure accuracy. Estimating the answer beforehand can help detect errors.
-
Visual Aids: Consider using diagrams or visual representations to aid in understanding complex percentage problems.
In conclusion, "11 is what percent of 20?" is more than a simple arithmetic problem; it’s a gateway to understanding a fundamental mathematical concept with far-reaching real-world applications. By mastering percentage calculations, you empower yourself with a crucial skill applicable across various domains, from personal finance to professional success. By applying the methods outlined above and practicing regularly, you can confidently tackle any percentage problem you encounter.
Latest Posts
Latest Posts
-
Common Multiples Of 3 And 6
Apr 17, 2025
-
What Is 60 Percent Of 50
Apr 17, 2025
-
How Much Is 75 Ml In Ounces
Apr 17, 2025
-
What Are The Names Of Great Lakes
Apr 17, 2025
-
What 4 Substances Are Recycled During Photosynthesis And Respiration
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about 11 Is What Percent Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.