12 Is What Percent Of 30
Kalali
Apr 02, 2025 · 5 min read
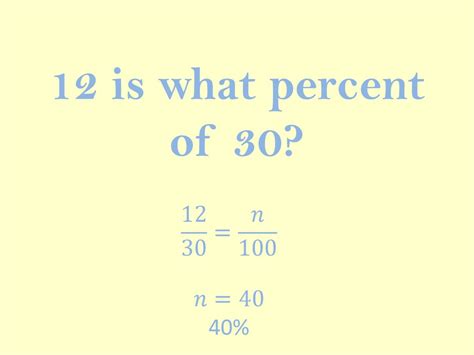
Table of Contents
12 is What Percent of 30: A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous areas of life, from calculating discounts and taxes to analyzing data and understanding financial reports. This comprehensive guide will not only answer the question, "12 is what percent of 30?" but also delve into the underlying principles of percentage calculations, providing you with the tools and knowledge to tackle similar problems with confidence.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred." Therefore, 10% means 10 out of 100, which can also be written as the fraction 10/100 or the decimal 0.10.
Understanding this fundamental concept is crucial for solving percentage problems. Whether you're calculating a discount on a product, determining the percentage increase in your salary, or analyzing statistical data, the underlying principle remains the same: expressing a part as a fraction of the whole, expressed as a value out of 100.
Calculating Percentages: Three Key Methods
There are several ways to calculate percentages. We'll explore three common methods, each offering a slightly different approach to solving the same problem.
Method 1: Using the Formula
The most common method utilizes a simple formula:
(Part / Whole) * 100% = Percentage
In our example, "12 is what percent of 30?", we can identify:
- Part: 12
- Whole: 30
Substituting these values into the formula:
(12 / 30) * 100% = 40%
Therefore, 12 is 40% of 30.
Method 2: Using Proportions
This method involves setting up a proportion to solve for the unknown percentage. A proportion is a statement that two ratios are equal. We can set up a proportion as follows:
x/100 = 12/30
Where 'x' represents the unknown percentage. To solve for 'x', we can cross-multiply:
30x = 1200
Then, divide both sides by 30:
x = 40
Therefore, 12 is 40% of 30.
Method 3: Using Decimal Conversion
This method involves converting the fraction to a decimal and then multiplying by 100%.
First, express the part as a fraction of the whole:
12/30
Next, simplify the fraction (if possible):
2/5
Convert the simplified fraction to a decimal by dividing the numerator by the denominator:
2 ÷ 5 = 0.4
Finally, multiply the decimal by 100% to express it as a percentage:
0.4 * 100% = 40%
Again, we find that 12 is 40% of 30.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in a wide range of real-world scenarios. Let's explore some examples:
1. Discounts and Sales
Stores frequently offer discounts on merchandise, often expressed as a percentage. For example, a 20% discount on a $50 item means you save 20% of $50, which is $10, resulting in a final price of $40. Percentage calculations allow you to easily determine the discounted price.
2. Taxes and Fees
Taxes and fees are often calculated as a percentage of a purchase price or income. Knowing how to calculate percentages enables you to determine the total cost of an item including taxes or the amount of tax withheld from your paycheck.
3. Financial Analysis
Percentage calculations are crucial in financial analysis. Investors use percentages to track changes in stock prices, calculate returns on investments (ROI), and analyze financial statements. Understanding percentage change (increase or decrease) is essential for making informed financial decisions.
4. Statistical Analysis
Percentages are frequently used in statistical analysis to represent proportions within a data set. For example, polling data often expresses results as percentages, enabling a clear visualization of public opinion on a particular issue. Understanding percentages allows for effective interpretation and comparison of statistical data.
5. Grade Calculations
In educational settings, grades are often expressed as percentages, reflecting the proportion of correctly answered questions or assignments completed successfully. Understanding percentages allows students to monitor their academic progress and understand their performance in relation to the total possible score.
Advanced Percentage Problems: Finding the Whole or the Part
While the initial problem focused on finding the percentage, percentage calculations can also involve finding the whole or the part, given the percentage and either the part or the whole.
Finding the Whole
Let's say you know that 25% of a certain number is 15. How do you find the whole number? You can use the formula:
Whole = (Part / Percentage) * 100
In this case:
Whole = (15 / 25) * 100 = 60
Therefore, the whole number is 60.
Finding the Part
Suppose you know that 60% of a number is 30. To find the part, you can use the formula:
Part = (Percentage / 100) * Whole
In this case:
Part = (60/100) * 50 = 30
The part is 30.
Mastering Percentage Calculations: Tips and Tricks
Mastering percentage calculations requires practice and understanding. Here are some tips and tricks:
-
Practice Regularly: The more you practice, the more comfortable you'll become with the different methods and approaches.
-
Use Different Methods: Experiment with different methods to find the one that best suits your understanding and problem-solving style.
-
Simplify Fractions: Simplifying fractions before converting to decimals can make the calculations easier.
-
Check Your Work: Always double-check your answers to ensure accuracy.
-
Use a Calculator: For complex calculations, use a calculator to avoid errors.
Conclusion: Unlocking the Power of Percentages
Understanding percentages is a crucial skill for navigating the complexities of daily life, from making informed financial decisions to comprehending statistical data. By mastering the various methods of calculating percentages and practicing regularly, you can unlock the power of percentages and confidently tackle a wide range of problems. This comprehensive guide has equipped you with the knowledge and tools to confidently approach any percentage calculation, making you a more informed and capable individual in both professional and personal endeavors. Remember, practice is key to mastering this essential skill. So, grab a calculator, work through some examples, and watch your percentage prowess grow!
Latest Posts
Latest Posts
-
What Is 8 12 As A Percent
Apr 03, 2025
-
1 73 M In Inches And Feet
Apr 03, 2025
-
How Many Hours Is 125 Minutes
Apr 03, 2025
-
4 To The Power Of 12
Apr 03, 2025
-
What Are The Disadvantages Of Sexual Reproduction
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about 12 Is What Percent Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
