2 Out Of 3 As A Percentage
Kalali
Apr 22, 2025 · 5 min read
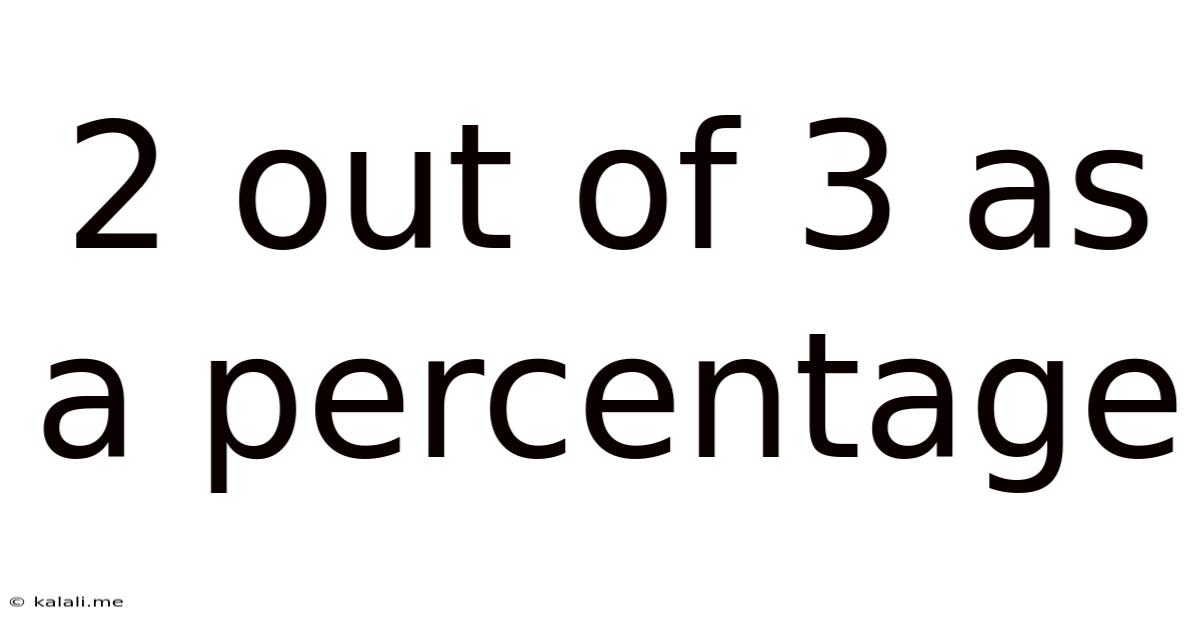
Table of Contents
2 Out of 3 as a Percentage: A Comprehensive Guide to Fraction-to-Percentage Conversion
Understanding how to convert fractions to percentages is a fundamental skill applicable across various fields, from everyday budgeting and shopping to complex scientific calculations and data analysis. This comprehensive guide will explore the intricacies of calculating "2 out of 3" as a percentage, providing multiple approaches and explaining the underlying principles. We'll also delve into practical applications and explore common misconceptions to solidify your understanding of this essential mathematical concept.
Meta Description: Learn how to calculate "2 out of 3" as a percentage. This detailed guide covers multiple methods, practical applications, and common mistakes, ensuring a thorough understanding of fraction-to-percentage conversion.
Understanding Fractions and Percentages
Before diving into the calculation, let's review the basics. A fraction represents a part of a whole. In the case of "2 out of 3," the fraction is expressed as 2/3. The numerator (2) represents the part, and the denominator (3) represents the whole.
A percentage, denoted by the symbol %, represents a fraction out of 100. Essentially, it expresses a proportion as parts per hundred. For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Converting a fraction to a percentage involves finding the equivalent fraction with a denominator of 100. This can be achieved through different methods, as explained below.
Method 1: Direct Conversion Using Division
The most straightforward method is to divide the numerator by the denominator and then multiply the result by 100. This approach works for any fraction, including 2/3.
-
Divide the numerator by the denominator: 2 ÷ 3 = 0.666666... (This is a recurring decimal)
-
Multiply the result by 100: 0.666666... × 100 = 66.6666...%
Therefore, 2 out of 3 is approximately 66.67%. The trailing digits are usually rounded to two decimal places for practical purposes.
Method 2: Finding an Equivalent Fraction with a Denominator of 100
This method involves finding an equivalent fraction to 2/3 that has a denominator of 100. While not always straightforward, this approach strengthens the understanding of fraction equivalence.
To achieve a denominator of 100, we need to determine what number, when multiplied by 3, equals 100. This involves solving the equation 3x = 100. The solution is x ≈ 33.33.
Now, multiply both the numerator and the denominator of 2/3 by this approximate value:
(2 × 33.33) / (3 × 33.33) ≈ 66.66 / 100
This shows that 2/3 is approximately equivalent to 66.66/100, which translates directly to 66.66%.
Method 3: Using Proportions
This method leverages the concept of proportions to solve the problem. We can set up a proportion to find the percentage:
2/3 = x/100
To solve for x (the percentage), cross-multiply:
3x = 200
x = 200/3 ≈ 66.67
Therefore, 2 out of 3 is approximately 66.67%.
Understanding Recurring Decimals and Rounding
As demonstrated, converting 2/3 to a percentage results in a recurring decimal (0.6666...). Rounding is necessary for practical applications. The standard practice is to round to two decimal places, resulting in 66.67%. The level of rounding depends on the context; in some cases, more decimal places might be required for greater accuracy.
Practical Applications of Fraction-to-Percentage Conversion
The ability to convert fractions to percentages is crucial in numerous real-world scenarios:
-
Calculating Grades: If you answered 2 out of 3 questions correctly on a test, your score is 66.67%.
-
Analyzing Sales Data: If 2 out of 3 customers purchased a specific product, the conversion rate is 66.67%.
-
Assessing Project Completion: If 2 out of 3 project milestones have been completed, the project is 66.67% complete.
-
Understanding Financial Statements: Financial ratios often involve fractions that need to be converted to percentages for easier interpretation.
-
Scientific Research: Data analysis in various scientific fields often involves converting fractions representing experimental results into percentages for clearer presentation and comparison.
Common Mistakes to Avoid
While the calculation is relatively straightforward, certain common mistakes should be avoided:
-
Incorrect Division: Ensure the numerator is divided by the denominator, not vice versa.
-
Forgetting to Multiply by 100: Dividing the fraction alone will not give the percentage; it's crucial to multiply the result by 100.
-
Inconsistent Rounding: Maintain consistency in the number of decimal places used for rounding throughout the calculation.
-
Misinterpretation of the Fraction: Ensure the fraction is correctly interpreted; the numerator represents the part, and the denominator represents the whole.
Beyond 2/3: Extending the Concept
The methods described above are universally applicable to any fraction. Let's consider a few examples:
-
1/4 as a percentage: 1 ÷ 4 = 0.25; 0.25 × 100 = 25%
-
3/5 as a percentage: 3 ÷ 5 = 0.6; 0.6 × 100 = 60%
-
5/8 as a percentage: 5 ÷ 8 = 0.625; 0.625 × 100 = 62.5%
Using Calculators and Spreadsheets
Modern calculators and spreadsheet software like Microsoft Excel and Google Sheets have built-in functions for percentage calculations. In Excel, the formula =2/3 will return the decimal value, and =2/3*100 will return the percentage. These tools can simplify the process significantly, especially when dealing with more complex calculations involving multiple fractions.
Conclusion: Mastering Fraction-to-Percentage Conversions
Converting fractions to percentages is a vital skill with extensive applications. By understanding the various methods – direct division, finding equivalent fractions, using proportions, and leveraging technological tools – you can confidently tackle these conversions in any context. Remembering the underlying principles and avoiding common errors ensures accuracy and mastery of this fundamental mathematical concept. This comprehensive guide aims to equip you with the knowledge and skills to perform these calculations efficiently and confidently. Remember to practice regularly to solidify your understanding and enhance your problem-solving abilities.
Latest Posts
Latest Posts
-
What Has A Definite Shape And Volume
Apr 22, 2025
-
How Many Grams In A 8th Of An Ounce
Apr 22, 2025
-
What Is Two Thirds Of 100
Apr 22, 2025
-
What Is 6 25 As A Fraction
Apr 22, 2025
-
44 Out Of 60 As A Percentage
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 2 Out Of 3 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.