31 Of 40 Is What Percent
Kalali
Apr 08, 2025 · 5 min read
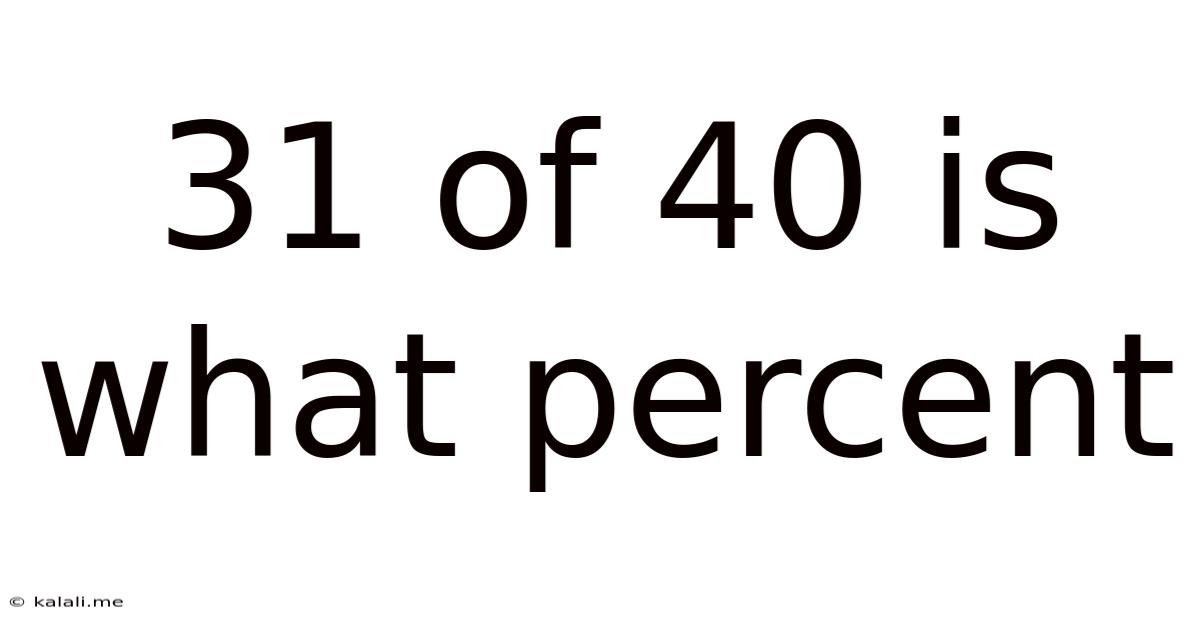
Table of Contents
31 out of 40 is What Percent? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with wide-ranging applications, from everyday budgeting and shopping to advanced statistical analysis and scientific research. Understanding how to determine percentages allows you to interpret data, make informed decisions, and communicate findings effectively. This article will delve into the calculation of "31 out of 40 is what percent?", providing a step-by-step guide, exploring different methods, and offering practical examples to solidify your understanding. We'll also cover related concepts like percentage increase, decrease, and applications in various fields.
Understanding Percentages: A Foundation
A percentage represents a fraction of 100. The word "percent" literally means "per hundred." Therefore, 25% means 25 out of 100, which can be expressed as the fraction 25/100 or the decimal 0.25. This foundational understanding is key to calculating any percentage.
Method 1: The Direct Calculation Method
The most straightforward way to determine what percentage 31 out of 40 represents is through a simple formula:
(Part / Whole) * 100 = Percentage
In this case:
- Part: 31 (the number we're interested in)
- Whole: 40 (the total number)
Applying the formula:
(31 / 40) * 100 = 77.5%
Therefore, 31 out of 40 is 77.5%.
Method 2: Using Proportions
Proportions offer another approach to solving percentage problems. We can set up a proportion:
31/40 = x/100
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
40x = 3100
x = 3100 / 40
x = 77.5
Again, we find that 31 out of 40 is 77.5%.
Method 3: Decimal Conversion and Multiplication
This method involves first converting the fraction to a decimal and then multiplying by 100:
- Convert the fraction to a decimal: 31 / 40 = 0.775
- Multiply by 100 to express as a percentage: 0.775 * 100 = 77.5%
This method is efficient and easily adaptable to various percentage calculations.
Practical Applications and Examples
The ability to calculate percentages is crucial in many real-world scenarios:
-
Academic Performance: If a student scored 31 out of 40 on a test, their percentage score is 77.5%. This allows for easy comparison of performance across different tests and subjects. Understanding percentage grades helps students track their academic progress and identify areas needing improvement. Analyzing percentage scores across multiple assessments provides a clearer picture of overall academic standing and potential areas for improvement.
-
Sales and Discounts: Imagine a store offering a discount. If an item originally costs $40 and is discounted to $31, the discount is 77.5% of the original price. Understanding discounts is vital for smart shopping and budget planning. Calculating percentage savings can help consumers make informed purchase decisions, and comparison shopping becomes more efficient when discounts are expressed as percentages. This knowledge empowers consumers to identify the best deals and optimize their spending.
-
Financial Calculations: In finance, percentages are essential for calculating interest rates, returns on investment, and analyzing financial statements. For instance, if an investment of $40 yields a profit of $31, the return on investment (ROI) is 77.5%. This highlights the importance of percentage calculations in assessing the profitability of investments. Financial planning and forecasting rely heavily on percentage analysis to model future scenarios and assess risk.
-
Data Analysis and Statistics: Percentages are fundamental in data analysis and statistical interpretations. Representing data as percentages enables easy comparison and visualization. This facilitates the identification of trends and patterns within data sets. Percentages are commonly used in surveys, polls, and research studies to summarize findings effectively and draw meaningful conclusions. Data visualization techniques like pie charts and bar graphs often utilize percentages for clear representation.
-
Survey Results: If 31 out of 40 respondents to a survey answered "yes" to a particular question, the percentage of "yes" responses is 77.5%. This concisely summarizes the survey results and allows for easy comparison with other surveys or datasets. Understanding survey results expressed as percentages helps interpret public opinion, assess consumer preferences, and inform business strategies.
Beyond Basic Percentage Calculations: Expanding Your Skills
While calculating "31 out of 40 is what percent?" is straightforward, understanding related concepts enhances your analytical capabilities:
-
Percentage Increase: This calculates the percentage change when a value increases. The formula is: [(New Value - Old Value) / Old Value] * 100.
-
Percentage Decrease: This calculates the percentage change when a value decreases. The formula is: [(Old Value - New Value) / Old Value] * 100.
-
Calculating the Whole from a Percentage: If you know a percentage and the part, you can calculate the whole using the formula: (Part / Percentage) * 100 = Whole.
Mastering Percentage Calculations: Tips and Tricks
-
Practice Regularly: Consistent practice solidifies your understanding and improves your calculation speed. Work through various examples and problem sets to build confidence.
-
Use Online Calculators: Online percentage calculators can be valuable tools for verifying your work and exploring different calculation methods. However, understanding the underlying principles remains crucial.
-
Focus on the Fundamentals: A strong grasp of fractions and decimals forms the basis for accurate percentage calculations. Review these concepts if needed.
-
Understand Context: Always consider the context of the problem. Understanding what the numbers represent helps in setting up the calculations correctly.
Conclusion
Calculating percentages is a vital skill with broad applicability. Understanding the various methods, from direct calculation to using proportions and decimal conversions, empowers you to tackle a wide range of percentage problems. By mastering these skills and expanding your knowledge to include percentage increase, decrease, and related calculations, you'll be well-equipped to analyze data, make informed decisions, and confidently navigate numerous situations involving percentages. Remember that consistent practice is key to developing fluency and accuracy in percentage calculations. The ability to perform these calculations is a valuable asset in both academic and professional contexts.
Latest Posts
Latest Posts
-
What Is A Cross Section Of A Pyramid
Apr 17, 2025
-
10 Ml Is Equal To How Many Oz
Apr 17, 2025
-
Why Was The Continental Drift Rejected
Apr 17, 2025
-
4 Out Of 6 As A Percentage
Apr 17, 2025
-
What Elements Have One Valence Electron
Apr 17, 2025
Related Post
Thank you for visiting our website which covers about 31 Of 40 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.