34 Out Of 50 As A Percentage
Kalali
Mar 09, 2025 · 5 min read
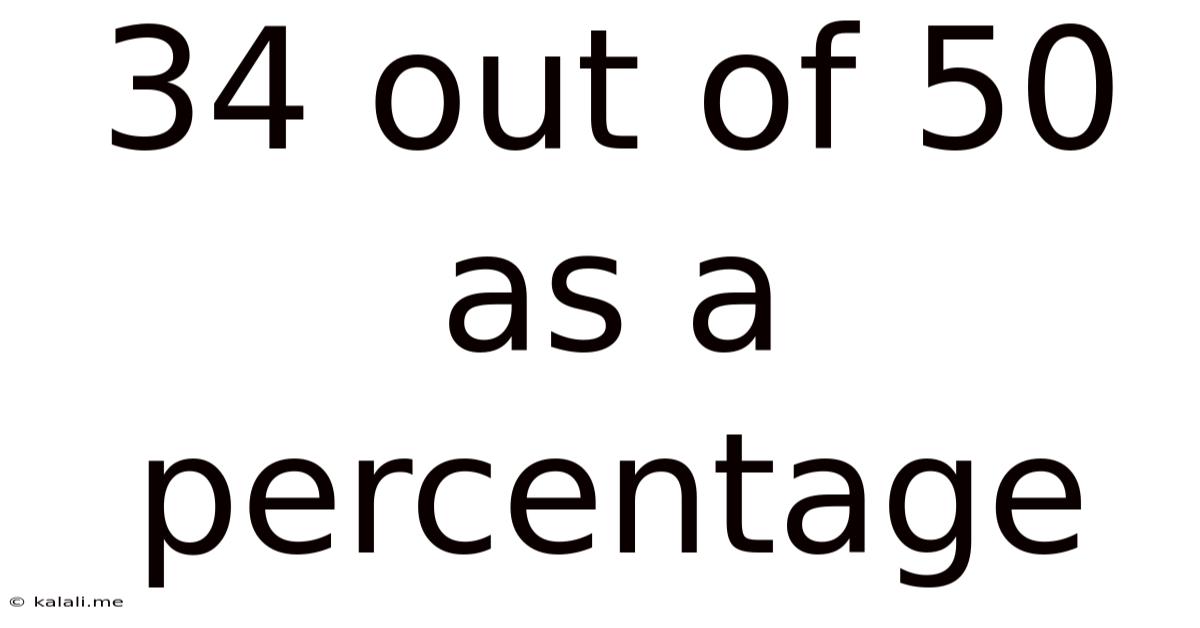
Table of Contents
34 out of 50 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from academic assessments to financial analysis and everyday life situations. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and effectively communicating quantitative information. This article will thoroughly explore how to calculate 34 out of 50 as a percentage, delve into the underlying principles, and provide practical examples and applications. We'll also cover some common mistakes to avoid and explore related percentage calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of 100" (per centum in Latin). This means that 50% represents 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5 as a decimal. Percentages are a convenient way to compare proportions and represent relative quantities.
Calculating 34 out of 50 as a Percentage
To calculate 34 out of 50 as a percentage, we follow these simple steps:
1. Express the Values as a Fraction:
The first step is to write the given numbers as a fraction. In this case, 34 out of 50 can be written as 34/50.
2. Convert the Fraction to a Decimal:
To convert the fraction to a decimal, divide the numerator (34) by the denominator (50):
34 ÷ 50 = 0.68
3. Convert the Decimal to a Percentage:
To convert the decimal to a percentage, multiply the decimal by 100 and add the percent symbol (%):
0.68 × 100 = 68%
Therefore, 34 out of 50 is equal to 68%.
Alternative Method: Using Proportions
Another way to calculate percentages is using proportions. We can set up a proportion to solve for the percentage:
34/50 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we can cross-multiply:
50x = 3400
x = 3400 ÷ 50
x = 68
Therefore, 34 out of 50 is 68%. This method reinforces the fundamental concept of percentages as a proportion out of 100.
Practical Applications and Examples
The ability to calculate percentages has numerous real-world applications. Here are a few examples:
1. Academic Performance:
Imagine a student scores 34 out of 50 on a test. Knowing that this translates to 68%, the student, teacher, and parents can easily understand their performance relative to the total marks. This allows for accurate assessment and identification of areas needing improvement.
2. Business and Finance:
In business, percentages are used extensively. For instance, calculating profit margins, sales growth, and market share all involve percentage calculations. A company might report that its sales increased by 68% compared to the previous year, directly utilizing the same principle as our example calculation. Understanding these percentages is key to making informed financial decisions.
3. Everyday Life:
Percentages are ubiquitous in daily life. Discounts in stores (e.g., "68% off!"), tax calculations, interest rates on loans, tip calculations in restaurants, and even the nutritional information on food labels all rely on percentage calculations. Being comfortable with percentages empowers you to navigate these everyday scenarios with ease.
4. Data Analysis and Statistics:
In statistics and data analysis, percentages are used to represent proportions within a larger dataset. For instance, in a survey of 50 people, if 34 responded positively to a certain question, the 68% figure provides a concise and easily understandable summary of the results. This allows for straightforward comparison and interpretation of data.
5. Scientific Research:
In various scientific fields, percentages are used to report experimental results, such as the success rate of a treatment, the percentage of a certain compound in a sample, or the percentage of participants who experienced a specific outcome in a clinical trial. The accuracy and precision in reporting percentages are crucial for the reliability and validity of the scientific findings.
Common Mistakes to Avoid
While calculating percentages is straightforward, some common mistakes can lead to incorrect results:
-
Incorrect Fraction Formation: Ensuring the correct fraction (34/50 in this case) is crucial. Reversing the numbers would lead to a completely different and inaccurate result.
-
Errors in Decimal Conversion: Accuracy in dividing the numerator by the denominator is essential. A simple calculation mistake can drastically alter the final percentage.
-
Forgetting to Multiply by 100: This step is fundamental in converting the decimal to a percentage. Omitting this step will result in an answer that is 100 times too small.
-
Misinterpreting the Percentage: Understanding what the percentage represents within the context is critical. A 68% score on a test has a different meaning than a 68% discount on an item.
Extending the Calculation: Related Percentages
Based on our understanding that 34 out of 50 is 68%, we can also easily calculate the percentage of the remaining portion:
The remaining portion is 50 - 34 = 16
To calculate the percentage of the remaining portion, we use the same method:
16/50 = 0.32
0.32 * 100 = 32%
Therefore, the remaining portion represents 32%. Notice that 68% + 32% = 100%, as expected. This demonstrates the complementary nature of percentages within a whole.
Conclusion: Mastering Percentage Calculations
The ability to confidently calculate percentages is a vital skill with broad applicability. Understanding the underlying principles, employing the correct methods (fraction to decimal to percentage or proportions), and avoiding common mistakes are key to achieving accurate results. From academic success to financial literacy and informed decision-making in daily life, mastering percentage calculations empowers you to better understand and interact with the quantitative world around you. This comprehensive guide has provided a detailed exploration of calculating 34 out of 50 as a percentage, along with practical examples and considerations to aid in your understanding and application of this essential mathematical skill. The ability to perform these calculations efficiently and accurately will undoubtedly enhance your problem-solving abilities and contribute to a better grasp of quantitative information across diverse contexts.
Latest Posts
Latest Posts
-
What Does We We Mean In French
Jul 06, 2025
-
What Is Three Score Cubits In Feet
Jul 06, 2025
-
What Is 1 2 Of 1 5
Jul 06, 2025
-
Words To Somewhere Over The Rainbow Judy Garland
Jul 06, 2025
-
How Old Am I If Born In 1986
Jul 06, 2025
Related Post
Thank you for visiting our website which covers about 34 Out Of 50 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.