4 1 2 As A Percent
Kalali
Mar 23, 2025 · 4 min read
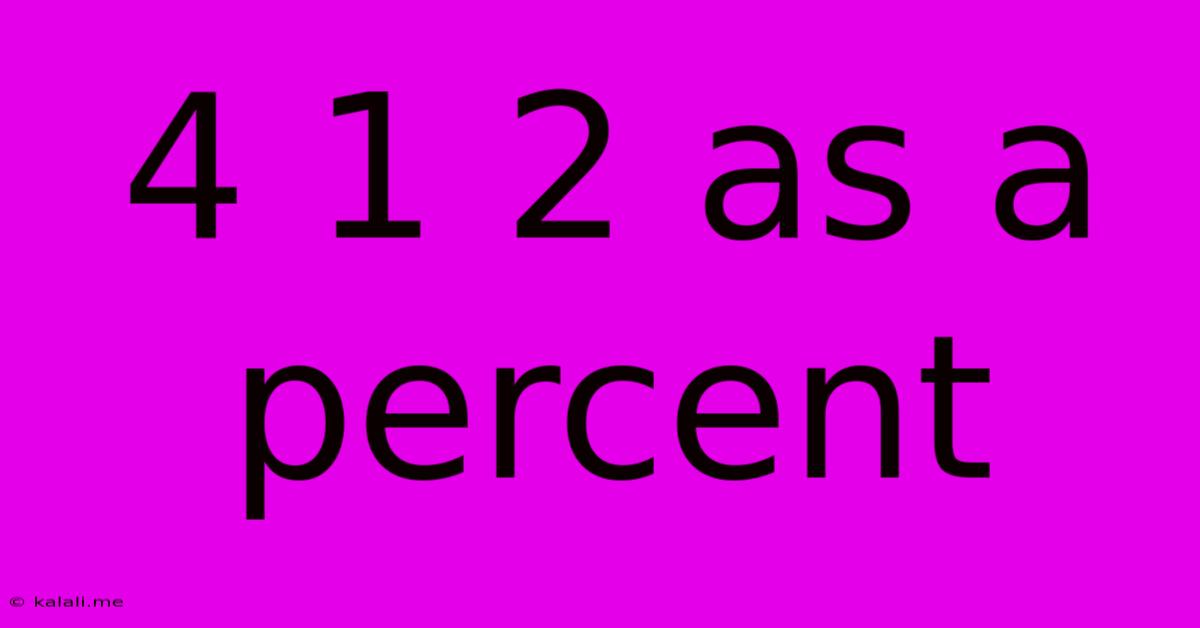
Table of Contents
4/1/2 as a Percent: A Comprehensive Guide to Fraction-to-Percentage Conversion
Understanding how to convert fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields. This article delves deep into converting the mixed fraction 4 1/2 into a percentage, explaining the process step-by-step and providing context to solidify your understanding. We will explore different methods, highlight common pitfalls, and offer practical examples to ensure you master this crucial concept.
Understanding Fractions and Percentages
Before diving into the conversion of 4 1/2, let's refresh our understanding of fractions and percentages.
Fractions: A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts we have, while the denominator indicates how many equal parts the whole is divided into. For instance, 1/2 represents one out of two equal parts.
Percentages: A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or 1/2.
Converting 4 1/2 to an Improper Fraction
The first step in converting 4 1/2 to a percentage involves transforming the mixed fraction into an improper fraction. A mixed fraction combines a whole number and a fraction. To convert it, we follow these steps:
- Multiply the whole number by the denominator: 4 * 2 = 8
- Add the numerator to the result: 8 + 1 = 9
- Keep the same denominator: The denominator remains 2.
Therefore, 4 1/2 as an improper fraction is 9/2.
Converting the Improper Fraction to a Decimal
The next step is to convert the improper fraction 9/2 into a decimal. This is achieved by dividing the numerator by the denominator:
9 ÷ 2 = 4.5
So, 9/2 is equal to 4.5.
Converting the Decimal to a Percentage
Finally, we convert the decimal 4.5 into a percentage. To do this, we multiply the decimal by 100 and add the percentage symbol (%):
4.5 * 100 = 450
Therefore, 4 1/2 is equal to 450%.
Alternative Method: Converting Directly from Mixed Fraction to Percentage
While the above method is straightforward, we can also convert a mixed fraction directly to a percentage. Here's how:
- Convert the fraction part to a decimal: 1/2 = 0.5
- Add the whole number: 4 + 0.5 = 4.5
- Multiply by 100 and add the % symbol: 4.5 * 100 = 450%
This method offers a slightly quicker route to the same result.
Understanding the Significance of 450%
A percentage greater than 100% indicates that the value exceeds the original whole. In the case of 4 1/2 representing 450%, it signifies that we have 4.5 times the original whole. This is commonly encountered in situations like:
- Percentage Increase: If a quantity increases by 450%, it means it has become 4.5 times its original size. For instance, if a company's profits increased by 450%, its new profit is 4.5 times the original profit.
- Growth Rates: In finance and economics, percentages exceeding 100% frequently represent substantial growth rates.
- Comparisons: Using percentages greater than 100% allows for easier comparison of values significantly larger than the reference point.
Practical Applications and Examples
The conversion of fractions to percentages is essential in numerous real-world scenarios:
- Calculating Grades: A student scoring 4.5 out of 2 on a test would have a score of 450%. While unusual, this highlights the mathematical concept. More realistically, a score of 9 out of 20 could be expressed as 45%.
- Financial Calculations: Interest rates, investment returns, and profit margins are often expressed as percentages.
- Data Analysis: Percentages are vital in summarizing and interpreting data, enabling comparisons and the drawing of meaningful conclusions.
- Sales and Discounts: Understanding percentage changes is crucial when calculating discounts, sales tax, or profit margins.
Common Mistakes and How to Avoid Them
Several common mistakes can arise during fraction-to-percentage conversions:
- Incorrect order of operations: Always follow the correct order of operations (PEMDAS/BODMAS) when dealing with mixed fractions.
- Forgetting to multiply by 100: A common error is neglecting to multiply the decimal by 100 to convert it into a percentage.
- Misinterpreting percentages over 100%: Understanding the significance of percentages greater than 100% is crucial for accurate interpretation.
Conclusion
Converting 4 1/2 to a percentage, resulting in 450%, demonstrates a fundamental mathematical concept with broad applications. Mastering this conversion process is crucial for navigating various aspects of daily life, academic studies, and professional endeavors. By understanding the steps involved and avoiding common pitfalls, you can confidently handle fraction-to-percentage conversions with accuracy and efficiency. Remember to practice regularly to solidify your understanding and build your mathematical fluency. The ability to effortlessly convert fractions to percentages is a valuable asset in various fields, empowering you to analyze data, solve problems, and make informed decisions. This comprehensive guide aims to equip you with the necessary skills and knowledge to achieve this mastery.
Latest Posts
Latest Posts
-
How Long Is 13 Miles In Minutes
Jul 03, 2025
-
What Is Half Of 1 1 2
Jul 03, 2025
-
How Much Do A Sandwich Bag Weigh
Jul 03, 2025
-
Why Did The Obtuse Angle Go To The Beach
Jul 03, 2025
-
How Much Is Three Quarts Of Water
Jul 03, 2025
Related Post
Thank you for visiting our website which covers about 4 1 2 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.