43 Out Of 80 As A Percentage
Kalali
Mar 09, 2025 · 5 min read
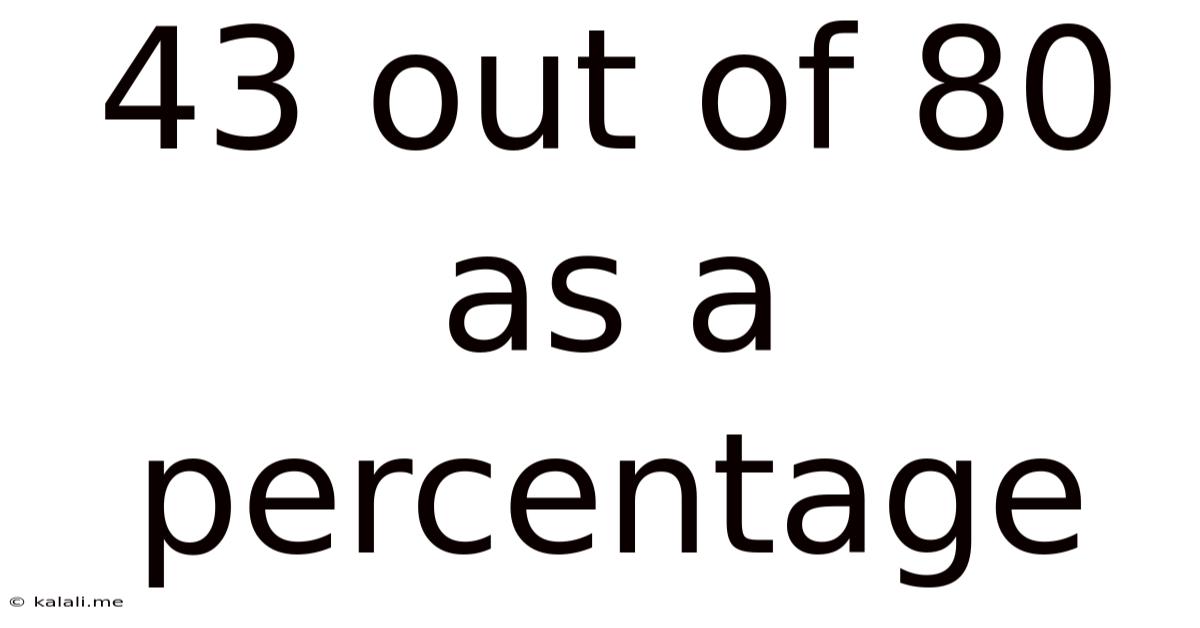
Table of Contents
43 out of 80 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill with wide-ranging applications, from everyday budgeting and shopping to complex statistical analysis and scientific research. Understanding how to express fractions as percentages is crucial for making informed decisions and interpreting data effectively. This comprehensive guide will delve into the calculation of 43 out of 80 as a percentage, providing multiple methods and exploring the broader context of percentage calculations.
Understanding Percentages
Before we dive into the specific calculation, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The word "percent" itself originates from the Latin "per centum," meaning "out of a hundred." Therefore, any percentage can be easily converted into a fraction by placing the percentage value over 100. For example, 25% is equivalent to 25/100, which simplifies to 1/4.
Method 1: Using the Basic Percentage Formula
The most straightforward method for calculating 43 out of 80 as a percentage involves using the fundamental percentage formula:
(Part / Whole) * 100% = Percentage
In this case:
- Part: 43
- Whole: 80
Substituting these values into the formula, we get:
(43 / 80) * 100% = 53.75%
Therefore, 43 out of 80 is 53.75%.
Method 2: Converting to a Decimal First
An alternative approach involves first converting the fraction 43/80 into a decimal and then multiplying by 100% to obtain the percentage. Dividing 43 by 80 using a calculator gives us 0.5375. Multiplying this decimal by 100% yields:
0.5375 * 100% = 53.75%
This method provides the same result, confirming our previous calculation. It's often preferable when dealing with larger numbers or more complex fractions.
Method 3: Using Proportions
The concept of proportions can also be employed to solve this problem. A proportion is a statement that two ratios are equal. We can set up a proportion to find the percentage:
43/80 = x/100
Here, 'x' represents the percentage we want to find. To solve for 'x', we can cross-multiply:
43 * 100 = 80 * x
4300 = 80x
x = 4300 / 80
x = 53.75
Therefore, 43 out of 80 is 53.75%. This method demonstrates the equivalence between fractions and percentages in a clear and logical manner.
Practical Applications: Real-World Examples
The ability to quickly and accurately calculate percentages has numerous real-world applications. Consider these scenarios:
1. Academic Performance:
Imagine a student scoring 43 out of 80 marks on a test. Understanding that this equates to 53.75% allows both the student and the teacher to easily gauge performance relative to the total possible marks. This percentage can be compared to other students' scores and used to assess overall class performance.
2. Sales and Discounts:
Retailers frequently use percentages to express discounts. If a store offers a 53.75% discount on an item, knowing this percentage helps consumers quickly determine the final price. Conversely, businesses use this calculation to understand the impact of discounts on their profit margins.
3. Financial Calculations:
Percentage calculations are fundamental in finance. Calculating interest rates, returns on investment, tax rates, and profit margins all rely heavily on understanding percentages. For instance, an investment that yields a return equivalent to 53.75% represents a significant gain.
4. Statistical Analysis:
In statistical analysis, percentages are essential for presenting and interpreting data. Researchers use percentages to represent proportions of a population or sample that possess a particular characteristic. For example, a survey might reveal that 53.75% of respondents prefer a specific product.
Beyond the Calculation: Understanding the Context
While the calculation of 43 out of 80 as 53.75% is straightforward, understanding the context in which this percentage is presented is crucial. For instance, in an academic setting, a score of 53.75% might be considered passing or failing depending on the grading system. In a sales context, a 53.75% discount is a substantial reduction, but the absolute value of the discount would depend on the initial price of the item.
Rounding and Significant Figures
In many practical applications, rounding the percentage to a more manageable number is acceptable. For example, 53.75% could be rounded to 54%. The level of precision required depends on the context. In scientific research, significant figures are crucial for maintaining accuracy.
Using Technology for Percentage Calculations
Modern technology simplifies percentage calculations immensely. Calculators, spreadsheets (like Microsoft Excel or Google Sheets), and even many smartphones have built-in functions to perform these calculations effortlessly. Spreadsheets, in particular, are incredibly powerful tools for handling large datasets and performing complex percentage-based calculations efficiently.
Troubleshooting Common Errors
While percentage calculations are relatively straightforward, several common errors can occur:
- Incorrect Formula: Using the wrong formula is a frequent mistake. Always double-check that you are using the correct formula: (Part / Whole) * 100%.
- Decimal Errors: Errors can arise during decimal calculations. Ensure your calculations are accurate and use a calculator when necessary.
- Misinterpretation of the Question: Carefully read and interpret the problem to ensure you are calculating the correct percentage. Understanding the context is vital.
- Rounding Errors: While rounding is sometimes necessary, be mindful of the level of precision required and avoid excessive rounding that might lead to significant inaccuracies.
Advanced Percentage Calculations
While this article focused on a single percentage calculation, the principles can be extended to more complex scenarios. These might include:
- Calculating percentage increase or decrease: This involves finding the percentage change between two values.
- Finding the original value after a percentage change: This involves working backward from a percentage change to determine the starting value.
- Calculating compound interest: This involves calculating interest on both the principal and accumulated interest over multiple periods.
- Applying multiple percentage changes: This requires a careful understanding of order of operations and the impact of successive percentage changes.
Conclusion
Calculating 43 out of 80 as a percentage – resulting in 53.75% – is a fundamental skill with broad applications. Mastering this skill, along with a clear understanding of the underlying concepts and potential pitfalls, empowers individuals to confidently navigate various real-world situations requiring percentage calculations. From academic assessments to financial decisions and statistical analysis, understanding percentages is essential for informed decision-making and effective communication of quantitative data. Remember to always double-check your work and consider the context when interpreting the results.
Latest Posts
Latest Posts
-
How Many Ounces In A Pint Of Berries
Jul 03, 2025
-
How Much Is A Peck Of Tomatoes
Jul 03, 2025
-
How Long Is 13 Miles In Minutes
Jul 03, 2025
-
What Is Half Of 1 1 2
Jul 03, 2025
-
How Much Do A Sandwich Bag Weigh
Jul 03, 2025
Related Post
Thank you for visiting our website which covers about 43 Out Of 80 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.