47 6 As A Mixed Number
Kalali
Apr 03, 2025 · 5 min read
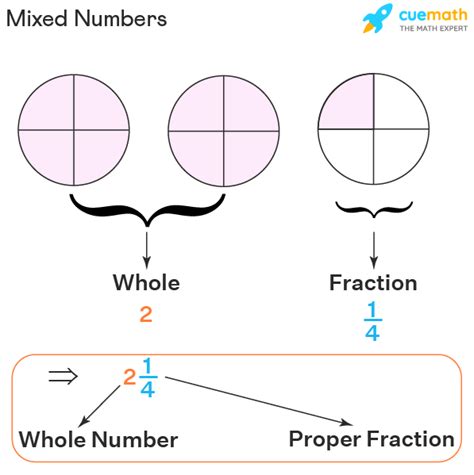
Table of Contents
47/6 as a Mixed Number: A Comprehensive Guide
Converting improper fractions to mixed numbers is a fundamental skill in arithmetic. This comprehensive guide delves deep into understanding how to convert the improper fraction 47/6 into its mixed number equivalent. We will explore the process step-by-step, providing clear explanations and examples to solidify your understanding. We’ll also examine the underlying concepts and offer practical applications to enhance your proficiency.
Understanding Improper Fractions and Mixed Numbers
Before diving into the conversion of 47/6, let's clarify the definitions of improper fractions and mixed numbers.
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). In simpler terms, it represents a value greater than or equal to one. Examples include 7/4, 11/5, and of course, our focus today, 47/6.
Mixed Number: A mixed number combines a whole number and a proper fraction. A proper fraction has a numerator smaller than its denominator. Examples include 1 1/2, 3 2/5, and the result we'll obtain from 47/6.
Converting 47/6 to a Mixed Number: The Step-by-Step Process
The conversion of an improper fraction to a mixed number involves division. Here’s how to convert 47/6:
-
Divide the numerator by the denominator: We divide 47 by 6.
- 47 ÷ 6 = 7 with a remainder of 5
-
The quotient becomes the whole number: The result of the division (7) becomes the whole number part of our mixed number.
-
The remainder becomes the numerator of the fraction: The remainder (5) becomes the numerator of the fractional part of our mixed number.
-
The denominator remains the same: The denominator of the improper fraction (6) remains unchanged in the mixed number.
Therefore, 47/6 as a mixed number is 7 5/6.
Visualizing the Conversion
Imagine you have 47 identical objects, and you want to group them into sets of 6. You can form 7 complete sets of 6, with 5 objects remaining. This perfectly illustrates the mixed number 7 5/6. Each complete set represents a whole number (7), and the remaining 5 objects represent the fractional part (5/6).
Practical Applications of Mixed Numbers
Mixed numbers are incredibly useful in various real-world scenarios:
-
Measurement: Imagine you're measuring the length of a piece of wood. You might find that it's 7 and 5/6 inches long. Using a mixed number makes the measurement much clearer than expressing it as 47/6 inches.
-
Cooking and Baking: Recipes often use mixed numbers to specify ingredient quantities, such as 2 1/2 cups of flour or 1 3/4 teaspoons of baking powder.
-
Time: We frequently use mixed numbers to represent durations. For instance, you might spend 1 1/2 hours studying or 2 3/4 hours sleeping.
-
Construction and Engineering: Mixed numbers are frequently used in calculations involving dimensions and measurements.
-
Everyday Fractions: In daily life, you'll encounter situations that require understanding fractions. Knowing how to convert between improper fractions and mixed numbers simplifies these scenarios.
Further Exploration: Equivalent Fractions
Understanding equivalent fractions strengthens your grasp of fractions in general. Equivalent fractions are fractions that represent the same value, even though their numerators and denominators differ.
For example, 47/6 is equivalent to fractions like 94/12, 141/18, and so on. These equivalent fractions all reduce back to 47/6. This concept can be valuable when working with more complex fraction problems.
Converting Mixed Numbers Back to Improper Fractions
The reverse process is equally important. To convert a mixed number back to an improper fraction:
-
Multiply the whole number by the denominator: Multiply the whole number (7) by the denominator (6): 7 * 6 = 42.
-
Add the numerator: Add the result (42) to the numerator (5): 42 + 5 = 47.
-
Keep the denominator: The denominator remains the same (6).
Therefore, 7 5/6 converts back to 47/6, confirming our original conversion.
Working with Mixed Numbers in Calculations
Adding, subtracting, multiplying, and dividing mixed numbers requires a clear understanding of the process. For addition and subtraction, it's often simpler to convert the mixed numbers to improper fractions, perform the calculation, and then convert the result back to a mixed number. For multiplication and division, you have the option to either convert to improper fractions first or to work directly with the mixed numbers; often converting first simplifies the calculations.
Let's illustrate with addition:
Example: Add 2 1/3 and 1 2/3.
-
Convert to improper fractions: 2 1/3 = 7/3 and 1 2/3 = 5/3.
-
Add the improper fractions: 7/3 + 5/3 = 12/3.
-
Simplify and convert back to a mixed number: 12/3 = 4.
Therefore, 2 1/3 + 1 2/3 = 4.
Advanced Applications and Problem Solving
The conversion between improper fractions and mixed numbers forms the basis for more advanced mathematical concepts, including:
-
Algebra: Solving equations involving fractions often necessitates the conversion between these forms.
-
Calculus: Understanding fractions is essential in the field of calculus, especially when dealing with limits and derivatives.
-
Geometry and Trigonometry: Various geometric and trigonometric calculations involve fractional values, often requiring conversion between improper fractions and mixed numbers.
Conclusion: Mastering the Conversion of 47/6
Converting 47/6 to the mixed number 7 5/6 is a straightforward process, yet it forms a crucial building block in many mathematical applications. By understanding the underlying principles and practicing the steps, you'll develop a strong foundation in fraction manipulation, enabling you to tackle more complex mathematical problems with confidence. Remember the visualization techniques and practice converting mixed numbers back to improper fractions to solidify your understanding. The ability to confidently work with fractions is a valuable skill in various aspects of life, from everyday calculations to advanced mathematical pursuits. So, embrace this fundamental concept and enjoy the journey of mastering fractions!
Latest Posts
Latest Posts
-
What Is 16 20 As A Percent
Apr 04, 2025
-
22 Is What Percent Of 40
Apr 04, 2025
-
Is Length An Intensive Or Extensive Property
Apr 04, 2025
-
14 Of 25 Is What Percent
Apr 04, 2025
-
Which Of The Following Are Renewable Resources
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 47 6 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
