5 Of 20 Is What Percent
Kalali
Apr 14, 2025 · 6 min read
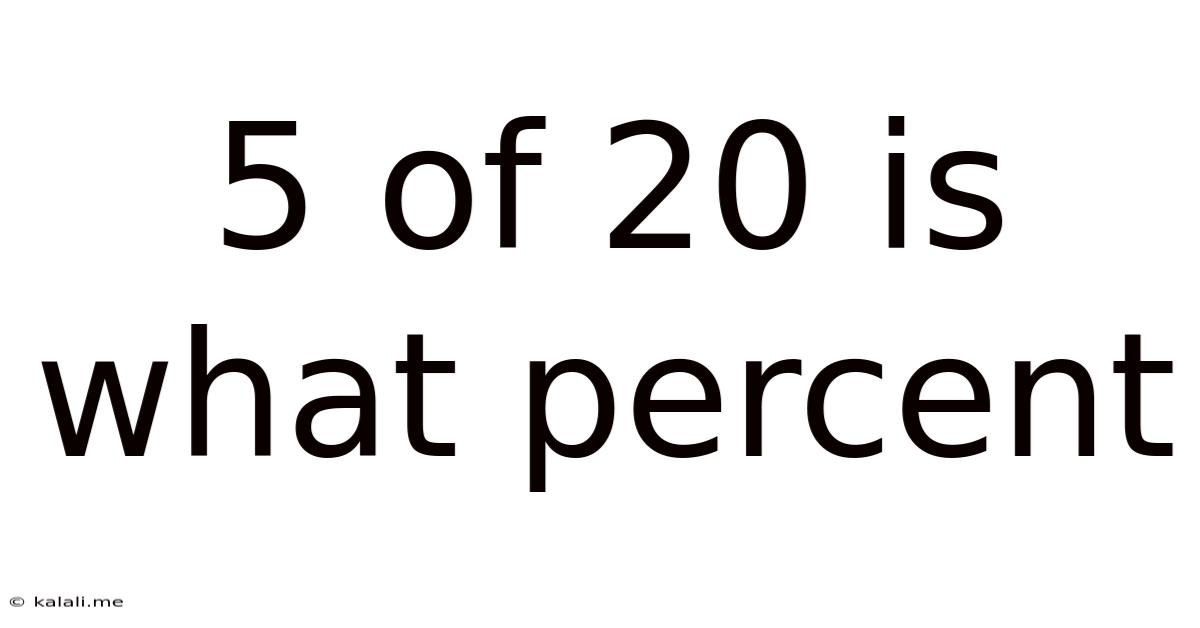
Table of Contents
5 out of 20 is What Percent? A Deep Dive into Percentage Calculations and Their Applications
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday finances to complex scientific calculations. This article will explore how to calculate the percentage represented by 5 out of 20, delve into the underlying principles of percentage calculations, and illustrate their practical applications in various scenarios. We'll also touch upon related concepts and offer further resources for those seeking a deeper understanding of this essential mathematical concept. This in-depth guide will equip you with the knowledge and tools to confidently tackle percentage problems in any context.
Meta Description: Learn how to calculate percentages with a step-by-step guide solving "5 out of 20 is what percent?". Explore various methods, practical applications, and related percentage concepts. Master percentage calculations and improve your mathematical skills.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred." Therefore, 25% means 25 out of 100, which can be written as the fraction 25/100 or the decimal 0.25. Percentages are used to represent proportions, ratios, and rates of change in a readily understandable format. They are ubiquitous in everyday life, appearing on price tags, in financial reports, and in countless other contexts.
Calculating 5 out of 20 as a Percentage: The Simple Method
The most straightforward way to determine what percentage 5 out of 20 represents involves the following steps:
-
Form a Fraction: Express the problem as a fraction: 5/20. This represents 5 parts out of a total of 20 parts.
-
Convert to a Decimal: Divide the numerator (5) by the denominator (20): 5 ÷ 20 = 0.25
-
Convert to a Percentage: Multiply the decimal by 100: 0.25 x 100 = 25
Therefore, 5 out of 20 is 25%.
Calculating 5 out of 20 as a Percentage: The Proportion Method
Another method, particularly useful for more complex problems, involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can set up a proportion to solve for the unknown percentage (x):
5/20 = x/100
To solve for x, we cross-multiply:
20x = 500
Then, divide both sides by 20:
x = 500/20 = 25
Therefore, once again, we find that 5 out of 20 is 25%. This method emphasizes the underlying relationship between fractions and percentages.
Beyond the Basics: Understanding Percentage Increase and Decrease
While the problem "5 out of 20 is what percent?" focuses on a static proportion, percentages are frequently used to represent changes. Let's explore percentage increase and decrease:
-
Percentage Increase: This calculates the increase in a value as a percentage of the original value. For example, if a price increases from $100 to $120, the percentage increase is calculated as follows:
- Find the difference: $120 - $100 = $20
- Divide the difference by the original value: $20 / $100 = 0.2
- Multiply by 100 to express as a percentage: 0.2 x 100 = 20%
Therefore, the price increased by 20%.
-
Percentage Decrease: This calculates the decrease in a value as a percentage of the original value. For instance, if a price decreases from $100 to $80, the percentage decrease is:
- Find the difference: $100 - $80 = $20
- Divide the difference by the original value: $20 / $100 = 0.2
- Multiply by 100 to express as a percentage: 0.2 x 100 = 20%
Therefore, the price decreased by 20%.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in numerous real-world scenarios:
-
Finance: Calculating interest rates, discounts, taxes, tips, and investment returns all rely heavily on percentage calculations. Understanding percentage changes in stock prices, for example, is critical for investors. Compound interest calculations, a key component of financial planning, rely heavily on percentage computations.
-
Retail: Stores use percentages to offer discounts (e.g., 20% off), calculate sales tax, and analyze profit margins. Understanding markups and markdowns is crucial for business owners and consumers alike.
-
Science: Percentages are frequently used to represent data in scientific experiments, such as the percentage of a solution, the percentage error in measurements, or the percentage change in a biological population.
-
Education: Grading systems often use percentages to represent student performance on tests and assignments. Calculating grade point averages (GPAs) also involves percentage-based calculations.
-
Everyday Life: Calculating the tip at a restaurant, figuring out the sale price of an item, or understanding statistics presented in news reports all require an understanding of percentages.
Advanced Percentage Calculations and Related Concepts
Beyond basic percentage calculations, several more advanced concepts are important to understand:
-
Compound Interest: This is interest calculated on the principal amount plus accumulated interest. It's a powerful concept used in savings accounts and loans.
-
Percentage Points: These represent the actual difference between two percentages, not the percentage change. For example, if interest rates increase from 5% to 8%, this is a 3 percentage point increase, not a 60% increase.
-
Percentage Change vs. Percentage Point Change: It's crucial to distinguish between these two concepts. A percentage change shows the relative change, while a percentage point change shows the absolute difference.
-
Ratio and Proportion: Percentages are directly linked to ratios and proportions, and understanding these concepts enhances problem-solving abilities.
Tips for Mastering Percentage Calculations
-
Practice Regularly: The best way to master percentages is through consistent practice. Solve various problems, including those involving percentage increases, decreases, and compound interest.
-
Use Different Methods: Try different methods for solving percentage problems to develop a deep understanding of the underlying principles.
-
Check Your Work: Always double-check your calculations to ensure accuracy.
-
Utilize Online Resources: Numerous online calculators and tutorials can help you practice and improve your understanding of percentage calculations.
-
Break Down Complex Problems: If you encounter a complex percentage problem, break it down into smaller, more manageable steps.
Conclusion: The Importance of Percentage Proficiency
The ability to calculate and interpret percentages is a highly valuable skill applicable across a wide range of contexts. From everyday finances to complex scientific analysis, understanding percentages is crucial for informed decision-making and problem-solving. Mastering this fundamental mathematical concept will significantly enhance your analytical skills and provide a valuable tool for navigating the numerical world. By understanding the principles outlined in this article and practicing regularly, you'll build a strong foundation for tackling percentage-related challenges with confidence. Remember that the core concept remains consistent—understanding the relationship between a part and a whole, expressed as a fraction of 100. Whether it’s calculating a simple proportion like "5 out of 20 is what percent?" or tackling more complex problems involving percentage changes and compound interest, the principles remain the same, making the mastery of this concept well worth the effort.
Latest Posts
Latest Posts
-
What Is 27 Out Of 30 As A Percentage
Apr 15, 2025
-
Select All Correct Features Of Reptile Reproduction
Apr 15, 2025
-
What Is 18 As A Fraction
Apr 15, 2025
-
Cuanto Es 8 Metros En Pies
Apr 15, 2025
-
Which Is A Gas At Room Temperature Sodium
Apr 15, 2025
Related Post
Thank you for visiting our website which covers about 5 Of 20 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.