60 Is What Percent Of 500
Kalali
Mar 31, 2025 · 4 min read
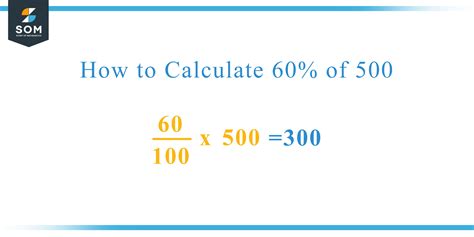
Table of Contents
60 is What Percent of 500? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday budgeting to complex financial analysis. This article will thoroughly explore how to calculate what percentage 60 represents of 500, providing a step-by-step guide and delving into the broader context of percentage calculations. We'll also explore practical applications and related concepts to solidify your understanding.
Understanding the Fundamentals of Percentages
Before diving into the specific calculation, let's establish a firm grasp of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" denotes a percentage. For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5 in decimal form.
Key Terms and Concepts:
- Percentage: A portion or share of a whole, expressed as a number out of 100.
- Base Value: The whole amount or the total value that a percentage is calculated from. In our case, this is 500.
- Part Value: The portion of the base value being expressed as a percentage. This is 60 in our example.
- Percentage Rate: The calculated percentage that the part value represents of the base value. This is what we need to find.
Calculating the Percentage: 60 out of 500
There are several methods to calculate what percentage 60 represents of 500. Let's explore the most common and straightforward approaches.
Method 1: Using the Formula
The most fundamental method involves using the basic percentage formula:
(Part Value / Base Value) * 100 = Percentage Rate
Substituting our values:
(60 / 500) * 100 = Percentage Rate
This simplifies to:
0.12 * 100 = 12%
Therefore, 60 is 12% of 500.
Method 2: Using Proportions
We can also approach this problem using proportions. We can set up a proportion to solve for the unknown percentage (x):
60/500 = x/100
To solve for x, we cross-multiply:
60 * 100 = 500 * x
6000 = 500x
Now, divide both sides by 500:
x = 6000 / 500 = 12
Therefore, x = 12%, confirming our previous result.
Method 3: Using Decimal Conversion
We can convert the fraction 60/500 into a decimal and then multiply by 100 to obtain the percentage.
60/500 = 0.12
0.12 * 100 = 12%
This method provides a straightforward way to calculate the percentage, especially when dealing with simpler fractions.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world scenarios. Here are some examples:
1. Financial Calculations:
- Interest rates: Calculating simple or compound interest on loans or investments.
- Discounts: Determining the final price of an item after a percentage discount.
- Taxes: Calculating sales tax, income tax, or other taxes based on a percentage.
- Profit margins: Analyzing the profitability of a business by calculating the percentage of profit relative to revenue.
- Investment returns: Assessing the performance of investments by calculating percentage returns.
2. Data Analysis and Statistics:
- Representing data: Visualizing data using charts and graphs that show percentages.
- Calculating probabilities: Expressing the likelihood of an event occurring as a percentage.
- Analyzing survey results: Interpreting survey data by calculating percentages of responses.
3. Everyday Life:
- Tipping: Calculating a tip amount in a restaurant based on a percentage of the bill.
- Sales and promotions: Understanding discounts and offers advertised as percentages.
- Cooking and baking: Adjusting recipes by increasing or decreasing ingredient amounts by a certain percentage.
Expanding Your Understanding: Related Percentage Concepts
To enhance your understanding of percentages, let's explore some related concepts:
1. Percentage Increase and Decrease:
These concepts involve calculating the percentage change between two values. For instance, if a value increases from 100 to 120, the percentage increase is calculated as:
((120 - 100) / 100) * 100 = 20%
Similarly, if a value decreases from 100 to 80, the percentage decrease is:
((100 - 80) / 100) * 100 = 20%
2. Percentage Points:
This term is often misused. Percentage points refer to the absolute difference between two percentages. For example, if interest rates increase from 5% to 8%, the increase is 3 percentage points, not 3%.
3. Percentage of a Percentage:
This involves calculating a percentage of a percentage. For example, finding 10% of 20% would involve multiplying 0.10 by 0.20, resulting in 0.02 or 2%.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages, such as determining that 60 is 12% of 500, is an essential skill applicable in numerous aspects of life. By mastering the different methods presented – using the formula, proportions, or decimal conversion – you can confidently tackle percentage calculations in various contexts. Remember to clearly identify the part value and the base value before applying the appropriate formula. Furthermore, exploring related concepts like percentage increase, decrease, and points will solidify your understanding and allow you to apply this knowledge effectively in both professional and personal settings. Practice regularly, and you'll find that percentage calculations become second nature.
Latest Posts
Latest Posts
-
How Many Feet In 70 Inches
Apr 01, 2025
-
What Is Standard Form Of A Polynomial
Apr 01, 2025
-
El 5 Por Ciento De 1000
Apr 01, 2025
-
How Many Feet Is 110 In
Apr 01, 2025
-
36 7 C To F In Fahrenheit
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about 60 Is What Percent Of 500 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
