What Is Standard Form Of A Polynomial
Kalali
Apr 01, 2025 · 5 min read
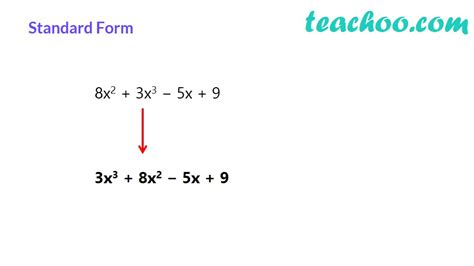
Table of Contents
What is Standard Form of a Polynomial? A Comprehensive Guide
Polynomials are fundamental building blocks in algebra, forming the basis for many mathematical concepts and applications. Understanding their structure, particularly their standard form, is crucial for manipulating and solving algebraic equations. This comprehensive guide will delve into the definition of a polynomial, explore its standard form, and illustrate its significance through various examples and applications. We'll cover different types of polynomials and provide practical tips for converting polynomials into standard form.
Understanding Polynomials: A Definition
A polynomial is an expression consisting of variables (often denoted by x, y, etc.) and coefficients, combined using addition, subtraction, and multiplication, but never division by a variable. The exponents of the variables must be non-negative integers. Each term in a polynomial is a monomial, meaning a single term that is a product of a constant and variables raised to non-negative integer powers.
Key characteristics of polynomials:
- Terms: Individual parts of the polynomial separated by addition or subtraction. For example, in the polynomial 3x² + 2x - 5, the terms are 3x², 2x, and -5.
- Coefficients: The numerical factor of each term. In 3x² + 2x - 5, the coefficients are 3, 2, and -5.
- Variables: The letters representing unknown values (typically x, y, z, etc.).
- Exponents: The powers to which the variables are raised. These must be non-negative integers.
- Degree: The highest power of the variable in the polynomial. For instance, 3x² + 2x - 5 has a degree of 2.
What is Standard Form of a Polynomial?
The standard form of a polynomial arranges the terms in descending order of their exponents. This means the term with the highest exponent is written first, followed by the term with the next highest exponent, and so on, until the constant term (the term with no variable) is last.
Example:
Let's consider the polynomial 2x + 5x³ - 7 + 4x². To write it in standard form, we arrange the terms according to their exponents:
5x³ + 4x² + 2x - 7
In this standard form, the terms are arranged in descending order of their exponents: 3, 2, 1, and 0 (for the constant term).
Types of Polynomials Based on Degree and Number of Terms
Polynomials are further categorized based on their degree and the number of terms they contain:
Based on Degree:
- Constant Polynomial: A polynomial of degree 0 (e.g., 5, -2).
- Linear Polynomial: A polynomial of degree 1 (e.g., 2x + 3, y - 7).
- Quadratic Polynomial: A polynomial of degree 2 (e.g., x² + 2x - 1, 3y² - 5y + 2).
- Cubic Polynomial: A polynomial of degree 3 (e.g., x³ - 4x² + 6x - 1).
- Quartic Polynomial: A polynomial of degree 4 (e.g., x⁴ + 2x³ - x² + 3x - 8).
- Quintic Polynomial: A polynomial of degree 5 (and so on for higher degrees).
Based on Number of Terms:
- Monomial: A polynomial with one term (e.g., 4x², -7y³).
- Binomial: A polynomial with two terms (e.g., 2x + 3, x² - 4).
- Trinomial: A polynomial with three terms (e.g., x² + 2x - 5, y³ - 3y + 7).
The Importance of Standard Form
Writing a polynomial in standard form offers several crucial advantages:
- Easy identification of the degree: The degree of the polynomial is immediately apparent as it's the exponent of the first term.
- Simplified addition and subtraction: Combining like terms becomes straightforward when polynomials are in standard form. Terms with the same exponent can be easily identified and added or subtracted.
- Efficient multiplication and division: Multiplying and dividing polynomials is simplified, especially when using techniques like long division.
- Finding roots (zeros): Standard form facilitates various methods for finding the roots or zeros of the polynomial (values of x that make the polynomial equal to zero).
- Graphing polynomials: The standard form helps in understanding the behavior of the polynomial's graph. The leading term (term with the highest exponent) dictates the end behavior of the graph.
Examples of Converting to Standard Form
Let's practice converting polynomials into standard form:
Example 1:
Convert the polynomial 3x - 5x³ + 2 + 7x² into standard form.
Solution: Arrange the terms in descending order of exponents:
-5x³ + 7x² + 3x + 2
Example 2:
Convert the polynomial 4y⁴ - 2y + 9y² - y³ + 6 into standard form.
Solution: Arrange the terms in descending order of exponents:
4y⁴ - y³ + 9y² - 2y + 6
Example 3: A more complex example
Convert the polynomial x²y³ + 3xy⁴ – 2x³y² + 5x⁴y into standard form. Note that this is a polynomial in two variables, and we will use a lexicographical order which prioritizes the powers of x before y.
Solution:
We prioritize terms with the highest power of x, then consider the powers of y within the terms with same power of x. This gives us the standard form:
5x⁴y – 2x³y² + 3xy⁴ + x²y³
Applications of Polynomials and Standard Form
Polynomials have a wide range of applications across various fields:
- Computer graphics: Polynomials are used to create curves and surfaces in computer-aided design (CAD) and computer graphics.
- Physics and engineering: They are used to model various physical phenomena, such as projectile motion, oscillations, and electrical circuits.
- Economics and finance: Polynomials are employed in modeling economic growth, predicting market trends, and analyzing financial data.
- Data analysis: Polynomial regression is used to fit curves to data sets, allowing for better understanding and prediction of trends.
- Numerical analysis: Polynomials are fundamental tools in numerical methods for solving equations and approximating functions.
Advanced Topics and Further Exploration
Beyond the basics, further exploration of polynomials includes:
- Polynomial factorization: Breaking down a polynomial into simpler factors.
- Polynomial long division: Dividing one polynomial by another.
- Synthetic division: A shorthand method for polynomial division.
- Partial fraction decomposition: Expressing a rational function as a sum of simpler fractions.
- Roots of polynomials and their multiplicity: Understanding the nature and repetition of solutions to polynomial equations.
- Complex roots of polynomials: Exploring solutions involving imaginary numbers.
- The Fundamental Theorem of Algebra: A cornerstone theorem stating that a polynomial of degree n has exactly n roots (counting multiplicity) in the complex numbers.
Conclusion
Understanding the standard form of a polynomial is crucial for effectively working with these algebraic expressions. By arranging terms according to their exponents in descending order, we simplify numerous operations, making calculations easier and providing insights into the polynomial's properties. From basic algebra to advanced applications in science and engineering, mastery of polynomials and their standard form is a cornerstone of mathematical understanding. This guide has provided a comprehensive overview; continued exploration of the advanced topics mentioned above will enhance your proficiency and allow you to tackle more complex polynomial problems.
Latest Posts
Latest Posts
-
185 Cm In Inches And Feet
Apr 03, 2025
-
What Percent Is 11 Out Of 20
Apr 03, 2025
-
A More Sustainable Form Of Managing Grasslands Is
Apr 03, 2025
-
Cuanto Es 10 Centimetros En Pulgadas
Apr 03, 2025
-
How Many Metres In 10 Yards
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Is Standard Form Of A Polynomial . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
