76 Is What Percent Of 80
Kalali
Apr 19, 2025 · 5 min read
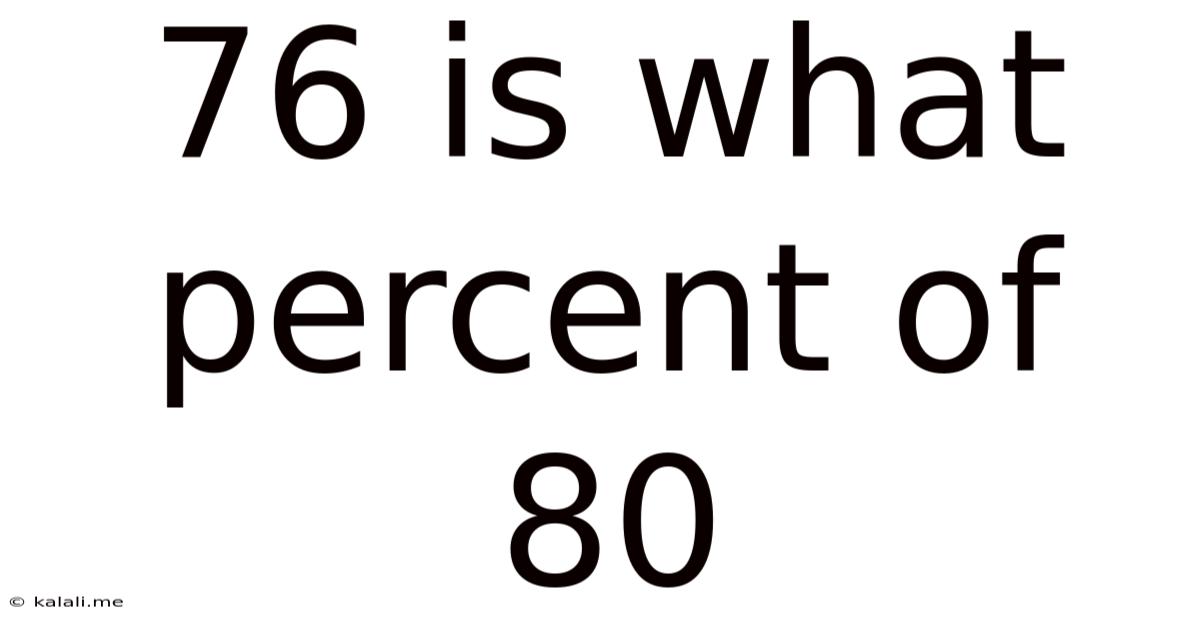
Table of Contents
76 is What Percent of 80? A Comprehensive Guide to Percentage Calculations
This seemingly simple question, "76 is what percent of 80?", opens the door to a deeper understanding of percentage calculations, a fundamental skill in various aspects of life, from finance and shopping to academic pursuits and data analysis. This article will not only answer the question directly but will also explore the underlying principles, provide multiple methods for solving similar problems, and delve into practical applications.
Meta Description: Learn how to calculate percentages with a comprehensive guide. We'll solve "76 is what percent of 80?" and explore different methods, real-world applications, and advanced percentage concepts.
Calculating percentages is a core mathematical skill that finds widespread use in everyday life. Understanding percentages allows us to compare proportions, interpret data, and make informed decisions in a variety of contexts. This article serves as a complete guide, equipping you with the knowledge and techniques to tackle percentage problems with confidence.
Understanding Percentages
Before we tackle the specific problem, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Percentages are incredibly useful because they provide a standardized way to compare different proportions. Whether you're comparing the performance of different stocks, calculating discounts, or assessing the success rate of a marketing campaign, percentages offer a clear and readily understandable representation of the data.
Method 1: Using the Formula
The most straightforward method to solve "76 is what percent of 80?" involves using a fundamental percentage formula:
(Part / Whole) * 100 = Percentage
In our problem:
- Part: 76 (the portion we are considering)
- Whole: 80 (the total amount)
Substituting these values into the formula:
(76 / 80) * 100 = Percentage
This simplifies to:
0.95 * 100 = 95
Therefore, 76 is 95% of 80.
Method 2: Setting up a Proportion
Another effective approach is to set up a proportion. A proportion is an equation stating that two ratios are equal. We can represent the problem as:
76/80 = x/100
Where 'x' represents the percentage we want to find. To solve for x, we cross-multiply:
76 * 100 = 80 * x
7600 = 80x
x = 7600 / 80
x = 95
Again, we find that 76 is 95% of 80.
Method 3: Using Decimal Equivalents
We can also approach this problem by first converting the fraction 76/80 into a decimal and then converting the decimal to a percentage.
76 divided by 80 equals 0.95. To convert a decimal to a percentage, we multiply by 100:
0.95 * 100 = 95%
This confirms our previous results, demonstrating the versatility of different calculation methods.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in many real-world scenarios. Here are a few examples:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all rely heavily on percentage calculations. Understanding percentages is crucial for managing personal finances effectively. For instance, determining the amount of interest accrued on a savings account or the total cost of an item after sales tax involves percentage calculations.
-
Shopping: Sales and discounts are often expressed as percentages. Calculating the final price of an item after a discount requires understanding how to determine a percentage of a number. Similarly, comparing prices and finding the best deal often involves comparing percentages.
-
Data Analysis: Percentages are widely used to represent data in various fields, such as business, science, and education. Analyzing survey results, interpreting statistical data, and comparing performance metrics frequently involves expressing data as percentages.
-
Academic Performance: Grade point averages (GPAs) are often expressed as percentages, providing a standardized measure of academic performance. Understanding percentages is essential for interpreting grades and tracking academic progress.
-
Science and Engineering: Percentages play a critical role in various scientific and engineering applications, such as expressing concentrations, efficiencies, and error margins.
Beyond the Basics: Advanced Percentage Calculations
While the problem "76 is what percent of 80?" provides a foundation for understanding percentage calculations, let's explore some more advanced concepts:
-
Percentage Increase and Decrease: Calculating the percentage increase or decrease between two values requires understanding the difference between the values and then expressing that difference as a percentage of the original value. For example, if a stock price increases from $50 to $60, the percentage increase is calculated as [(60-50)/50] * 100 = 20%.
-
Percentage Points: It's crucial to differentiate between percentage change and percentage points. A percentage point refers to an absolute change in percentage values, not a relative change. For instance, if interest rates rise from 2% to 5%, the increase is 3 percentage points, not a 150% increase.
-
Compound Interest: Compound interest involves earning interest on both the principal amount and the accumulated interest. Understanding how to calculate compound interest requires a grasp of exponential growth and percentage calculations.
-
Percentage of a Percentage: Calculating a percentage of a percentage involves performing multiple percentage calculations sequentially. For example, finding 20% of 15% of 100 requires first finding 15% of 100 (15), and then finding 20% of 15 (3).
Practical Tips for Mastering Percentage Calculations
-
Practice regularly: The key to mastering any mathematical concept is consistent practice. Solve a variety of percentage problems to build your understanding and improve your speed and accuracy.
-
Utilize online resources: Many online resources, including calculators and tutorials, can help you learn and practice percentage calculations.
-
Understand the underlying concepts: Don't just memorize formulas; understand the underlying principles behind percentage calculations. This will help you approach problems more effectively and solve them with greater confidence.
-
Break down complex problems: If you encounter a complex percentage problem, break it down into smaller, more manageable steps. This will make the problem less daunting and increase your chances of success.
In conclusion, the answer to "76 is what percent of 80?" is definitively 95%. However, the true value of this seemingly simple calculation lies in the underlying principles and applications it unlocks. Mastering percentage calculations equips you with a crucial skill applicable across numerous disciplines, empowering you to make informed decisions and navigate various real-world scenarios with confidence. Remember to practice regularly, utilize available resources, and delve into the more advanced concepts to solidify your understanding of percentages and their widespread applications.
Latest Posts
Latest Posts
-
14 Of 20 As A Percentage
Apr 22, 2025
-
What Is 30 Out Of 41
Apr 22, 2025
-
What Is The Reciprocal Of 6
Apr 22, 2025
-
At What Temperature Does Blood Boil
Apr 22, 2025
-
What Is 8 12 As A Percentage
Apr 22, 2025
Related Post
Thank you for visiting our website which covers about 76 Is What Percent Of 80 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.