88 Out Of 150 As A Percentage
Kalali
Apr 06, 2025 · 5 min read
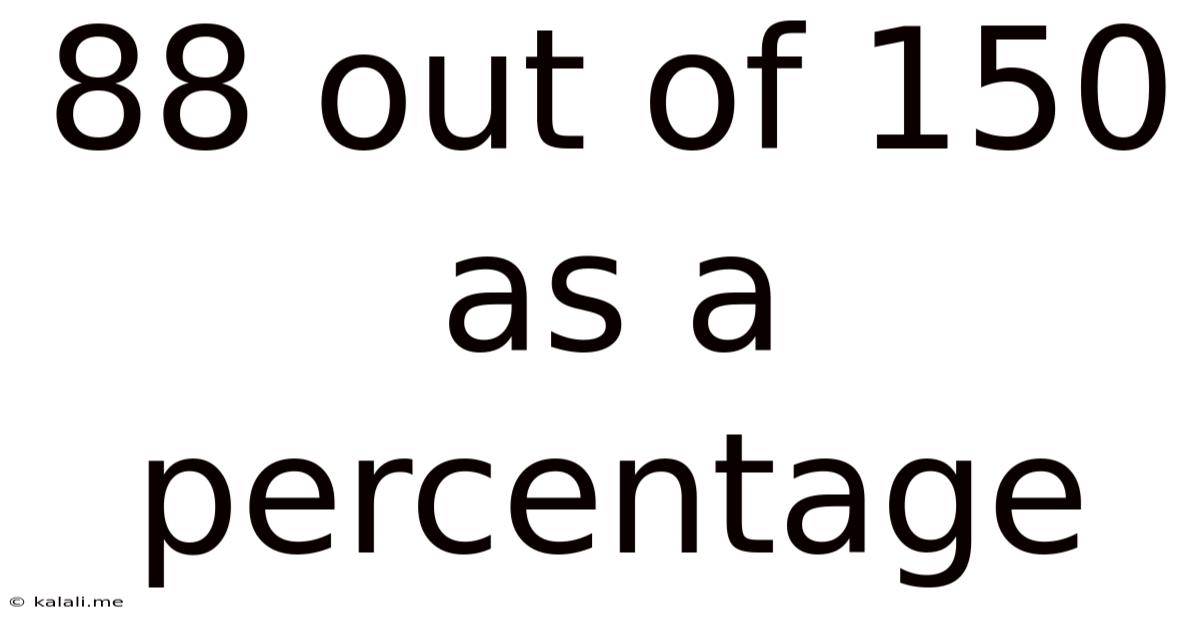
Table of Contents
88 out of 150 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from academic assessments and financial transactions to everyday decision-making. Understanding how to convert fractions and ratios into percentages allows for clearer comparisons and a more intuitive grasp of proportions. This comprehensive guide delves into the specifics of calculating "88 out of 150 as a percentage," providing a detailed explanation of the process and exploring related concepts.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred." For example, 50% means 50 out of 100, which can also be represented as the fraction 50/100 or the decimal 0.5. Percentages provide a standardized way to compare different quantities and proportions.
Calculating 88 out of 150 as a Percentage
To calculate 88 out of 150 as a percentage, we follow these steps:
Step 1: Express the ratio as a fraction.
The ratio "88 out of 150" can be written as the fraction 88/150.
Step 2: Convert the fraction to a decimal.
To convert the fraction to a decimal, divide the numerator (88) by the denominator (150):
88 ÷ 150 = 0.586666...
Step 3: Convert the decimal to a percentage.
To convert a decimal to a percentage, multiply the decimal by 100 and add the "%" symbol:
0.586666... × 100 = 58.6666...%
Step 4: Round the percentage (optional).
Depending on the desired level of precision, you can round the percentage. Rounding to two decimal places, we get:
58.67%
Therefore, 88 out of 150 is 58.67%.
Different Methods for Percentage Calculation
While the above method is straightforward, several other approaches can be used to calculate percentages, each offering different advantages depending on the context and the available tools.
Method 1: Using a Calculator
Most calculators have a percentage function (%) that simplifies the calculation. Simply enter 88 ÷ 150 and then press the % button. The calculator will automatically display the result as a percentage.
Method 2: Using a Spreadsheet Program (like Excel or Google Sheets)
Spreadsheets provide built-in functions for percentage calculations. The formula =88/150*100 in a spreadsheet cell will directly calculate and display the percentage. Formatting the cell as a percentage will automatically add the "%" symbol and handle decimal places.
Method 3: Using Proportions
The problem can also be solved using proportions:
Let x be the percentage we want to find. We can set up the proportion:
88/150 = x/100
To solve for x, cross-multiply:
150x = 8800
x = 8800/150
x = 58.67%
Applications of Percentage Calculations
Understanding percentage calculations is crucial in various fields:
1. Academic Performance:
Calculating grades, percentages of correct answers, and overall academic progress often involves percentage calculations. For instance, if a student scores 88 out of 150 on a test, their score is 58.67%, providing a clear indication of their performance.
2. Financial Matters:
Percentages are fundamental in finance. Calculating interest rates, discounts, taxes, profits, and losses all require understanding percentage calculations. For example, a 10% discount on a $100 item reduces the price to $90.
3. Statistics and Data Analysis:
Percentages are essential for interpreting statistical data. Representing data as percentages makes it easier to compare different datasets and draw meaningful conclusions. For instance, expressing the percentage of respondents who prefer a particular product helps understand consumer preferences.
4. Business and Economics:
Analyzing market share, growth rates, profit margins, and various other business metrics relies heavily on percentage calculations. Understanding these percentages helps businesses make informed decisions.
5. Everyday Life:
Even in daily life, percentages pop up frequently. Calculating tips, sales discounts, and understanding proportions in recipes all involve percentage calculations.
Beyond the Basics: Advanced Percentage Concepts
Understanding the basic calculation of 88 out of 150 as a percentage opens the door to more complex percentage-related problems. Here are some advanced concepts to consider:
1. Percentage Increase and Decrease:
These concepts deal with the change in a value expressed as a percentage of the original value. For instance, if a price increases from $100 to $120, the percentage increase is 20% [(120-100)/100 * 100%]. Conversely, a decrease from $120 to $100 represents a 16.67% decrease [(120-100)/120 * 100%].
2. Percentage Points:
It's crucial to distinguish between percentage change and percentage points. A change from 50% to 60% is a 10 percentage point increase, but a 20% increase relative to the original value.
3. Compound Percentage Growth:
This refers to the growth of a value where the percentage increase is applied to the accumulated value in each period. Compound interest is a prime example of this concept.
4. Percentage of a Percentage:
This involves calculating a percentage of another percentage. For example, finding 20% of 50% involves multiplying 0.20 by 0.50 resulting in 0.10 or 10%.
5. Weighted Averages and Percentages:
In situations with multiple values carrying different weights, weighted averages are necessary. This involves assigning weights to each value and calculating a percentage based on these weights.
Conclusion
Calculating "88 out of 150 as a percentage" is a simple yet important skill. Mastering this fundamental concept opens the door to a wider understanding of percentages and their applications in various aspects of life, from academic achievements to financial decisions and beyond. The methods discussed in this guide provide a solid foundation for approaching percentage problems efficiently and accurately. By understanding the different calculation methods and related concepts, you can confidently tackle more complex percentage-related challenges. Remember to practice regularly to solidify your understanding and improve your proficiency in handling percentages.
Latest Posts
Latest Posts
-
Percentage Of 8 Out Of 12
Apr 09, 2025
-
How Many Liters In 8 Gallons
Apr 09, 2025
-
Whats A 13 Out Of 15
Apr 09, 2025
-
Concave Mirror Focal Length Positive Or Negative
Apr 09, 2025
-
What Is The Percent Of 2 25
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about 88 Out Of 150 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.