9 Out Of 12 As A Percentage
Kalali
Mar 08, 2025 · 5 min read
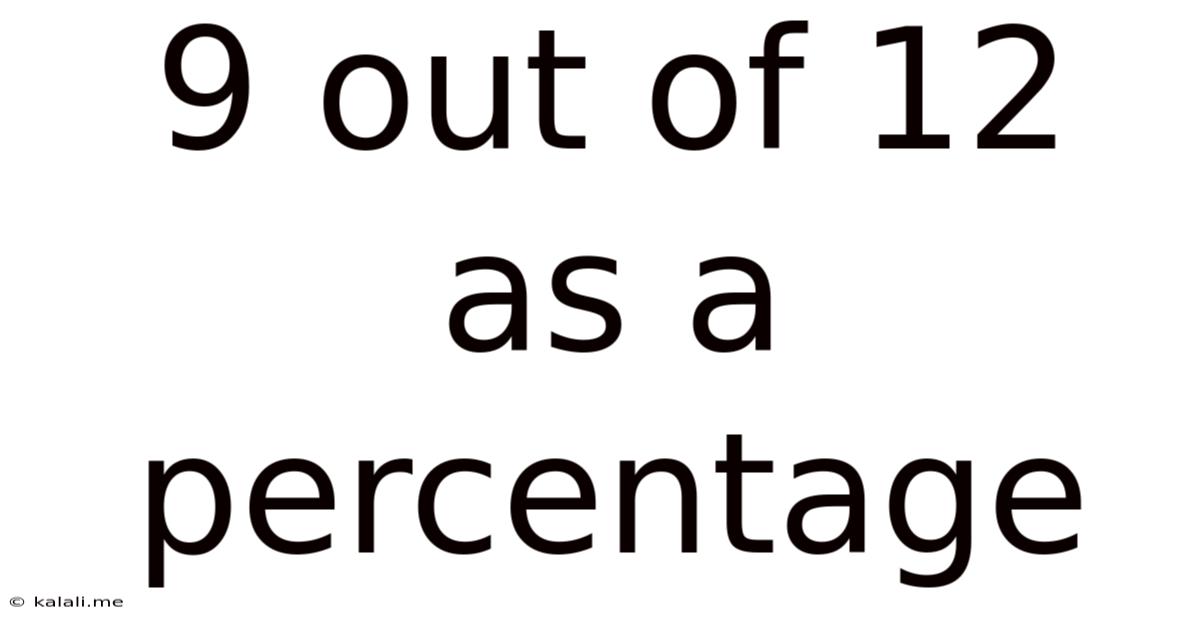
Table of Contents
9 Out of 12 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from academic assessments to financial transactions and everyday decision-making. Understanding how to convert fractions and ratios into percentages allows for clearer comparisons and a more intuitive grasp of proportions. This comprehensive guide will delve into the specific calculation of 9 out of 12 as a percentage, exploring multiple methods and providing context for practical application.
Understanding the Basics of Percentages
Before diving into the specific calculation, let's solidify our understanding of percentages. A percentage is simply a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Key Terminology:
- Numerator: The top number in a fraction (in this case, 9). It represents the part.
- Denominator: The bottom number in a fraction (in this case, 12). It represents the whole.
- Percentage: The fraction expressed as a number out of 100, often represented with the "%" symbol.
Method 1: Using the Fraction Method
This is the most straightforward approach. We begin by expressing "9 out of 12" as a fraction: 9/12.
-
Simplify the Fraction: The fraction 9/12 can be simplified by dividing both the numerator and the denominator by their greatest common divisor, which is 3. This simplifies the fraction to 3/4.
-
Convert to a Decimal: To convert the fraction 3/4 to a decimal, divide the numerator (3) by the denominator (4): 3 ÷ 4 = 0.75
-
Convert to a Percentage: Multiply the decimal by 100 to express it as a percentage: 0.75 x 100 = 75%
Therefore, 9 out of 12 is equal to 75%.
Method 2: Using the Proportion Method
This method involves setting up a proportion to solve for the percentage.
-
Set up the Proportion: We set up a proportion where x represents the percentage we want to find:
9/12 = x/100
-
Cross-Multiply: Cross-multiply the terms:
12x = 900
-
Solve for x: Divide both sides by 12:
x = 900/12 = 75
Therefore, x = 75%, confirming that 9 out of 12 is equal to 75%.
Method 3: Using a Calculator
Modern calculators make percentage calculations incredibly simple. Most calculators have a percentage function. Simply enter 9 ÷ 12 and then multiply by 100 to obtain the percentage. The result, as before, will be 75%.
Practical Applications of Calculating Percentages
The ability to calculate percentages like "9 out of 12" has a wide range of applications across various fields:
1. Academic Performance:
Imagine a student scoring 9 out of 12 on a quiz. Knowing that this equates to 75% provides a clear understanding of their performance relative to the total possible score. This allows for effective tracking of progress and identification of areas needing improvement.
2. Business and Finance:
In business, calculating percentages is vital. For instance, if a company aims to increase sales by 9 out of 12 units, understanding that this represents a 75% increase helps in setting realistic targets and evaluating performance. Similarly, calculating profit margins, discounts, and interest rates all rely on percentage calculations.
3. Everyday Life:
Percentages permeate everyday life. Discount percentages in stores, tax calculations, tip calculations at restaurants, and even understanding weather forecasts (e.g., a 75% chance of rain) all involve working with percentages.
4. Data Analysis and Statistics:
In data analysis and statistical studies, percentages are used to represent proportions within datasets. Understanding the percentage of a specific outcome within a larger sample is crucial for drawing meaningful conclusions and making informed decisions.
Expanding on the Concept: Variations and Further Exploration
While we've focused on "9 out of 12," the principles can be applied to any fraction or ratio. Let's explore some variations and related concepts:
1. Calculating Percentages from Different Fractions:
The methods discussed above—using fractions, proportions, or calculators—are universally applicable to other fractions. For example, let's consider 7 out of 15:
- Fraction: 7/15
- Decimal: 7 ÷ 15 ≈ 0.4667
- Percentage: 0.4667 x 100 ≈ 46.67%
Therefore, 7 out of 15 is approximately 46.67%.
2. Working with Decimals and Percentages:
Converting between decimals and percentages is straightforward. To convert a decimal to a percentage, multiply by 100. To convert a percentage to a decimal, divide by 100. For instance:
- 0.6 = 60%
- 85% = 0.85
3. Understanding Percentage Increase and Decrease:
Calculating percentage changes involves finding the difference between two values and expressing this difference as a percentage of the original value. For example, if a value increases from 10 to 19, the percentage increase is calculated as follows:
- Difference: 19 - 10 = 9
- Percentage Increase: (9/10) x 100 = 90%
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages is a valuable skill with far-reaching applications. This comprehensive guide has explored multiple methods for calculating 9 out of 12 as a percentage (75%), emphasizing the underlying principles and providing practical examples. Mastering these techniques will significantly enhance your ability to interpret data, make informed decisions, and navigate various aspects of life involving proportions and ratios. Remember that consistent practice and application are key to solidifying your understanding and improving your proficiency in working with percentages. From academic success to financial literacy and beyond, the ability to handle percentages with confidence will prove an invaluable asset.
Latest Posts
Latest Posts
-
A Warehouse Received 250 Orders In April
Jul 10, 2025
-
How Many Square Inches Are In 1 Square Foot
Jul 10, 2025
-
How Many Square Feet Is 3 4 Acre
Jul 10, 2025
-
How Many Centimeters Are In A Meter Stick
Jul 10, 2025
-
Why Did The Orchestra Get An R Rating
Jul 10, 2025
Related Post
Thank you for visiting our website which covers about 9 Out Of 12 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.