9 Out Of 24 As A Percentage
Kalali
Mar 31, 2025 · 5 min read
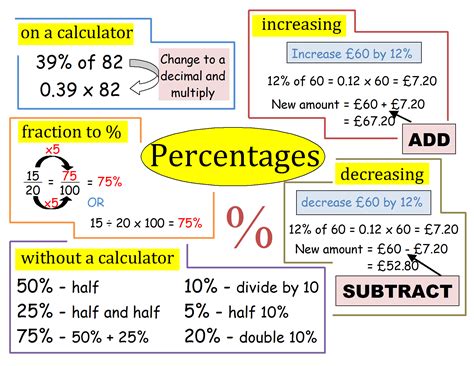
Table of Contents
9 out of 24 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields. Understanding how to calculate percentages is crucial for everyday tasks, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will delve into the calculation of "9 out of 24 as a percentage," providing a step-by-step explanation, exploring different methods, and showcasing practical examples. We'll also examine the broader context of percentage calculations and their importance in various scenarios.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Percentages are a convenient way to compare proportions and represent parts of a whole.
Calculating 9 out of 24 as a Percentage: Method 1 (Using Fractions)
The most straightforward method involves converting the given fraction into a percentage. The steps are:
1. Express the Information as a Fraction:
The phrase "9 out of 24" is easily represented as the fraction 9/24.
2. Simplify the Fraction (If Possible):
We can simplify this fraction by finding the greatest common divisor (GCD) of 9 and 24, which is 3. Dividing both the numerator and the denominator by 3, we get:
9/24 = 3/8
3. Convert the Fraction to a Decimal:
To convert the fraction 3/8 to a decimal, divide the numerator (3) by the denominator (8):
3 ÷ 8 = 0.375
4. Convert the Decimal to a Percentage:
Multiply the decimal by 100 to convert it to a percentage:
0.375 × 100 = 37.5%
Therefore, 9 out of 24 is equal to 37.5%.
Calculating 9 out of 24 as a Percentage: Method 2 (Using Proportions)
This method utilizes the concept of proportions to solve the problem. We set up a proportion where 'x' represents the percentage we want to find:
9/24 = x/100
To solve for 'x', we cross-multiply:
24x = 900
Now, divide both sides by 24:
x = 900/24 = 37.5
Therefore, x = 37.5%. This method confirms the result obtained using the fraction method.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in numerous real-world scenarios. Here are a few examples:
-
Sales and Discounts: Calculating discounts on products, understanding sales tax, and determining the final price after a discount are all based on percentage calculations. For instance, a 20% discount on a $100 item means a reduction of $20, resulting in a final price of $80.
-
Finance and Investments: Calculating interest rates on loans and investments, determining returns on investments, and analyzing financial statements all involve working with percentages. Understanding compound interest, for example, requires a solid grasp of percentage calculations.
-
Statistics and Data Analysis: Percentages are frequently used to represent proportions in data sets. For example, in surveys and polls, results are often expressed as percentages to show the distribution of responses. Analyzing market share, conversion rates, and other key performance indicators (KPIs) also relies heavily on percentage calculations.
-
Everyday Life: Calculating tips in restaurants, determining the percentage of ingredients in recipes, and understanding nutritional information on food labels all involve working with percentages.
Beyond the Basics: More Complex Percentage Problems
While calculating "9 out of 24 as a percentage" is a relatively simple problem, many percentage calculations are more complex. These can involve:
-
Finding the Percentage Increase or Decrease: This involves determining the percentage change between two values. For instance, if a quantity increases from 50 to 60, the percentage increase is calculated as [(60-50)/50] * 100 = 20%.
-
Calculating Percentage Points: This is different from a percentage change. A change from 50% to 60% represents a 10-percentage point increase, not a 20% increase.
-
Working with Multiple Percentages: Some problems involve applying multiple percentages sequentially, such as calculating compound interest or applying successive discounts.
-
Reverse Percentage Problems: These involve finding the original value when a percentage and the final value are known. For example, if a discounted item costs $80 after a 20% discount, the original price can be calculated using reverse percentage techniques.
Mastering Percentage Calculations: Tips and Tricks
Here are some helpful tips to improve your understanding and proficiency in percentage calculations:
-
Practice Regularly: The key to mastering any mathematical concept is consistent practice. Work through various problems to build your confidence and understanding.
-
Utilize Online Calculators and Resources: While understanding the underlying principles is important, using online calculators can be helpful for checking your answers and exploring different approaches.
-
Break Down Complex Problems: For complex percentage problems, break them down into smaller, more manageable steps. This will make the problem easier to approach and solve.
-
Understand the Context: Pay close attention to the wording of the problem to understand exactly what is being asked. Misinterpreting the question can lead to incorrect answers.
-
Visual Aids: Using diagrams or visual representations can help to conceptualize percentage problems, especially those involving percentage increases or decreases.
Conclusion
Calculating "9 out of 24 as a percentage" is a fundamental exercise that highlights the importance of understanding fraction-to-percentage conversions. This skill is applicable in diverse fields, from finance and statistics to everyday life scenarios. By mastering different methods and understanding the underlying principles, you can confidently tackle various percentage problems and apply this essential skill in numerous practical contexts. Remember to practice regularly, utilize resources when needed, and break down complex problems into smaller steps to build your proficiency and confidence in percentage calculations. The ability to accurately and efficiently calculate percentages is a valuable asset in both academic and professional settings.
Latest Posts
Latest Posts
-
How Many Miles An Hour Is The Speed Of Sound
Apr 02, 2025
-
What Is The Percent Of 20 Out Of 25
Apr 02, 2025
-
Where Is The North Magnetic Pole Of This Current Loop
Apr 02, 2025
-
Magnesium Metal Or Nonmetal Or Metalloid
Apr 02, 2025
-
How Many Ounces Are In 125 Ml
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 9 Out Of 24 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
