Common Multiples Of 16 And 12
Kalali
Mar 24, 2025 · 5 min read
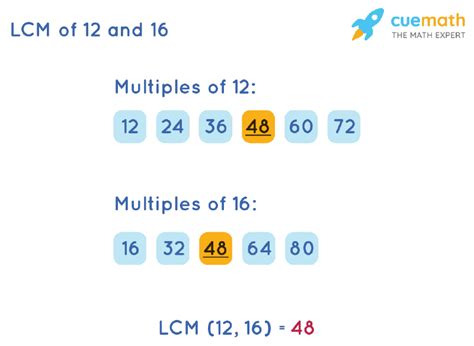
Table of Contents
- Common Multiples Of 16 And 12
- Table of Contents
- Unveiling the Secrets of Common Multiples: A Deep Dive into the Multiples of 16 and 12
- Understanding Multiples and Common Multiples
- Finding Common Multiples of 16 and 12: Method 1 - Listing Multiples
- Finding Common Multiples of 16 and 12: Method 2 - Prime Factorization and LCM
- Understanding the Relationship Between LCM and Common Multiples
- Applications of Common Multiples
- Beyond the Basics: Exploring Further Concepts
- Conclusion: Mastering the Art of Common Multiples
- Latest Posts
- Latest Posts
- Related Post
Unveiling the Secrets of Common Multiples: A Deep Dive into the Multiples of 16 and 12
Finding common multiples might seem like a simple arithmetic task, but understanding the underlying principles and exploring different methods to identify them unlocks a deeper appreciation of number theory. This comprehensive guide delves into the fascinating world of common multiples, specifically focusing on the multiples of 16 and 12. We'll explore various techniques, from listing multiples to employing the least common multiple (LCM), and discuss practical applications of this concept.
Understanding Multiples and Common Multiples
Before we dive into the specifics of 16 and 12, let's establish a firm understanding of the fundamental concepts.
What is a Multiple?
A multiple of a number is the result of multiplying that number by any integer (whole number). For example, multiples of 4 are 4, 8, 12, 16, 20, and so on. Each of these numbers is a product of 4 and another integer.
What is a Common Multiple?
A common multiple is a number that is a multiple of two or more numbers. For instance, consider the numbers 6 and 8. Multiples of 6 include 6, 12, 18, 24, 30... Multiples of 8 include 8, 16, 24, 32, 40... Notice that 24 appears in both lists. Therefore, 24 is a common multiple of 6 and 8.
Finding Common Multiples of 16 and 12: Method 1 - Listing Multiples
The most straightforward approach to finding common multiples is by listing the multiples of each number and identifying the common ones. Let's apply this method to 16 and 12:
Multiples of 16: 16, 32, 48, 64, 80, 96, 112, 128, 144, 160, 176, 192, 208, 224, 240...
Multiples of 12: 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, 132, 144, 156, 168, 180, 192, 204, 216, 228, 240...
By comparing the two lists, we can easily identify common multiples. Some of the common multiples of 16 and 12 are: 48, 96, 144, 192, 240... This method works well for smaller numbers but can become cumbersome with larger numbers.
Finding Common Multiples of 16 and 12: Method 2 - Prime Factorization and LCM
A more efficient method involves finding the least common multiple (LCM) using prime factorization. The LCM is the smallest positive number that is a multiple of all the numbers in a given set. Once we find the LCM, all other common multiples will be multiples of the LCM.
Prime Factorization:
- 16: 2 x 2 x 2 x 2 = 2<sup>4</sup>
- 12: 2 x 2 x 3 = 2<sup>2</sup> x 3
Finding the LCM:
To find the LCM, we take the highest power of each prime factor present in the factorizations:
- Highest power of 2: 2<sup>4</sup> = 16
- Highest power of 3: 3<sup>1</sup> = 3
Therefore, the LCM of 16 and 12 is 16 x 3 = 48.
This means that 48 is the smallest common multiple of 16 and 12. All other common multiples are multiples of 48: 48, 96, 144, 192, 240, and so on. This method is far more efficient, especially when dealing with larger numbers or multiple numbers.
Understanding the Relationship Between LCM and Common Multiples
The LCM plays a crucial role in determining all common multiples. Every common multiple of a set of numbers is a multiple of their LCM. This provides a concise and elegant way to generate all common multiples: simply multiply the LCM by successive integers.
In our case, the LCM of 16 and 12 is 48. Therefore, all common multiples are of the form 48n, where n is any positive integer. This gives us the sequence: 48, 96, 144, 192, 240, 288... and so on to infinity.
Applications of Common Multiples
The concept of common multiples finds practical applications in various fields:
-
Scheduling: Imagine two events happening on a repeating schedule. One event occurs every 12 days and another every 16 days. To determine when both events will occur on the same day, we need to find the common multiples of 12 and 16. The LCM (48) tells us that both events will coincide every 48 days.
-
Measurement and Conversions: When dealing with measurements that need to be expressed in different units, common multiples become important. For instance, converting between inches and centimeters involves working with common multiples of their respective units.
-
Modular Arithmetic: In cryptography and computer science, modular arithmetic relies heavily on the concept of multiples and common multiples to solve various problems.
-
Music Theory: The concept of common multiples plays a significant role in music theory, especially in determining harmonious intervals and chords.
-
Geometry and Tessellations: Common multiples are essential when designing repeating patterns and tessellations, ensuring patterns align seamlessly.
Beyond the Basics: Exploring Further Concepts
Having mastered the fundamentals of finding common multiples of 16 and 12, we can delve into more advanced aspects:
-
Greatest Common Divisor (GCD): The GCD is the largest number that divides evenly into both numbers. Understanding the relationship between the LCM and GCD can further streamline the process of finding common multiples.
-
Euclidean Algorithm: This efficient algorithm provides a method for finding the GCD of two numbers, which can then be used to calculate the LCM.
-
Working with More Than Two Numbers: The principles of finding common multiples extend seamlessly to cases involving more than two numbers. The prime factorization method provides a consistent way to find the LCM of any set of numbers.
-
Infinite Number of Common Multiples: It's important to note that every pair of numbers (except for 0) has an infinite number of common multiples. The LCM simply identifies the smallest positive common multiple.
Conclusion: Mastering the Art of Common Multiples
Understanding common multiples is not just about solving arithmetic problems; it's about grasping fundamental concepts in number theory with wide-ranging applications. While listing multiples can be a starting point, employing prime factorization and the LCM provides a much more efficient and powerful method, especially when dealing with larger numbers. By mastering these techniques, you'll not only improve your mathematical skills but also gain a deeper appreciation for the interconnectedness of seemingly simple arithmetic concepts. Remember, practice is key! Try working through different sets of numbers to solidify your understanding and unlock the fascinating world of common multiples.
Latest Posts
Latest Posts
-
Cuanto Es 37 Grados Fahrenheit En Centigrados
Mar 29, 2025
-
63 Is What Percent Of 84
Mar 29, 2025
-
Is Pure Water Homogeneous Or Heterogeneous
Mar 29, 2025
-
80 Out Of 90 As A Percentage
Mar 29, 2025
-
What Is The Continuous Flow Of Electric Charge
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Common Multiples Of 16 And 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
