Common Multiples Of 5 And 3
Kalali
Apr 04, 2025 · 5 min read
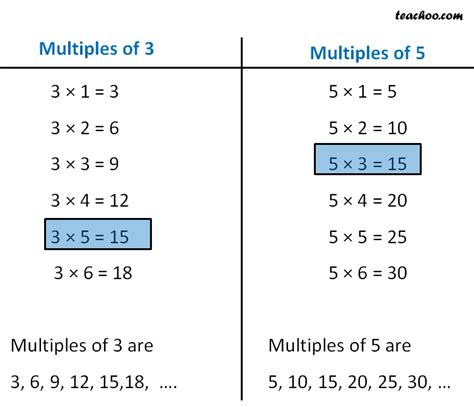
Table of Contents
Common Multiples of 5 and 3: A Deep Dive into Number Theory
Finding common multiples, especially those of seemingly simple numbers like 5 and 3, might seem straightforward at first glance. However, delving deeper reveals a rich tapestry of mathematical concepts, algorithms, and applications. This comprehensive guide explores the fascinating world of common multiples of 5 and 3, covering fundamental concepts, advanced techniques, and real-world examples. We’ll unravel the mystery behind these numbers and equip you with the knowledge to tackle similar problems with confidence.
Understanding Multiples and Common Multiples
Before we dive into the specifics of 5 and 3, let's establish a firm understanding of the core concepts.
What is a Multiple?
A multiple of a number is the product of that number and any integer (a whole number, including zero and negative numbers). For example:
- Multiples of 5: 0, 5, 10, 15, 20, 25, 30, 35, 40, ... (and so on to infinity in both positive and negative directions)
- Multiples of 3: 0, 3, 6, 9, 12, 15, 18, 21, 24, 30, ... (and so on to infinity)
Notice that the multiples extend infinitely in both positive and negative directions. We usually focus on the positive multiples in practical applications.
What is a Common Multiple?
A common multiple of two or more numbers is a number that is a multiple of all of those numbers. Looking at our examples above, we can see that 15 and 30 are common multiples of both 5 and 3.
Finding Common Multiples of 5 and 3
There are several ways to determine the common multiples of 5 and 3. Let's explore the most common methods:
Method 1: Listing Multiples
This is the most straightforward method, especially for smaller numbers. We simply list out the multiples of each number until we find common ones.
- Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60...
- Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48, 51, 54, 57, 60...
By comparing the lists, we easily identify the common multiples: 15, 30, 45, 60, and so on. This method works well for smaller numbers, but becomes cumbersome for larger ones.
Method 2: Using Prime Factorization
This method is more efficient for larger numbers and provides a deeper understanding of the underlying mathematical principles.
-
Prime Factorization: Find the prime factorization of each number.
- 5 = 5 (5 is a prime number)
- 3 = 3 (3 is a prime number)
-
Least Common Multiple (LCM): To find the least common multiple (the smallest positive common multiple), we take the highest power of each prime factor present in either factorization and multiply them together. In this case:
LCM(5, 3) = 5 × 3 = 15
-
Finding other common multiples: Once we have the LCM, we can find other common multiples by multiplying the LCM by any positive integer:
- 15 × 1 = 15
- 15 × 2 = 30
- 15 × 3 = 45
- 15 × 4 = 60
- and so on...
Method 3: Using the Formula (LCM and GCD)
This method utilizes the relationship between the Least Common Multiple (LCM) and the Greatest Common Divisor (GCD).
-
Greatest Common Divisor (GCD): The GCD is the largest number that divides both numbers without leaving a remainder. Since 5 and 3 are both prime and have no common factors other than 1, their GCD is 1. (GCD(5,3) = 1)
-
Formula: The relationship between LCM and GCD is given by the formula:
LCM(a, b) × GCD(a, b) = a × b
Where 'a' and 'b' are the two numbers.
-
Calculating LCM: Substituting the values for 5 and 3:
LCM(5, 3) × 1 = 5 × 3 LCM(5, 3) = 15
-
Other common multiples: As before, we multiply the LCM (15) by any positive integer to find other common multiples.
Applications of Common Multiples
Understanding common multiples extends far beyond simple number theory. They have practical applications in various fields:
Scheduling and Time Management
Imagine two buses that leave a station at different intervals. One bus leaves every 5 minutes, and the other every 3 minutes. Determining when both buses leave at the same time requires finding the common multiples of 5 and 3. The first time they leave together is after 15 minutes.
Pattern Recognition and Geometry
Common multiples often appear in repeating patterns and geometric designs. For instance, tiling a floor with tiles of two different sizes often requires understanding their common multiples to ensure seamless fitting.
Modular Arithmetic and Cryptography
The concept of common multiples plays a crucial role in modular arithmetic, which forms the basis of many modern cryptographic systems. These systems rely on the properties of common multiples to ensure the security of sensitive data.
Beyond 5 and 3: Generalizing the Concepts
The principles discussed above can be applied to find common multiples of any two or more numbers. The prime factorization method and the LCM/GCD formula are particularly useful for larger and more complex numbers.
Conclusion: Mastering Common Multiples
Finding common multiples, particularly those of 5 and 3, is a fundamental concept in number theory with surprisingly wide-ranging applications. By understanding the different methods for finding common multiples—listing multiples, prime factorization, and using the LCM/GCD relationship—we gain a powerful tool for solving various mathematical and real-world problems. This knowledge equips you to approach more complex scenarios involving common multiples and further explore the fascinating world of mathematics. Remember, the key lies in understanding the underlying principles, not just memorizing formulas. Practice makes perfect, so keep exploring and experimenting with different numbers to solidify your understanding.
Latest Posts
Latest Posts
-
What Does A Turtle Look Like Without Its Shell
Apr 05, 2025
-
For Which Elements Is The Stock System Primarily Used
Apr 05, 2025
-
A Surgical Procedure To Remove Necrosed Bone Is Called
Apr 05, 2025
-
180 Degrees Celsius Is How Many Degrees Fahrenheit
Apr 05, 2025
-
Diagonals Of Rhombus Bisect Vertex Angles
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Common Multiples Of 5 And 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
