How Are The Wavelength Frequency And Wave Speed Related
Kalali
Apr 01, 2025 · 5 min read
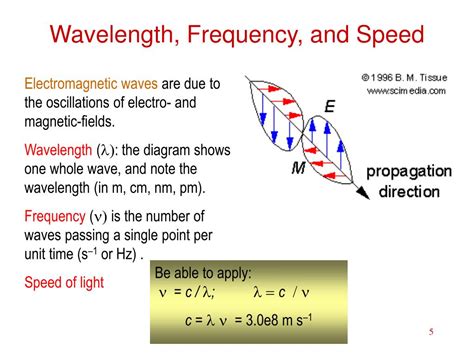
Table of Contents
How Are Wavelength, Frequency, and Wave Speed Related?
Understanding the relationship between wavelength, frequency, and wave speed is fundamental to comprehending various phenomena in physics, from the behavior of light and sound to the intricacies of quantum mechanics. This comprehensive guide will explore this crucial relationship, explaining the concepts individually before delving into their interconnectedness through a simple yet powerful formula. We will also examine real-world applications and implications of this relationship.
What is Wavelength?
Wavelength (λ, pronounced "lambda") is the spatial period of a wave—the distance over which the wave's shape repeats. Imagine a wave in the ocean; the wavelength is the distance between two successive crests (or troughs). It's measured in units of length, typically meters (m), centimeters (cm), or nanometers (nm), depending on the type of wave.
Key characteristics of wavelength:
- Directly related to the wave's energy: Shorter wavelengths generally correspond to higher energy waves. For example, ultraviolet light has a shorter wavelength than infrared light and is therefore more energetic.
- Dependent on the medium: The wavelength of a wave can change as it travels from one medium to another. This is because the speed of the wave changes, and as we'll see, wavelength and speed are directly related.
- Used to categorize electromagnetic waves: The electromagnetic spectrum is organized based on wavelength, ranging from long radio waves to short gamma rays.
What is Frequency?
Frequency (f) represents the number of complete wave cycles that pass a given point per unit of time. It's essentially how often the wave oscillates. The standard unit for frequency is Hertz (Hz), where 1 Hz equals one cycle per second.
Key characteristics of frequency:
- Inversely related to the wave's period: The period (T) is the time it takes for one complete wave cycle to pass a point. The relationship is: f = 1/T.
- Related to energy: Higher frequency waves carry more energy. This is why high-frequency electromagnetic radiation like X-rays is more damaging than low-frequency radio waves.
- Determines the pitch of sound: In the context of sound waves, frequency determines the perceived pitch. Higher frequency sounds are perceived as higher pitched.
What is Wave Speed?
Wave speed (v) describes how fast the wave propagates through a medium. It represents the distance the wave travels in a given amount of time. The unit for wave speed is typically meters per second (m/s).
Key characteristics of wave speed:
- Dependent on the medium: The speed of a wave varies depending on the properties of the medium it's traveling through. For example, sound travels faster in solids than in gases. Light travels fastest in a vacuum.
- Constant in a uniform medium: In a uniform medium (a medium with consistent properties), the wave speed remains constant.
- Independent of frequency (in some cases): For some types of waves, like light in a vacuum, the wave speed is independent of its frequency. However, for other waves (e.g., sound waves in air), the speed can be affected by frequency, a phenomenon known as dispersion.
The Fundamental Relationship: The Wave Equation
The relationship between wavelength (λ), frequency (f), and wave speed (v) is elegantly expressed by the wave equation:
v = fλ
This equation signifies that the wave speed is the product of its frequency and wavelength. Let's examine what this implies:
- Direct proportionality between speed and wavelength: If the frequency remains constant, an increase in wavelength leads to an increase in wave speed, and vice-versa.
- Direct proportionality between speed and frequency: If the wavelength remains constant, an increase in frequency leads to an increase in wave speed, and vice-versa.
- Inverse proportionality between wavelength and frequency: If the wave speed remains constant, an increase in frequency leads to a decrease in wavelength, and vice-versa.
Real-World Applications and Implications
The wave equation has wide-ranging applications in various fields:
1. Acoustics: Understanding the relationship between frequency, wavelength, and speed is crucial in acoustics. It helps in designing concert halls, soundproofing materials, and musical instruments. The specific frequencies and wavelengths of sound waves determine the quality and pitch of the sound we hear.
2. Electromagnetism: In the study of electromagnetic waves (light, radio waves, X-rays, etc.), this relationship is essential. Different wavelengths of electromagnetic radiation have different properties and applications. For instance, radio waves with longer wavelengths are used for broadcasting, while X-rays with shorter wavelengths are used in medical imaging. The speed of light in a vacuum is a fundamental constant in physics.
3. Oceanography: The study of ocean waves relies heavily on understanding the relationship between wavelength, frequency, and wave speed. This knowledge is crucial for predicting wave heights and patterns, essential for coastal engineering and maritime safety.
4. Seismology: Seismic waves, generated by earthquakes, are characterized by their wavelength, frequency, and speed. The relationship between these parameters helps seismologists to understand the propagation of seismic waves through the Earth's interior and to locate the epicenter of earthquakes.
Beyond the Basics: Dispersion and Wave Interference
While the wave equation (v = fλ) is fundamental, it's important to note that certain phenomena can complicate this simple relationship:
1. Dispersion: Dispersion occurs when the wave speed depends on the frequency (or wavelength) of the wave. This is observed in various media, such as optical fibers or water. Different frequencies of light will travel at slightly different speeds, leading to phenomena like rainbow formation and chromatic aberration in lenses.
2. Wave Interference: When two or more waves interact, they can interfere with each other, leading to constructive interference (waves add up, resulting in a larger amplitude) or destructive interference (waves cancel each other out, resulting in a smaller amplitude). These interference patterns are influenced by the wavelengths and frequencies of the interacting waves.
Conclusion
The relationship between wavelength, frequency, and wave speed, as encapsulated in the wave equation (v = fλ), is a cornerstone of wave physics. Understanding this relationship provides the key to comprehending a wide spectrum of physical phenomena, from the propagation of sound to the behavior of light. Although dispersion and wave interference introduce complexities, the fundamental equation remains a vital tool for analyzing wave behavior in various settings and applications. Mastering this concept unlocks a deeper appreciation of the intricate world of waves and their pervasive influence on our physical reality.
Latest Posts
Latest Posts
-
1 8 Of An Ounce In Grams
Apr 02, 2025
-
How Many Feet Are In 150 Inches
Apr 02, 2025
-
What Is 30 X 40 Cm In Inches
Apr 02, 2025
-
Is Toasting Bread A Physical Or Chemical Change
Apr 02, 2025
-
Dos Pares De Angulos Alternos Internos Ejemplos
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How Are The Wavelength Frequency And Wave Speed Related . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
