How To Find Cross Sectional Area
Kalali
Mar 29, 2025 · 5 min read
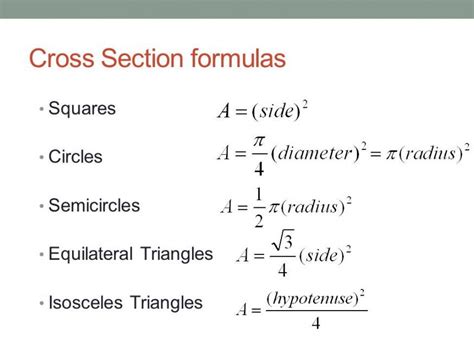
Table of Contents
How to Find Cross-Sectional Area: A Comprehensive Guide
Finding the cross-sectional area of an object is a fundamental concept in various fields, including engineering, physics, and mathematics. Understanding how to calculate this area is crucial for solving problems related to stress, strain, fluid flow, and more. This comprehensive guide will explore different methods for determining cross-sectional area, catering to various shapes and complexities. We'll delve into both simple and advanced techniques, providing you with the tools to tackle any challenge.
Understanding Cross-Sectional Area
Before diving into the calculations, let's define what cross-sectional area truly means. The cross-sectional area is the area of a two-dimensional shape that is obtained when a three-dimensional object is sliced perpendicular to its longitudinal axis. Imagine slicing a loaf of bread; each slice represents a cross-section, and its area is the cross-sectional area. The shape of this cross-section varies depending on the object's geometry.
The importance of understanding cross-sectional area stems from its application in numerous real-world scenarios. In structural engineering, it's vital for calculating the stress and strain on materials under load. In fluid mechanics, it plays a crucial role in determining fluid flow rates through pipes or channels. Even in simple scenarios like calculating the volume of a cylinder, the cross-sectional area is a fundamental building block.
Calculating Cross-Sectional Area for Common Shapes
Let's begin with the simplest shapes and gradually move towards more complex geometries.
1. Circle
The most straightforward case is a circular cross-section. The formula is:
Area = πr²
where:
- π (pi) is approximately 3.14159
- r is the radius of the circle (half of the diameter)
Example: A pipe has a diameter of 10 cm. Its radius is 5 cm. Therefore, its cross-sectional area is π * (5 cm)² ≈ 78.54 cm².
2. Square and Rectangle
For squares and rectangles, the calculation is equally simple:
Area = length × width
For a square, length and width are equal.
Example: A square beam with sides of 4 inches has a cross-sectional area of 4 inches × 4 inches = 16 square inches. A rectangular beam with length 6 cm and width 3 cm has a cross-sectional area of 6 cm × 3 cm = 18 cm².
3. Triangle
Finding the area of a triangular cross-section requires a slightly more complex formula:
Area = (1/2) × base × height
where:
- base is the length of the triangle's base
- height is the perpendicular distance from the base to the opposite vertex
Example: A triangular metal plate has a base of 8 cm and a height of 5 cm. Its cross-sectional area is (1/2) × 8 cm × 5 cm = 20 cm².
4. Trapezoid
A trapezoidal cross-section, often encountered in structural elements, requires the following formula:
Area = (1/2) × (base1 + base2) × height
where:
- base1 and base2 are the lengths of the two parallel sides
- height is the perpendicular distance between the two parallel sides
Example: A trapezoidal channel has parallel sides of 10 meters and 6 meters, with a height of 4 meters. Its cross-sectional area is (1/2) × (10 m + 6 m) × 4 m = 32 m².
Calculating Cross-Sectional Area for Irregular Shapes
Dealing with irregular shapes requires more advanced techniques. Here are a few approaches:
1. Dividing into Smaller Shapes
A complex shape can often be broken down into smaller, simpler shapes (rectangles, triangles, etc.). Calculate the area of each smaller shape and sum them up to find the total cross-sectional area. This method is particularly useful when dealing with shapes that resemble composite figures.
2. Using Grid Method (Planimeter Method)
For highly irregular shapes, the grid method provides a reasonable approximation. Overlay a grid of squares over the cross-section. Count the number of squares completely inside the shape, and estimate the area of partially covered squares. Multiply the total count by the area of a single square to obtain an approximate cross-sectional area.
3. Using a Planimeter
A planimeter is a specialized instrument designed for measuring the area of any two-dimensional shape, regardless of its complexity. It's a precise mechanical device that traces the outline of the shape, automatically calculating the enclosed area. This method is often used for intricate shapes that are difficult to approximate using other techniques.
4. Numerical Integration
For precise measurement of complex cross-sectional areas, numerical integration techniques, such as the trapezoidal rule or Simpson's rule, can be applied. These techniques involve dividing the area into numerous small segments and approximating the area of each segment using simple shapes. This method provides a highly accurate estimate, especially when combined with computational software or programming.
Advanced Techniques and Applications
The techniques described above form the foundation for calculating cross-sectional area. However, more advanced scenarios often require further considerations:
-
Composite Shapes: Many engineering components consist of multiple shapes combined. To find the cross-sectional area, calculate the area of each individual shape and then sum them up. This is crucial for beams with holes, channels with flanges, or other similar structures.
-
Curved Shapes: For shapes with curved boundaries, numerical integration or approximation methods are often necessary. Software like CAD programs can automate these calculations.
-
Three-Dimensional Objects with Variable Cross-Sections: For objects with cross-sections that vary along their length (e.g., a tapered beam), you need to consider the variation and potentially use calculus to find the total volume. This involves integrating the cross-sectional area along the object's length.
-
Applications in Fluid Mechanics: In fluid dynamics, the cross-sectional area is critical for calculating flow rates using the continuity equation. Understanding how cross-sectional area changes along a pipe or channel is crucial for optimizing flow.
Conclusion
Determining the cross-sectional area is a crucial skill across numerous disciplines. While simple shapes have straightforward formulas, complex geometries often demand more advanced techniques like numerical integration or the use of specialized instruments. Mastering these methods empowers you to solve problems involving stress, strain, fluid flow, and much more. Remember to always carefully consider the shape and select the appropriate calculation method for accurate and reliable results. The ability to accurately calculate cross-sectional areas is an essential skill for anyone working in fields where structural integrity and fluid dynamics are paramount. This comprehensive guide has provided you with the tools and knowledge to approach these calculations with confidence.
Latest Posts
Latest Posts
-
Is Snow A Solid Liquid Or Gas
Mar 31, 2025
-
Convert 1 1 2 Ounces To Cups
Mar 31, 2025
-
Explain The Difference Between How Autotrophs And Heterotrophs Acquire Energy
Mar 31, 2025
-
How Do You Find The Volume Of A Solid Figure
Mar 31, 2025
-
What Is 20 Percent Of 6
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How To Find Cross Sectional Area . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
